The exciting discovery of 7 earth-like planets 40 light years away raises the following question: If an exploration mission is scheduled to one or more of these planets in 2017 to find a possible home for the future, how long would it take for spaceships using current technology to travel there?
I'm looking for realistic answers, no matter how uninteresting, rather than hypothetical technologies such as a warp drive.
Although this is a simple $t=d/v$ calculation, where $v$ is the heliocentric velocity, $d$ the heliocentric distance and $t$ the travel time, one must use the velocity appropriate for current technology, which is Solar system escape through chemical rockets together with gravity-assisting planetary flybys. One must effectively use the velocity that the spacecraft will have at infinite distance from the Sun, when the conversion of its kinetic energy to gravitational potential energy is complete.
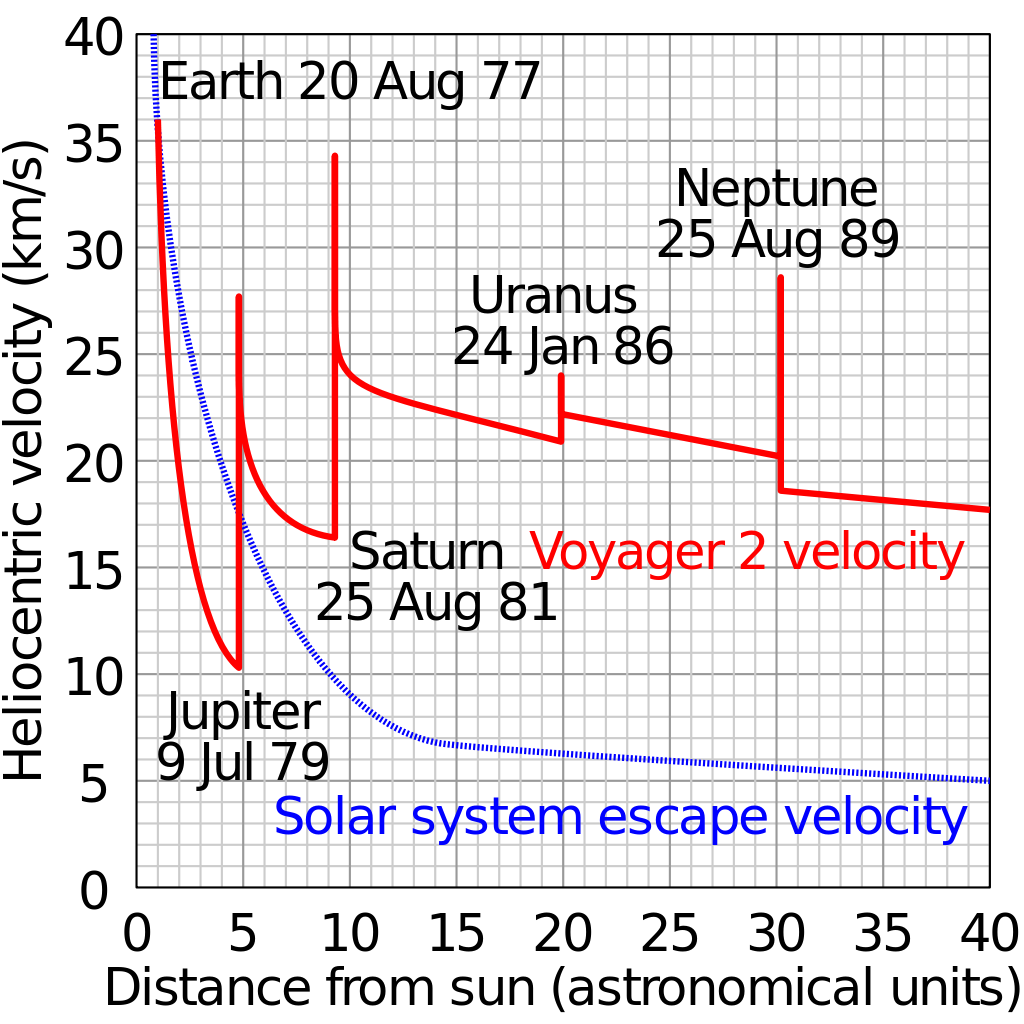
The above graph shows Voyager 2's heliocentric velocity (red) alongside the computed Sun-system escape velocity (blue) calculated from $\frac{1}{2}\,v_e^2 = \frac{G\,M_\odot}{r}$. One can see that, at 40 astronomical units, after all the flybys are done, and therefore after Voyager 2 has gotten all the kinetic energy it can from the assisting planets, the helocentric velocity is about $17.5{\rm km\,s^{-1}}$ whereas the escape velocity (essentially the gravitational potential deficit expressed as a kinetic energy) is $5{\rm km\,s^{-1}}$, thus the fraction of Voyager's kinetic energy leftover after achieving infinite separation from the Sun is $\frac{17.5^2 - 5^2}{17.5^2}$ and so the spaceship's velocity in this state will be:
$$\sqrt{\frac{17.5^2 - 5^2}{17.5^2}} \times 17.5=\sqrt{17.5^2 - 5^2}\approx 16.8{\rm km\,s^{-1}}$$
whence the travel time for 40 light years will be $40\times \frac{300\,000}{16.8}\approx 700\,000\,{\rm years}$.
I found this article: http://www.livescience.com/32655-whats-the-fastest-spacecraft-ever.html - The fastest object we are planning to build (Solar Probe Plus) will reach 724,000 km/h - But that is just from Earth to the Sun. Considering that speed, It would take aprox.: 59,627 years with today technology (https://www.google.com/#q=40+light+years+%2F+724000+km%2Fh&*).
I read an article stating a spacecraft traveling 38000 miles an hour would take approximately 80,000 years to travel 1 light year. If this is accurate, it will take 3,200,000 years to reach one of those planets.
The distance from here to the moon is approx 238,000 miles and we get there in about 3 days.
1 trillion is 1,000,000,000,000,000,000
1,000,000,000,000,000,000 Divided by 238,000 is 420.168.
420.168 times 3 days is 1,260.504 days to travel 1 light year.
1,260.504 times 40 light years is 50,420.168 days
50,420.168 divided by 365 days in a year is 138.137 years to reach them.
