Consider a pipe with length $L$ and uniform radius $A$ is held vertically. According to the continuity equation, the velocity of water going into the pipe seems to be the same as the velocity of water coming out. But according to Bernoulli's equation: $$P_{atm}+\frac{1}{2}\rho v_1^2+\rho gL=P_{atm}+\frac{1}{2}\rho v_2^2$$ $$v_2=\sqrt{v_1^2+2gL}$$ Which means that the water would come out faster, which doesn't make much sense. What is wrong with my equations?
$\begingroup$
$\endgroup$
asked Jan 20, 2017 at 15:37
Add a comment
|
6 Answers
$\begingroup$
$\endgroup$
14
You cannot have the same pressure at both ends of the pipe. The two velocities must be the same (assuming constant pipe diameter) due to mass conservation, so you'll have just the hydrostatic equation, $$p_2-p_1=\rho\,g\,L.$$ Note that your use of the Bernoulli equation for this problem implies that the flow is assumed to be ideal, with no friction. Also notice that the velocity in this problem remains indeterminate.
-
$\begingroup$ It could be fed at atmospheric pressure and be open to air at the bottom. My answer addresses how that works with continuity. This answer does explain the potential other interpretitation. $\endgroup$– JMacCommented Jan 20, 2017 at 16:45
-
$\begingroup$ @JMac No, it could not. Continuity requires that the velocities be the same everywhere in the pipe if the pipe has constant diameter as assumed in the OP. The Bernoulli equation then requires there to be a pressure increase top to bottom. $\endgroup$– PirxCommented Jan 20, 2017 at 16:49
-
$\begingroup$ The pipe has constant diameter; but there is nowhere that says the fluid must fill the pipe the entire time. Like I said, it's a different interpretation of the question. They don't specify if both ends are open or part of a closed in system; so we can't say for sure that it wont be atmospheric pressure on both ends. Realistically if there weren't a high enough pressure difference to account for the gravitational change the stream could thin itself down as it gains speed through the pipe. $\endgroup$– JMacCommented Jan 20, 2017 at 16:56
-
$\begingroup$ @JMac No, this won't happen. Feel free to perform an experiment with a straw... $\endgroup$– PirxCommented Jan 20, 2017 at 16:59
-
$\begingroup$ can you explain what would stop the water from thinning out as it gained velocity if both ends were open to atmosphere? $\endgroup$– JMacCommented Jan 20, 2017 at 17:03
$\begingroup$
$\endgroup$
2
The diameter of the pipe is likely included to mislead you.
Obviously the fluid should gain speed, but what's also obvious is that it can't increase in mass, and it shouldn't expand since water is basically incompressible.
I had to picture a stream of water allowed to pour freely. What will happen is that the stream diameter will become smaller to account for the faster velocity. If the water were a bar of elastic material this would be the same as stretching it. It's mass flow is the same, it just elongates as it becomes faster. By the time it reaches the bottom of the vertical pipe the stream will not have a diameter of A.
-
$\begingroup$ +1 Your proposed solution is a possible one. In fact I have witnessed it in a vertical drainage pipe that lets out rain water from the roof, nearby my laboratory. $\endgroup$– DeepCommented Jan 21, 2017 at 12:15
-
$\begingroup$ Basically, the water in the pipe goes into free fall. It is accelerating. To maintain continuity, the cross-section of flow will decrease. $\endgroup$ Commented Nov 11, 2019 at 20:29
$\begingroup$
$\endgroup$
Your version of Bernoulli's equation only applies to steady flow -ie. to motion in which the velocity at any point is indepenedent of time. In the case of your pipe open at both ends the fluid will accelerate. In that case you must use the time-dependent version of Bernoulli. For the irrotational flow of in incompressible fluid (so that we can write ${\bf v}=\nabla \phi$) Bernoulli says that $$ \frac 12 |{\bf v}|^2 + \frac{P}{\rho} +\frac{\partial \phi}{\partial t}= const. $$ You are omitting the $\partial \phi/\partial t$.
answered Nov 11, 2019 at 19:46
$\begingroup$
$\endgroup$
3
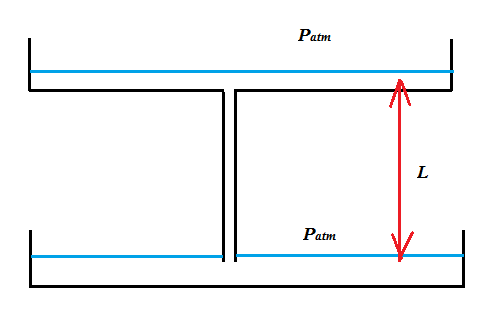
Imagine two very large (the liquid levels don't vary), very shallow (hydrostatic pressure is negligible at entrance and outlet) reservoirs, connected by a vertical pipe:
It's very tempting to apply Bernoulli here but as the OP observed that would violate the continuity equation.
In reality:
$$P_{atm}+\frac{1}{2}\rho v_1^2+\rho gL=P_{atm}+\frac{1}{2}\rho v_2^2,$$
is not applicable here because it neglects the resistance to flow (viscous drag) exerted by the pipe. In reality $v_1=v_2$ and we need to introduce a head loss term $\Delta P_{\mathrm{friction}}$:
$$P_{atm}+\frac{1}{2}\rho v_1^2+\rho gL=P_{atm}+\frac{1}{2}\rho v_1^2 +\Delta P_{\mathrm{friction}}$$
Or:
$$\rho gL=\Delta P_{\mathrm{friction}}$$
In the case of laminar flow through the pipe the Darcy-Weisbach equation can be used to calculate $\Delta P_{\mathrm{friction}}$:
$$\Delta P_{\mathrm{friction}}=f_{D}\frac{\rho}{2}\frac{v_1^2}{D}L$$
So that $v_1$ is not indeterminate.
For turbulent flow the calculation of $\Delta P_{\mathrm{friction}}$ is more complex.
-
$\begingroup$ it should be possible to satisfy energy equation and continuity without friction $\endgroup$ Commented Jan 20, 2017 at 18:32
-
$\begingroup$ This is not correct: It does not matter how shallow your top reservoir is. Within the confines of the simplifying assumptions made here, the pressure at the pipe entrance cannot be equal to $p_{atm}$. If you apply the Bernoulli equation to a streamline somewhere on the surface of the reservoir where we can assume the velocity to vanish, we can immediately see that the pressure at the pipe entrance will have dropped by $\frac{1}{2}\rho v^2$. @John Steed is right, of course. $\endgroup$– PirxCommented Jan 20, 2017 at 18:33
-
$\begingroup$ I note that the situation described by @Gert is a standard undergraduate fluid mechanics problem. The solution (for inifinitely shallow top reservoir and no friction) is simply a flow velocity of $v=\sqrt{2\rho\,g\,L}$, and the pressure at the pipe entrance is $p_e=p_{atm}-\rho\,g\,L$. If $p_e$ drops too low, interesting things (such as cavitation) will happen. $\endgroup$– PirxCommented Jan 20, 2017 at 18:43
$\begingroup$
$\endgroup$
Following the schematics offered by Gert, you have to consider two stages of water flow:
1 - First stage while water flow velocity goes from zero to a certain max that with acceptable negligencies is given by gravity laws. Max velocity magnitude is function of the pipe length...
2 Second stage stable velocity stage if the water level of the feeding and hosting reservoirs remains constant or such fluctuations should be considered. Bernoulli equation applies considering also head losses from first entrance and length losses.
$\begingroup$
$\endgroup$
Consider a vertical pipe connected to a shallow reservoir:
There are mainly 3 points of interest:
The outlet of the vertical pipe:
The heads are: $P_{atm}+\frac{1}{2}ρv^2$
The inlet of the vertical pipe:
By continuity, the stream has same velocity at inlet and outlet
$P_{in}+\frac{1}{2}ρv^2 + ρgL = P_{atm}+\frac{1}{2}ρv^2$
$P_{in} = P_{atm} - ρgL$
At the surface of the reservoir:
$P_{atm} + ρgL = P_{in} + \frac{1}{2}ρv^2 + ρgL $
$v = \sqrt{2gL}$
which is the text book answer of Torricelli's law https://en.wikipedia.org/wiki/Torricelli%27s_law
The principle behind Bernoulli's equation is conservation of energy. The problem can be understood this way:
On water surface of the reservoir, the velocity is zero, but when water is entering the pipe, it gains velocity. Since there is no change in elevation in a shallow reservoir, energy must be converted from pressure head to kinetic head.
As water travels along the pipe, the elevation head decreases but the kinetic head has to remain unchanged due to continuity, so energy is converted from elevation head to pressure head.
