Relative clock speeds of two satellites with same but opposite direction orbits

Question

Naïve reasoning: consider two satellites A and B that are in almost identical but opposite direction orbits, just not colliding. When A meets B, B is going past at a good speed, hence its clock is running slow relative to A. Half an orbit later they meet again, and again B is going past at a good speed, its clock running slow relative to A. But since the situation is symmetrical no clock difference can have accumulated. B’s clock is running slow relative to A in at least two points in the orbit, but it doesn’t accumulate a clock difference relative to A.

How is this explained?

---

Addendum:

Some answers have already been posted, and it seems they all can benefit from a common description of how clock ticks are communicated from satellite B to satellite A.

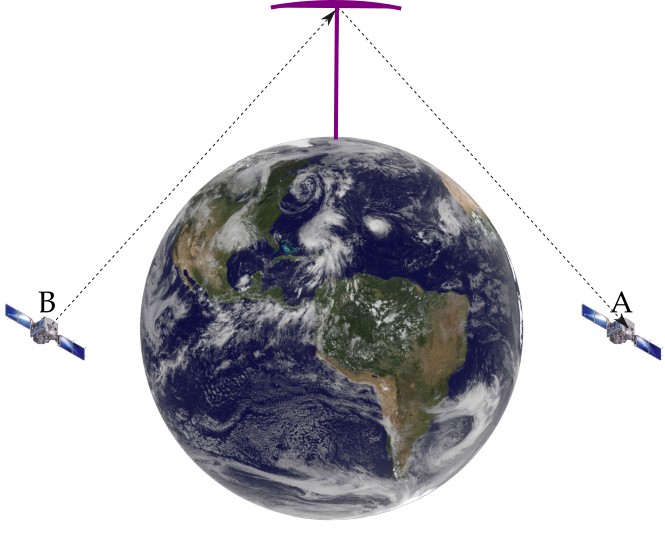
To avoid doppler effects and all that stuff, the satellites are assumed to have circular orbits outside the equator, and they communicate optically via a huge relay mirror placed on a some thousand km high pole, at the geographic north pole, like this signalling from a point X on B, to A:

The nice thing about this scheme is that the distance from B to A along the signal path is constant, so there's a constant communications delay: simple!

In order to make sure that special relativity can be considered as a valid approximation for when the satellites pass by each other (this has to do with clock skew in the reference frames), B's clock ticking is communicated not only via the above constant length path, but also directly from the single point X on B's side to closest receiving point on A. A's side is chock full of really tiny densely packed receiving points. As measured on board A, after a receiving point receives a B clock tick from the effectively coinciding point X on B, there is a fixed time delay until that same clock tick is also received via the North Pole Mirror signal path.

The question can be reformulated in terms of the clock ticks that A receives from B: one line of reasoning (e.g. considering receipt at the mirror) dictates constant spacing, while another line of reasoning, using special relativity as a valid approximation when A and B meet, says that at those occasions A will see longer intervals between the received ticks.

Answer 1

Special relativity applies to objects which are at rest in an inertial reference frame — that is, not accelerating. Objects which are moving relative to each other at constant velocity may have exactly one closest approach; afterwards they are moving away from each other for ever.

When your two satellites pass each other, there'll be a period of time when you may neglect the curvature of their orbits and analyze their clocks using special relativity. However that period of time does not extend for a half-orbit until their next interaction: if you neglected the satellites' accelerations, they would never meet again. So the symmetry must be restored if you treat the problem using general relativity.

Answer 2

This is a variation on the well-known (apparent) twin paradox. The general description of these kind of problems is a s follows: You have worldlines $C_1$ and $C_2$ which meet at events A and B. Both travelers reset their clocks at A and you want to know which clock is running late (or fast) at B, where they meet again. In order to solve such a problem you "simply" calculate the proper time of each traveler along her own worldline and compare them.

$$\Delta\tau = \int_{C_1} \, d\tau- \int_{C_2} \, d\tau$$

In general these line integrals are calculated using the metric tensor (thus implicitly taking into account spacetime curvature, if present)

$$\int_C \, d\tau = \int_C \sqrt{-g_{\mu\nu} \; dx^\mu \; dx^\nu}$$

where the metric has signature $(-+++)$.

In your case - ignoring the spinning of the planet - the situation is symmetric, so the proper time along $C_1$ and $C_2$ is the same.

If the planet is spinnng, then the proper time on the prograde and retrograde orbit is in general different.

Answer 3

This is a perfect example of how you can confuse yourself unnecessarily by not following the correct procedure! Time dilations in the Special Theory of Relativity (SR) and in the General Theory of Relativity (GR) are always with respect to an inertial system clock. Both the satellites are non-inertial accelerating systems, changing each instant. You cannot compare clocks in the two non-inertial systems by using relative velocities in the SR equations. So both the satellite clocks will run slow with respect to an inertial system clock and once synchronized will show the same time forever. The time dilation equation in SR depends on v^2 and in GR depends on just location in the gravitational field.

Answer 4

At any given instant, $A$'s clock is running slow in $B$'s (instantaneous inertial) frame.

At any given instant, $B$'s frame is not the same as it was an instant ago, and therefore $B$ changes his mind from one instant to the next both about how long it's been since the clocks were synchronized and about how fast $B's$ own clock (as well as $A$'s own clock) was running at various times in the past.

By the time $A$ and $B$ come back together, $B$ says:

There's $A$. His clock is running slow at the moment. It has also run slow by various factors at various times since we last met. My own clock has also run slow by various factors at various times since we last met. As a result, both of our clocks are ``incorrect'' in the sense that the time that (according to my current frame) has passed since we synchronized to zero is different from the time currently showing on both of our clocks.

You can, of course, quantify this, reconstructing (from $B$'s point of view at the instant of the reunion) exactly how slow each clock was running at each point in the past, and verifying that the total slowdown on one clock is equal to the total slowdown on the other. But of course, you already know by simple symmetry considerations how this is going to turn out.

Edited to add: (No new ideas in this addendum, just a bit more mathematical detail)--- Take the radius of the earth to be $1$, and suppose both satellites travel at speed $v$ with respect to an earthbound observer I will call Jack.

Then according to Jack: At time $t$, satellite $A$ is over the point $(\cos(vt),\sin(vt))$ while satellite $B$ is over the point $(\cos(vt),-\sin(vt))$. They synchronize their clocks to $0$ at time $0$, when they are both over the point $(0,1)$.

When the satellites pass each other again (according to Jack) at time $\pi/v$ and location $(0,-1)$. According to either satellite, whose velocity with respect to Jack is $(v,0)$, this event takes place at time

$$T_0={\pi\over v\sqrt{1-v^2}}$$

(That is, this expression is, according to either satellite, the time interval between their first crossing and their second crossing.)

But at the event of the second crossing, the time shown on either satellite's clock is the length of the path $t\mapsto (t,\cos(vt),\sin(vt))$, which is

$$T_1=\int_{0}^{\pi/v} (1-v^2)dt={\pi(1-v^2)\over v}=(1-v^2)^{3/2}T_0 < T_0$$

Thus, at the moment of their second passing, each satellite says

We synchronized our clocks $T_0$ minutes ago, but now our clocks both show time $T_1$, which is less than $T_0$. That's because both of our clocks have been running slow, by different amounts at different times. At this particular moment, my own clock is keeping perfect time, but his is running slow by a factor of $(1-v^2)/(1+v^2)$.

Answer 5

It is a standard observation in special relativity that for A and B having constant velocity relatively to each other (and no acceleration), both A and B experiment the same thing and see the other's clock running slower: the situation is perfectly symmetric. Here, in your case, the situation is similar, the symmetry is perfect and thus each time A and B cross each other, their clocks will be perfectly synchronized.

Answer 6

If we peel this back to the original heart it comes down to this: Take 2 spacecraft orbiting a single mass in opposite circular directions and both experiencing zero g.

There appears to be a general consensus that as the ships pass each other they will both see the others' clock passing time slower than their own clock. Let them synchronise their clocks in the instant they pass. Clearly, that being the case, a moment later both ships would observe the others' clock to be running very slightly behind their own. Yet there appears to be a general consensus that when the ships next pass they will each observe their clocks to be synchronised once again. All statements allowing for Doppler effects of course.

So... if A is to observe B's clock catch up up with its own there must be a period during which A will observe B's clock to be running faster than its own. Similarly if B is to observe A's clock catch up up with its own there must be a period during which B will observe A's clock to be running faster than its own. In this scenario both ships are experiencing zero g so we're left only with the ships relative velocities - and that difference drops momentarily to zero when the 2 ships are opposite one another so that's not going to resolve the apparent paradox.

The only answer I can come up with relates to each ship's observation of the other ships' orbit.

I think they will each observe the other ship to be in an elliptical orbit. Furthermore, as they each carry out their multiple mid-orbit calculations to monitor the other ships position and "clock time when the light left the other ship" they will indeed observe the other clock to be passing time faster than their own peaking at the point where they are opposite one-another. At that point they will both observe the other to be further from the object being orbited than they themselves are.

Reasonable?

Answer 7

Let us consider the picture.

Satellite says: "The mirror is ahead of me, the other satellite is time dilated, I receive signals at normal rate because the Doppler shift cancels out the slowness of the other satellite's clock."

A simplified version: ground control at the north pole sends signals to an orbiting satellite. Satellite says: "The ground control station is ahead of me, the station is time dilated, I receive signals at extra fast rate because the Doppler shift exceeds the slowness of the ground station's clock.

The reason the satellite says the north pole is ahead of it is the so called aberration effect. https://en.wikipedia.org/wiki/Aberration_(astronomy)#Apparent_and_true_positions

Answer 8

To get an intuitive feel for what happens in this case, we can compare it with the "third frame" resolution of the twin paradox. In that resolution, when the traveling twin turns around, he hops into another reference frame. Before the turn-around he is in a frame that is moving away from the twin that stayed home. After the turn-around he is in a frame that is moving towards the home-twin. That new frame has a different perception about what the home-twin's clock shows. It is as if the turn-around suddenly moves the home-twin's clock forward, at least in the traveling twin's perception.

Something similar happens here. Due to the relative speed between the satellites, each satellite should see the other's clock run more slowly. But each satellite is also changing its direction the whole time. In a sense, it is constantly hopping onto a new reference frame that is moving in a slightly different direction. Each of those hops causes the (perception of) the other clock to jump forwards in the same manner as for the turn-around in the twin-paradox. Because this "frame hopping" is a continuous process, that results in a perceived higher clock rate of the other clock.

Then end result should be checked by somebody with General Relativity knowledge. But based on these arguments, I would predict that they perceive each other's clocks to be running at a slower rate at the moment they pass each other. But that is compensated for by a faster perceived rate when they are at opposite sides of the planet.

Answer 9

The arrangement you describe is entirely symmetrical between A and B so the elapsed time for an orbit will be the same for each satellite.

Your question about how can both clocks be running slower than the other is based on a common misconception about the nature of time dilation. Time dilation is another entirely symmetrical effect in SR and results from the fact that two reference frames moving relative to each other have tilted planes of constant time. A level plane of time in one frame corresponds to a sloping slice through time in the other, the slope being upwards in the direction of motion. This means that if you synchronise your watch at t'=t=0 with a passing clock in the other frame, at that instant, when it is t'=0 everywhere in your frame, it is already later than t=0 everywhere ahead of you, the value of t increasing with distance.

Imagine you are travelling at set your watch at t=0, and at some point ahead of you there is a person whose watch is set 1s ahead of yours. If you then take 100s to reach that person, you will find that their watch reads 101s when you get there, not because it ticks faster than your watch, but because it was already showing 1s when you started your journey. That is broadly analogous to the way in which time dilation rises in SR. It is not because moving clocks slow down, but that the path they take through spacetime has a shorter elapsed time which they correctly measure as being less. This is a key point to remember about time dilation if you want to avoid various logical conflicts based on misconceptions. It arises not because one clock runs slower than another, but because a moving clock is compared against successive clocks in the other frame which are progressively out of synch with the time in the frame of the moving clock.

The example you gave is based on a misconception that each clock runs more slowly than the other, which is logically impossible. The fact that the satellites are constantly accelerating makes the set-up more complicated and therefore easier to misunderstand, but you can reduce it to a thought experiment in which runners run in opposite directions around a square, and if you like you can replace the square with a hexagon and then with an octagon and so on until you end up with a circle- the physics always remains the same.

And in response to some of the other comments, the arrangement you describe is in principle no different to a version of the twin paradox in which both twins travel out and back in opposite directions and have aged the same amount when they re-meet, in spite of the fact that each has constantly been time dilated in the frame of the other throughout the journey.

Answer 10

The correct answer is, both clock would tick at identical speed, since their absolute speed is identical (7.9 km/sec). Absolute speed is the one making the clocks tick slower, not relative speed. Special Relativity is wrong. It produces usable results only when you compare high-speed vs low-speed objects, where absolute and relative speeds are similar.

In your case the objects have identical orbital speed, and orbital speeds are absolute. You don't need another object to measure orbital speed, so rotational speed doesn't need relativity (it's absolute). Hence, using rotation is the easiest way to refute relativity.

This doesn't mean Lorentz transformation is wrong. It's correct, but has to be used with absolute speeds, not relative. In other words, clocks on satellites tick slower than clocks on Earth's surface.

Why? Because satellites orbit the Earth at 7.9 km/s to keep their orbit, while surface of the planet, at equator, travels at 0.46 km/s. So you have a difference of 7.4 km/s between the surface and satellite, which makes satellite clock slower.

However, satellites have the equally ticking clocks since they all travel at the same speed. This is why time difference won't accumulate. There is no time-dilation between the satellites. Their atomic clocks tick at the same rate, because satellites have the same speed.

However, relativists refuse to accept this simple truth and are ready to introduce the most complex explanations and formulas to attempt to keep the dead theory alive. It's not possible. Special Relativity is wrong, but Lorentz transformations using absolute speeds are still used to adjust GPS devices and other measurements.