In a Time Varying Magnetic Field like the one shown in the picture below,
if we connect two points on the closed conducting loop using a conducting wire, why is the current flowing zero ?
My textbook says something like "the emf and the potential drop across the connected points will cancel each other out". But I could not understand the meaning of the statement. Can someone please clarify ?
Superposition Theorem: The total current in any part of a linear circuit equals the algebraic sum of the currents produced by each source separately.
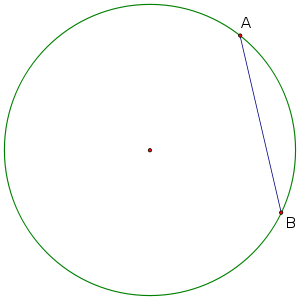
Let resistance per unit angle subtended at the center (in radians) of conducting loop be $\lambda$.
Total EMF ($E_{total}$ ) around a circular path in a TVMF is $ \dfrac{d\phi}{dt}=A \dfrac{dB}{dt} $ (where $A$ is the area of the circle)
We join points A and B on the loop. Suppose AB subtends an angle $\theta$ at the centre C.
Now considering only minor arc :
$E_{AB,minor}=\dfrac{E_{total}\theta}{2\pi}$
$i_{AB,minor}=\dfrac{E_{total}\theta}{2\pi \lambda \theta}=\dfrac{E_{total}}{2\pi \lambda }$
$E_{AB,major}=\dfrac{E_{total}(2\pi-\theta)}{2\pi}$
$i_{AB,major}=\dfrac{E_{total}(2\pi-\theta)}{2\pi \lambda (2\pi-\theta)}=\dfrac{E_{total}}{2\pi \lambda }$
Now, in the connecting wire AB currents $i_{AB,major}$ and $i_{AB,minor}$ are in opposite directions and cancel each other out, giving a net current of $0$ in the wire by Superposition Theorem.
