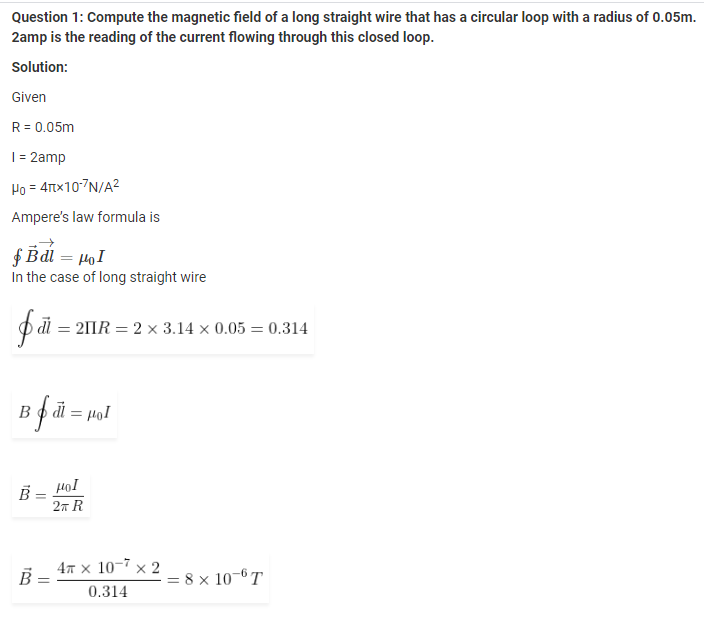Magnetic Field of a current carrying loop

Question

The numerical is given below:

Q: Compute the magnetic field of a long straight wire that has a circular loop with a radius of 0.05m. 2amp is the reading of the current flowing through this closed loop.

My teacher solved the above numerical as follows:

But I have a question that is:

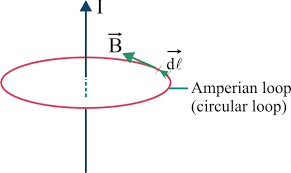
Q: In ampere's law, there is a dot product of B and dl so where is cosθ? If Cosθ is not here, this means Cosθ = 1 which means that θ = 0° but how is θ = 0° when the B points outward or into the loop and dl is the small piece of the circumference of the loop?

If the solution is correct, please explain it and give the answer to my above query. If not correct, then please provide the correct solution to the problem.

EDIT:
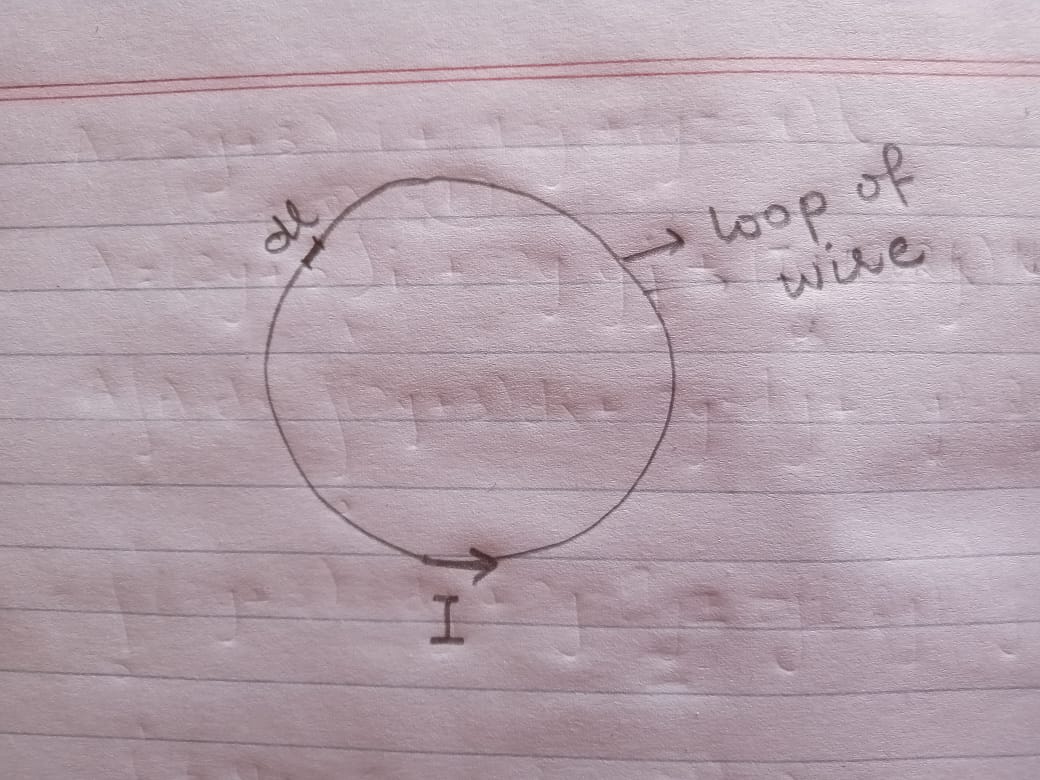
There is no figure included in the numerical. We have to make ourselves. I think the figure of wire might be like this if the current loop itself is supposed as amperian loop:

Answer 1

The original question doesn't make sense with respect to the solution. The question should have been like,

Compute the magnitude of magnetic field due to a long straight wire at a distance of $0.05\mathrm m$ from it, given that the current in the wire is $2A$

You now see that the thing called as "loop" in the original is an imaginary loop known as amperian loop but not a wire.

Q: In ampere's law, there is a dot product of B and dl so where is cosθ? If Cosθ is not here, this means Cosθ = 1 which means that θ = 0° but how is θ = 0° when the B points outward or into the loop and dl is the small piece of the circumference of the loop?

You could see from the figure that indeed $\cos\theta = 1$, this video may be used for further information on ampere's law

Answer 2

The way I read this question, a straight wire comes in from infinity, makes a circular loop, and then continues on out to infinity (in the same direction). Given this, there would be two contributions to the field at the center of the loop. One from the straight segments which they calculated using Ampere's law, and one from the loop, which cannot be calculated using Ampere's law.