I'm only just now starting to get my fingertips around Feynman diagrams, but I have an intuitive question: if I see a Feynman diagram that is valid insofar as it correctly expresses some physical process, does that mean all of the symmetries are also guaranteed to be possible in the scope of the same law?
In other words, if I have a process that goes from left to right in time, does the opposite process necessarily exist, and so on in the spatial direction(s)?
From Wikipedia Feynman Diagram
In other words, if I have a process that goes from left to right in time, does the opposite process necessarily exist, and so on in the spatial direction(s)?
I am assuming you mean, in effect do Feynman Diagram represent time reversible processes and I would say no, (or at least for the reasons below you would need to be very careful in your assumptions) but this link Perturbation Theory might provide you with a better informed answer.
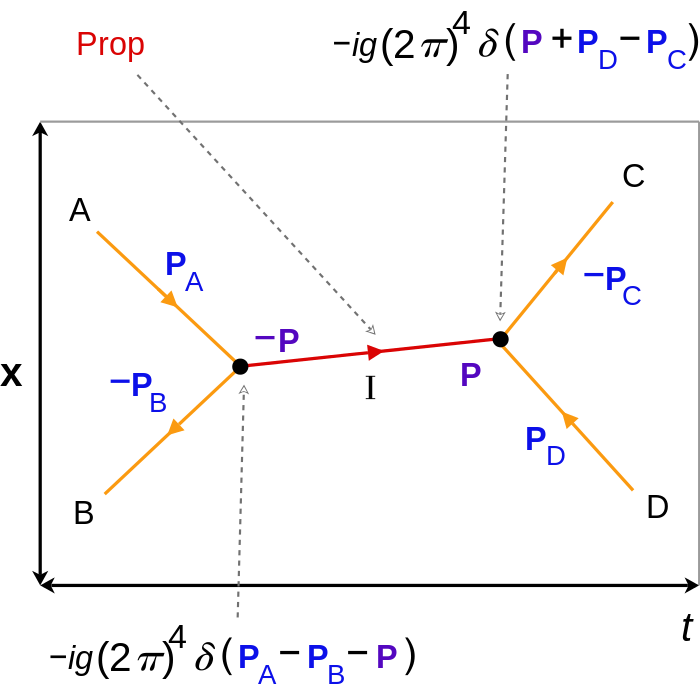
General features of the scattering process A + B → C + D:
• internal lines (red) for intermediate particles and processes, which has a propagator factor ("prop"), external lines (orange) for incoming/outgoing particles to/from vertices (black),
• at each vertex there is 4-momentum conservation using delta functions, 4-momenta entering the vertex are positive while those leaving are negative, the factors at each vertex and internal line are multiplied in the amplitude integral,
I am starting to learn this myself, and I would remind you that, say for Moller scattering, you need two diagrams, as you cannot be sure what process actually occurred.
For this reason, and because:
As you know already, the lines are in no way representative of paths or trajectories in reality.
Also the fact that the directions of external lines correspond to the passage of time.
The fact that space and time arrows are not always shown.
Diagrams can be left to right or up and down,
I would guess you need to be very careful with symmetry assumptions.
My apologies if I have made the wrong assumption regarding your question.
