Usually, in the papers the electronic band structure for monolayers $WS_2$ is something like in the figure below:
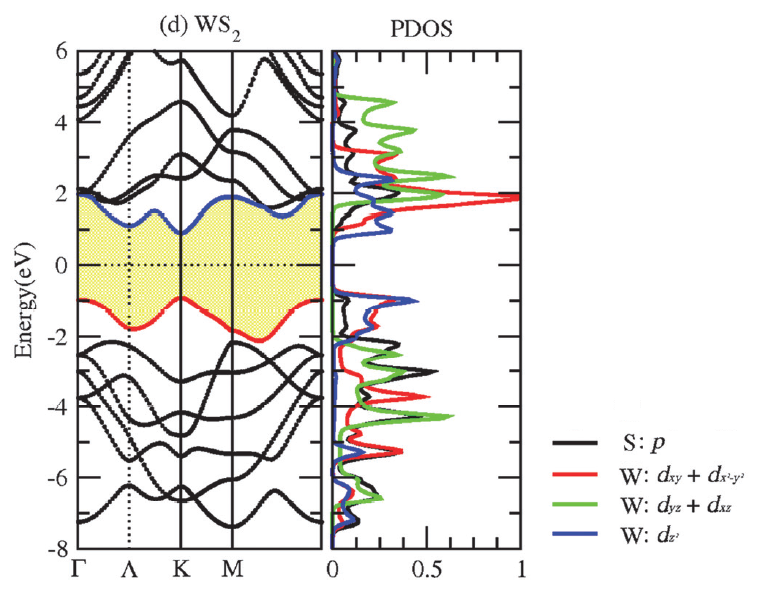As you can see the direct bandgap is around ~2.0 eV.
When we excite electrons at the K point they eventually form excitons. The binding energy of excitons in these materials is known to be around ~0.5 eV.
However, it has also been found that the direct energy gap at the K point is about ~2.5 eV. And photoluminescence (PL) emission was observed at the frequencies correspond to ~2.0 eV, which is energy band gap, obtained by first-principles calculations (DFT, etc.). The PL signal is supposed to come from excitonic recombination process. So the question is then, do the diagrams (like presented above) represent electronic band structure or excitonic?
I am asking this question because I'm not familiar with the details of the different first-principles approaches to calculate band structures. Maybe these approaches already include excitonic effects, so they actually do not show the actual electronic band structures, but, instead, they show the band structures modified in some way by excitonic effects.
1 Answer
In general, or as far as I'm aware, the band diagrams and Density of States shown in your question are electronic band structures. You can also see this from the graph's legend which shows that you're dealing with d and p orbitals belonging to W and S. However, looking at the numbers in your question, I can see where the confusion comes from.
The direct band gap of monolayer WS$_2$ has been proven to be about 2.5 eV. Because the binding energy of your exciton is 0.5 eV, the exciton will have a total energy of 2.0 eV. This is the 2.0 eV you extract from your PL measurements because your PL measurement originates from exciton recombination. This has nothing to do with the 2.0 eV from the Density Functional Theory (or DFT) calculation and it's just mere coincidence.
Underestimation of the band gap of solids is a known problem in DFT. This is also described in the following article:
Perdew, J. P. (1985), Density functional theory and the band gap problem. Int. J. Quantum Chem., 28: 497–523.
Without more information about the exchange-correlation functional used for this specific calculation it's difficult to guess how big the deviation would be.
