Does this shadow diffraction effect have a name?

Question

I noticed a cool diffraction effect today as I was sitting outside in bright sunshine: Suppose you have a fixed edge casting a shadow. If you put your hand on the side of the edge nearer to the shadow and move your hand so that its shadow just touches the shadow of the edge, you will see that your hand's shadow gets slightly wider when it is very close to the shadow of the edge. However, if you put your hand on the side of the edge away from the shadow, then it is the edge's shadow that gets wider.

(Aside: It occurs to me that the finite angular size of the sun may be significant for this effect. I suspect the same would be true of a point source, but I haven't investigated this yet.)

My questions are: Does this effect have a name, and can someone point me to a mathematical analysis of it? I would expect such a basic phenomenon would have been analyzed long ago, but when I try to search for it I just find a bunch of papers on CGI shadows (not what I want).

(Of course, if no one has done the analysis on this, please tell me, and I'll gladly be the first :)

Edit: In response to the suggested duplicate (Why do shadows from the sun join each other when near enough?), the effect I am describing is different because it depends in an essential way on the separation of the two shadow-casting objects in the third dimension. A third case that I didn't mention above is where your hand is at the same distance from the shadow as the edge, in which case we return to the two-dimensional problem referred to in the linked question.

Edit 2: Here's a picture of the setup:

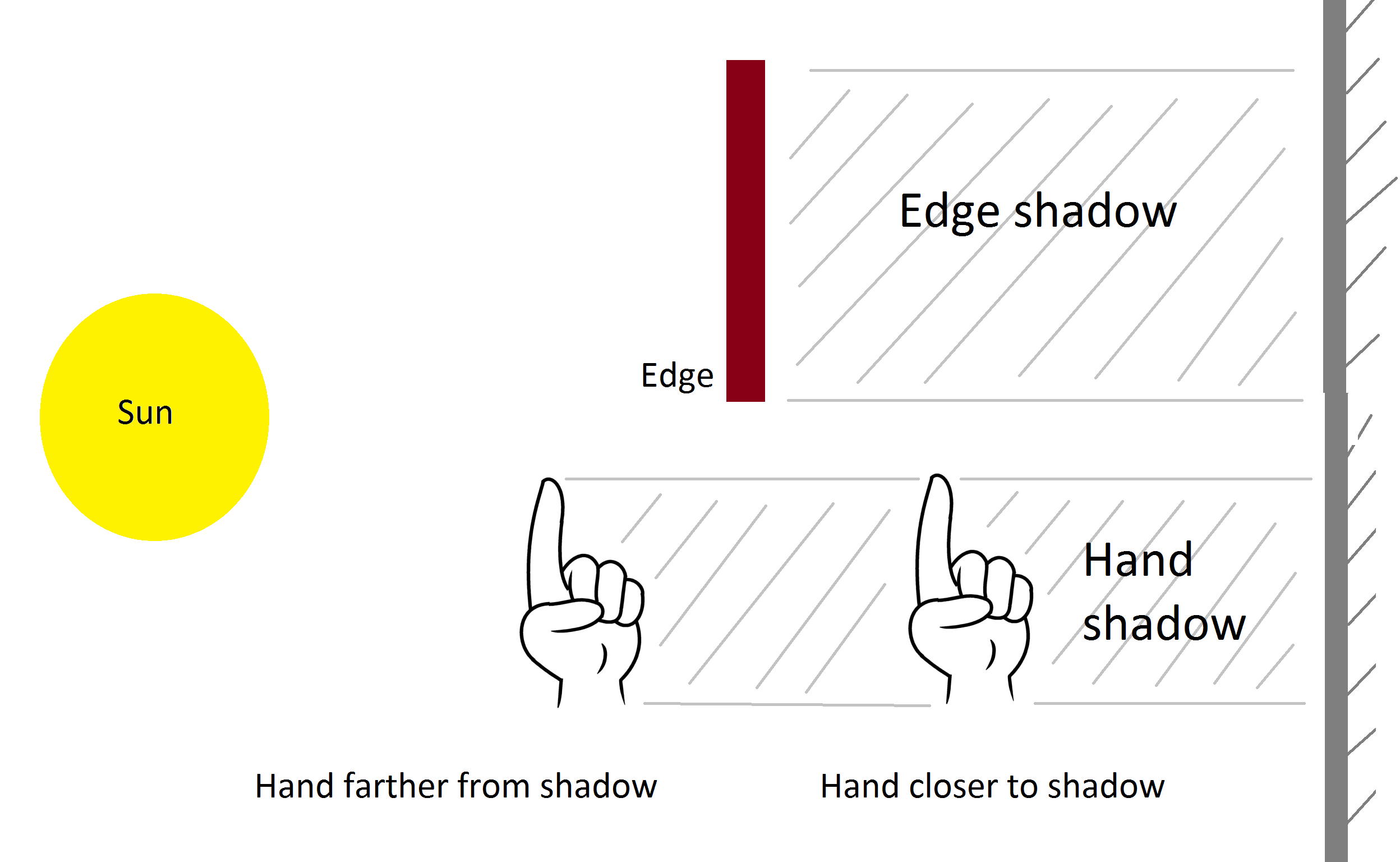
When your hand is closer to the shadow than the edge, your hand's shadow bulges towards the edge shadow as the two get very close. When the edge is closer to the shadow than your hand, the edge's shadow bulges toward your hand's shadow.

Answer 1

According to Wikipedia, this effect is known as shadow blister effect. They have very nice animation which explains the phenomena using ray optics: Here's the link.

From the explanation:

The effect takes place when two objects are located at varying distances between a non-point light source and a backdrop upon which their shadows are cast. As the two objects move transversely such that their shadows approach each other, the object nearest the light source will begin blocking light from reaching the inside of the other object's penumbra, thereby expanding its umbra. This expansion of the further object's umbra will continue until the umbras of both objects meet.

I recommend just look at the animation it will really clear things up.

Answer 2

If you look at two fingers, at different distances, then bring the fingers together until the edges appear about to overlap, you will see the effect. The effect is MUCH stronger if you move your fingers close enough to your eye that you cannot focus on either one.

If you do the experiment carefully, you will see that you only see the effect when the fingers are is not in focus. If you do the same experiment with a pinhole mask in front of your eye, you will see that the effect is dramatically reduced.

If you use a diffuse light source and cast slightly blurry shadows from your two fingers onto a white card, you will see the effect strongly. But change to a point source and do the same experiment, and you won't see the effect at all.

Those facts all show that the effect is not due to diffraction or interference, it is purely due to geometric optics.

Answer 3

We know light diffracts into the geometrical shadow, and as a result the "diffraction pattern/shadow" extends beyond the geometrical edge of each object. That effect can explain many such phenomena. See Spot of Arago. However, some similar effects also occur in a purely geometrical manner due to an extended light source like the Sun. See Applied Optics 38(9), 1573. BOTH effects probably contribute to your experiment, and I haven't yet found an analysis that includes them both. BTW, diffraction occurs regardless of the coherence of the light and the size of the object.

Answer 4

Fresnel diffraction by a straight edge.

Take $v = y \cdot \sqrt{2~/~(550nm \cdot h)}$ where $y$ is displacement of geometrical edge at shadow side, $h$ is distance between the edge and the shadow plane, and $550nm$ is wavelength of a typical visible light.

Then diffraction patter will shift the edge into shadow side by approximately $v=-2$ and into the illuminated region by approximately $v=+1.25$, due to theory of Fresnel diffraction by a straight edge.

From these you may compute $\Delta y$ by taking $\Delta v = 1.25-(-2) = 3.25$, divided by $\sqrt{2~/~(550nm \cdot h)}$. Such derived $\Delta y$ will be a good estimate of conceived "blurring width" of the shadow edge.

For example if $h = 10 m$ (height of tree leaves for instance), $\Delta y$ will be $5.4mm$, and if $h=50cm$ (height of your hand), $\Delta y$ will be $1.2mm$.