4
$\begingroup$

Following experimental setup.

We take copper coils which are small enough to be subject to brownian motion. We combine those coils with some other material to make them about as heavy as the liquid we submerge them in (same weight per volume) so they would neither all gather up at the top nor bottom of the liquid but rather spread out evenly.

Through permanent magnets we create a static magnetic field within the vicinity of the liquid.

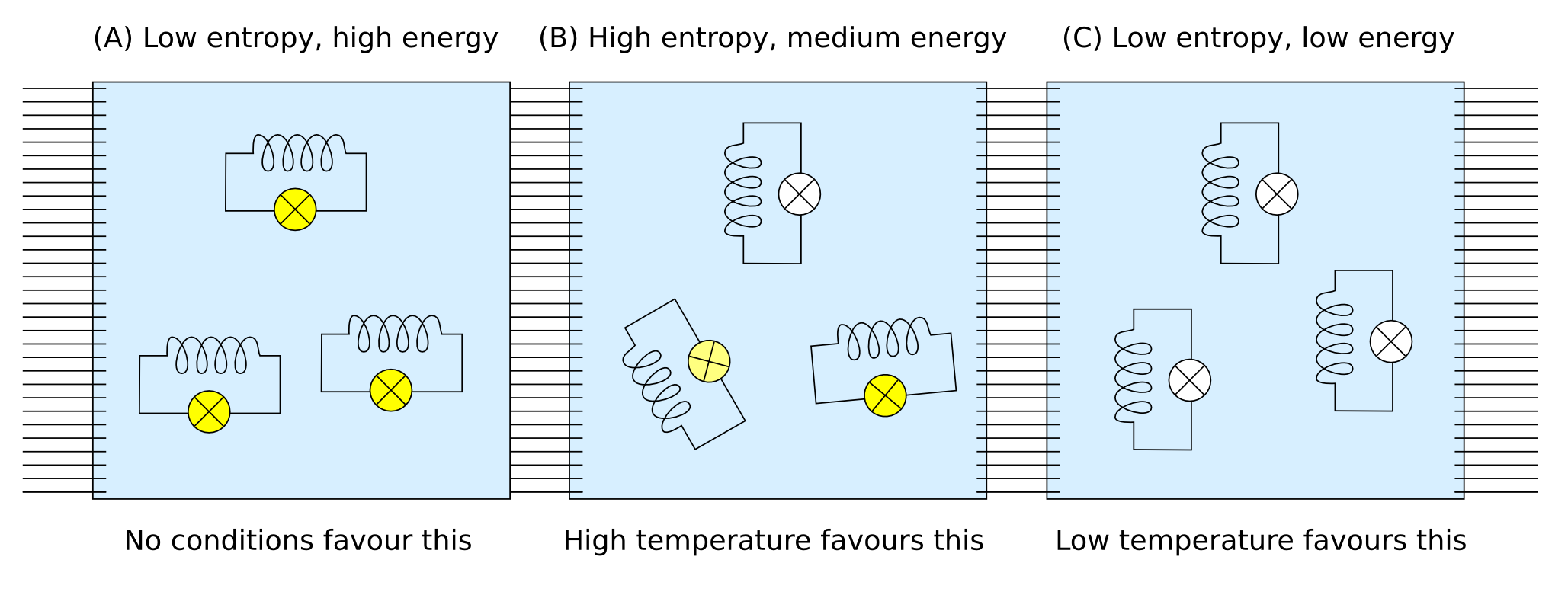
When the coils are moved around randomly through brownian motion inside the liquid, wouldn't this induce some current? Wouldn't this basically resemble a magnetic brake which would result in cooling down the liquid? A refrigerator working without any outside source, other than the energy of particles moving around randomly and pushing the coils around randomly?

As a bonus, one could possibly imagine to use the current the coils are subject to when moving through the magnetic field in a way which would have them emit some electromagnetic waves in the spectrum of visible light. Possibly allowing us to see a glow in a dark room.

Since this would most likely violate the second law of Thermodynamics, and we cannot have that, my question is, what part of this experiment would not work as i imagine it?

Note that this experiment was proposed the other way around, where we would rather use nano magnets being moved through brownian motion, but those would clump together into a bigger magnet, so i was just thinking that instead of moving magnets, one could just move coils through a magnetic field if coils that small in the nano/micro size range could actually be built.

Improve this question
$\endgroup$
1

2 Answers 2

Reset to default
8
$\begingroup$

When the coils are moved around randomly through brownian motion inside the liquid, wouldn't this induce some current?

Yes.

Wouldn't this basically resemble a magnetic brake

Accounting for the fact that copper has a nonzero resistance, then the thermal energy of the water causes the coils to move, this causes induced current, and this current is dissipated as heat in the coils (see Joule heating). In other words, thermal energy of the liquid turns into heat in the copper coils. So yes, there is a braking action going on, but...

which would result in cooling down the liquid?

No. The heat flow goes in both directions, from water to coil and from coil to water.

As the coil gets hot it's still in contact with the water, so of course if the temperature of the copper fluctuates above that of the water, then we get net heat flow from copper to water, thus keeping everything in equilibrium.

In fact, even if you imagine thermally insulated wires which cannot conduct heat to the water, you still don't get cooling of the water. To dissipate the electrical energy as heat, the coils need some resistance. It turns out that resistors at any nonzero temperature make electrical noise. This electrical noise would cause the coils to jitter around, thus stirring up the water and heating it until the coils and water are all at the same temperature.

A refrigerator working without any outside source, other than the energy of particles moving around randomly and pushing the coils around randomly?

No. See previous point.

As a bonus, one could possibly imagine to use the current the coils are subject to when moving through the magnetic field in a way which would have them emit some electromagnetic waves in the spectrum of visible light. Possibly allowing us to see a glow in a dark room.

Sure, we could rig something so that the induced currents cause radiation, but that doesn't change anything. If the surrounding environment were colder than the water and coils, then the radiation would go out and warm up those surroundings. Meanwhile, the surroundings emit radiation of their own because they're at a nonzero temperature, and again once everything's at the same temperature the power flows between all the elements are exactly balanced. So, while the coils may "glow", the surroundings also "glow" right back at the coils and you still don't have a magic refrigerator.

Since this would most likely violate the second law of Thermodynamics, and we cannot have that, my question is, what part of this experiment would not work as I imagine it?

Well to review:

  1. Materials with resistance create electrical noise, so the coils warm up the water just as the water warms up the coils.

  2. Even background radiation has a temperature and if you wait for a while everything which can radiate at each other winds up with the same temperature.

Improve this answer
$\endgroup$
20
  • $\begingroup$ "So, while the coils may "glow", the surroundings also "glow" right back at the coils and you still don't have a magic refrigerator." Let's say you are correct and lemon is wrong. Wouldn't you have a magic lightbulb still? Hence turned brownian motion into some useful energy/work? $\endgroup$
    – pZombie
    Commented Feb 20, 2016 at 8:27
  • $\begingroup$ Rereading you, you seem to be saying that there actually is a cooling effect which however is countered same fast or faster by the environment passing back heat onto the water. What if we made the coils emit some frequency which heats up a certain object/liquid perfectly and used some heatpipe system to transport the heat out of the thermally shielded room. Wouldn't that cool down the whole room? $\endgroup$
    – pZombie
    Commented Feb 20, 2016 at 9:05
  • 2
    $\begingroup$ @pZombie Where is your "heat pipe" going? If the other end of the pipe is not at absolute zero temperature, then there's heat coming in from that other end. If the temperature of the other end is the same as the water and coils, then the heat flow out of the pipe is equal to the heat flow in from the pipe's end. You can't get around this. When you connect two systems they will always exchange heat. When their temperatures are equal then the outgoing and incoming heat to and from each system are all equal. $\endgroup$
    – DanielSank
    Commented Feb 20, 2016 at 10:06
  • $\begingroup$ @pZombie Regarding the "magic light bulb"... are you familiar with thermal radiation? All things at nonzero temperature emit electromagnetic radiation (i.e. light), so in a sense everything is a thermal (magic) light bulb. However, if two thermal light bulbs are at the same temperature, then they emit the same amount of power at each other and neither one heats up or cools down. You can easily verify what I'm saying is true by looking at a red hot electric stove top or by using an infra-red camera. $\endgroup$
    – DanielSank
    Commented Feb 20, 2016 at 10:10
  • 2
    $\begingroup$ @pZombie "as soon as i imagine both liquid at the same temperature, i imagine the liquid with the coils inside emitting more radiation because of coils emitting some extra if they were designed that way." /quote Ah, now I see the fundamental problem! Ok, here we go: the most important thing to remember here is that a (passive) system's ability to emit and absorb are always exactly equal. This is a deep, very important fact. So yes, in a sense the coils emit more than a liquid without coils, but they also absorb more! The same goes for the idea of different colors. $\endgroup$
    – DanielSank
    Commented Feb 26, 2016 at 0:33
-3
+50
$\begingroup$

The state of the nano-circuits can be classified as one of the following three:

enter image description here

State A outputs large amounts of energy but is a low entropy state and therefore (due to the free energy being $F=U-TS$) will never arise.

State B consists of high orientational disorder which will generate light (or some other energy output). Due to the high entropy, this will be the favoured configuration at elevated temperatures. You correctly suggest that the energy will be extracted from the kinetic energy of the water, causing the temperature to decrease.

As the temperature decreases, the water will jiggle less, reducing the energy yield of the nano-circuits. As the temperature decreases more and more, one of two things could happen. The nano-circuits may retain their high entropy state, but the lack of brownian motion eliminates the energy output. Or (in an ideal case) the nano-circuits will align in a low-energy state (C).

Improve this answer
$\endgroup$
4
  • 3
    $\begingroup$ This answer is incomplete. It says that at high temperature the coils will emit light and cool the water. That's only true if the surrounding electromagnetic environment is at a lower temperature than the water. If not, then the environment is shooting more radiation at the coils than the coils are shooting at the environment, and the water temperature will increase. If the temperatures are the same, then of course the net power exchange is zero. $\endgroup$
    – DanielSank
    Commented Feb 20, 2016 at 10:15
  • $\begingroup$ @lemon I believe your answer is correct still if the coils can be manufactured to emit some form of radiation which is easily absorbed by the material the container, containing the water, is made of. The material of the container would be chosen to absorb or even better allow the the form of radiation of the coils to pass through, yet would block most of the radiation coming in from the environment. Or any other of the imaginable ideas to have the coils do some useful work through brownian motion they are subject to. Waiting for more input on this topic still. $\endgroup$
    – pZombie
    Commented Feb 21, 2016 at 7:27
  • 1
    $\begingroup$ @pZombie You can say that the radiation from the coils leaves and takes heat energy away, but the reverse process in which the environment also sends thermal radiation back to the coils is there as well. Only if the outside environment is colder than the coils will the net power flow be away from the coils. This is just the old story that two things at different temperatures trade heat until they're at the same temperature, and here radiation is doing the job! $\endgroup$
    – DanielSank
    Commented Feb 21, 2016 at 19:13
  • 4
    $\begingroup$ For the future hapless reader who find this: you have to remember that the environment plays a role. The net power flow leaving the water/coil system depends on the relative temperature between the water/coil system and the environment. $\endgroup$
    – DanielSank
    Commented Feb 25, 2016 at 18:55

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.