Induced Electric field due to magnetic field in Faraday experiment

Question

Regarding this experiment where a magnet is moved in and out of a coil -(see the picture)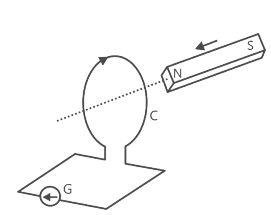

what i considered to be true is that when there is a changing magnetic flux through the coil(due to changing magnetic field coz area and orientation of coil is kept constant), it produces a circular Electric field around the coil which produces current in coil and that Electric field is given by faraday law as we all know very well. Also assume change in magnetic field to be as such that the current produced in coil is constant. Lastly this current produces a opposing magnetic field which opposes motion of bar magnet. Case Close

But now in this Answer-Click to Teleport

It says even if there is no current produced coz there is no coil there is stil some $\frac{\partial E}{\partial t}$ that produces the magnetic field. The problem is-(1)Why isn't this magnetic field in case we discussed above, was it missed or it wouldn't exist? (2) how different will the magnetic field produced by this $\frac{\partial E}{\partial t}$ be from the one produced by constant current in above case we discussed? (3) will this magnetic field be opposing the motion of magnet?

Answer 1

To start with question 3), the case where there is no coil but just a magnet that you move through empty space, as @user1245 asked in questionquestions/343447: is there a force opposing the motion? Yes there is, if the movement is not at constant velocity! Then there will be what is called "radiative reaction", or "radiation damping". Because you radiate energy creating EM waves (although very weak ones) there must be an opposing force.

This also happens for an electric dipole instead of a magnet and an even simpler case is one single particle with electric charge. See Radiation_damping, or Jackson's "Classical Electrodynamics", Chapt. 16. But even the simplest case is not trivial to compute and can lead to runaway solutions if you are not careful.

Your reasoning is basically correct that moving the magnet results in $d{\bf B}/dt$ and to have the matching $\nabla \times {\bf E}$ there must be a nonzero ${\bf E}$, which by itself will also be time-dependent so you have $d{\bf E}/dt$ and there should be a matching $\nabla \times {\bf B}$. Since the magnetostatic field of a stationary magnet has $\nabla \times {\bf B}=0$, there must indeed be some change in the shape of the ${\bf B}$-field.

As for your question 1), this radiative reaction is present also in the case with the coil present but its opposing force will usually be much weaker that that from the coil. For question 2): the change in field from the presence of the coil is usually much bigger than the departure from the magnetostatic field that you get by movement in empty space. (Unless we would talk about very strong acceleration, or a coil with extremely high resistance).

NB: we should be cautious in saying that the fields are "produced" or "caused" by each other. They must be present in a combination consistent with Maxwell's equations, but that doesn't prove whether the curl of one field causes the time derivative of the other, or the time derivative of one field causes the curl of the other.