Do people wearing glasses have different field of view than those who don't?

Question

There is one thing I sometimes wonder about ever since I was a child.

• Do people who wear eye glasses see objects in different size than those who don't?(Technically different size means different projected image size on the retina.)

• For example do myopic people who wear diverging lens see the world smaller, than a person with healthy eyes?

• Or conversely do hyperopic people who wear magnifying lenses to see sharp have a zoomed-in view of world?

EDIT: Sorry all, I wanted to mean "field of view" instead of line of sight.

Answer 1

Yes. I am myopic and I see a slight double image along the edge of my glasses:

This means that the field of view inside the frame is bigger - zoomed out - than what it would be with the same frames but without the lenses.

Similarly, objects are slightly but perceptibly smaller than they are without the glasses, as is clear from the size of the mugs at bottom left of the image. Zooming in on them and putting the inside- and outside-the-glasses versions in direct contrast gives a clearer picture:

As has been pointed out, the effect is strongly dependent on your exact position with respect to the lens, and it is exaggerated in the picture above because the camera is artificially far from the lens. Visually, and with the glasses correctly worn, the effect is milder and it looks closer to the door handle below:

For the handle to look like this I need to be about 2m from the door; I have about 2.5 dioptres in that direction if my memory serves.

---

In practice, though, your field of clear vision is restricted to the frame of the glasses, which do not cover a lot of your peripheral vision. This means that in practice you have a much reduced line of sight compared with someone without glasses, or who uses contact lenses. Whatever amplification was gained through this mechanism - the optics of which have been described in detail in other answers - is completely lost to this, and it's something to be aware of with people wearing glasses - our field of view is rather restricted, regardless of whether one is myopic or hyperopic.

Answer 2

Assume two people have identical (size) eyes, but one has a weaker lens than the other. In order to see an object at a certain distance properly, this person needs a second lens to get the focus. This lens will typically be some distance in front of the eye (not touching). If I understand your question correctly, you are asking whether that results in an image on the retina that is the same size, or not.

The simplest way to analyze this is to assume that the person who needs glasses has "no lens at all" - that is, their glasses have to take the role of the lens in the eye. In that case, it's easy to see that if the focal length of the lens-in-the-eye of the normal eye is $f$, and the glasses are $d$ away from the optical center of the lens, then the lens for the second person has a focal length of $f+d$. Now the magnification is approximately proportional to the focal length (for objects that are much further away than the focal length of the object), so in this case the second person (with the glasses) sees an image that is $\frac{f+d}{f}$ times larger than the person with the healthy eye. In reality, the second lens is only contributing a small amount to the focusing, and therefore the magnification will be much smaller.

That is the case for a person with hyperopia (farsightedness). The same analysis for a person with myopia (nearsightedness) will show that they see a slightly smaller image than the person with the healthy eye.

UPDATE While the above explanation is nice for giving a quick intuitive answer, I realize that it might be helpful to show the math for a two-lens system. I will do it with similar assumptions as I used earlier: the size of the eye is unchanged, and an object at a certain distance $d_1$ is imaged in focus on the retina; but in one case this is done with a single lens of focal length $f$, and in the second case there is an additional lens that is a distance $d$ in front of the eye; and it's the combination of the two lenses that produces an object in focus on the retina.

Here is the diagram for the unaided eye:

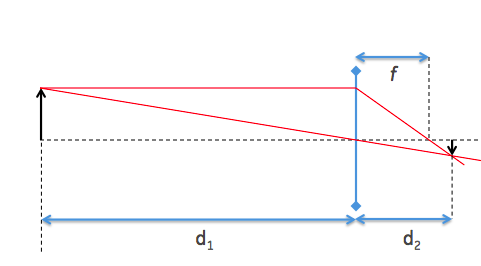
We know that $$\frac{1}{f}=\frac{1}{d_1}+\frac{1}{d_2}$$

and the magnification (minification) is

$$m = \frac{d_2}{d_1}$$

For the aided eye, the diagram looks like this:

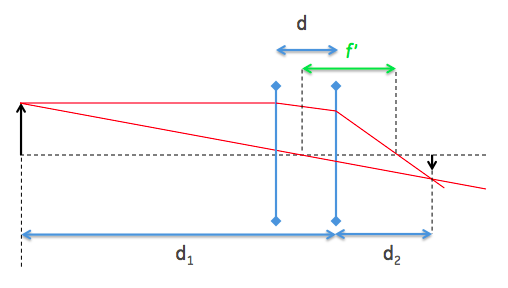
But it's quite hard to construct the optical rays properly without making a very confusing diagram. Therefore, I am going to split the analysis in two parts. First, I will demonstrate that the secondary lens produces a magnified image "further away" - and that this makes it possible for the weaker eye lens to focus. We can then compute the size of the image that the eye lens produces as though the other lens is not there:

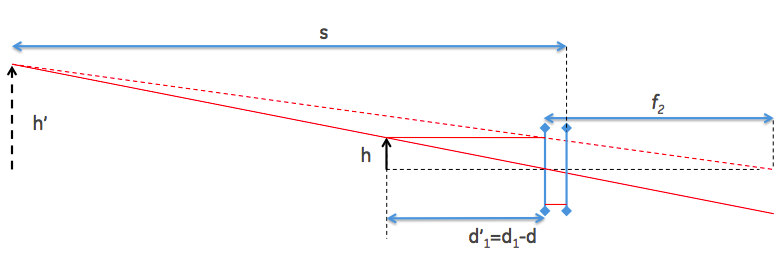
I constructed a virtual image of height $h'$ at some distance $s$ from the eye lens. For this, I have assumed a focal length of the eye $f_1$ (not shown), and focal length for the auxiliary lens $f_2$. The distance of the virtual image is calculated from the usual lens formula:

$$\frac{1}{f_2}=\frac{1}{d_1-d}+\frac{1}{s-d}$$

Solving for $s$, we obtain

$$s = \frac{f_2(d_1-d)}{(d_1-d)-f_2}+d$$

This will be a negative number for the situation drawn, where we have a virtual image (namely, the object is in focus on the "wrong" side of the lens).

The height $h'$ of the virtual object is given (from simple geometry) by

$$h' = h \frac{s-d}{d_1-d}$$

Now we can consider the size of an object of height $h'$, at a distance $s$ from a lens with focal length $f_1$. The object will be projected on the retina in-focus (that's how we defined $f_1$ and $f_2$), and the retina is still at distance $d_2$ as before. It follows that the size of the object on the retina is now

$$h' \frac{d_2}{s} = h\frac{s-d}{d_1-d}{d_2}{s}$$

We can do a first order expansion to find out how this is different from the size for the unaided eye, which was $h\frac{d_2}{d_1}$.

$$\begin{align}m&=h\frac{s-d}{d_1-d}\frac{d_2}{s}\\ &\approx h\left(1-\frac{d}{s}\right)\frac{d_2}{d_1}\left(1+\frac{d}{d_1}\right)\\ &\approx h\frac{d_2}{d_2}\left(1-\frac{d}{s}+\frac{d}{d_1}\right)\\ &\approx h\frac{d_2}{d_1}\left(1-\frac{d\left(f_2-d\right)}{f_2 d} + \frac{d}{d_1}\right)\\ &= h\frac{d_2}{d_1}\left(1+\frac{d}{f_2}\right)\end{align}$$

And there it is. The last term represents the additional magnification due to the auxiliary lens. It is directly related to the ratio of the focal length of the auxiliary lens and the distance between that lens and the eye. So if you have a +2 diopter lens (focal length = 50 cm) at 1 cm from the eye, you get a 2% change in apparent size (size of projected image on the retina is 2% larger). That is noticeable, but not massive. Negative focal lengths will make objects appear smaller according to the same formula.

Note that if you have a "good" eye, but choose to wear (reading) glasses anyway to see close up, the lens in your eye will relax, and let the external lens do more of the work. In the process, the image will get larger. And the further your push the glasses to the front of your nose, the greater the magnification. That effect is strongest with stronger glasses, since the additional magnification depends on the ratio of distance and focal length: if you put +3 diopter glasses at the tip of your nose (say 6 cm from your eye) you will get approximately a 20 % magnification

Some additional information about optical construction with compound lenses can be found at this link

Answer 3

The eye forms an image on the retina just as any lens does:

The eye is actually a compound lens because the cornea and the lens both play a role in the focussing, but let's ignore the cornea for now and just treat it as a simple lens. In that case the magnification is:

$$ M = \frac{v}{u} $$

where $u$ is the distance from the lens to the object and $v$ is the distance from the lens to the retina. Assuming a normal, myopic and hyperopic eye are looking at the same object at a distance $u$, any difference in the magnification will be due to a different distance $v$.

Myopia can be caused by the eyeball being too long, in which case $v$ is larger than normal eye and the magnification is therefore greater. Such people see objects slightly larger than normal people do. However myopia can also be caused by a too powerful lens, and the eye length is normal. In that case a myopia sufferer would see objects the same size as a normal person. So there is no simple relationship between being short sighted and the magnification in the eye.

The same applies to hyperopia (long sightedness). It can be due to the eye length being too short, in which case the magnification will be lower than normal, or to the lens power being too small in which case the magnification is normal. Again there is no simple relationship.

Note that the eyeball size increases as we grow up, so even among normal sighted people the magnification changes as we grow up.

Answer 4

You have to define what you mean by see objects as a given size. There is a lot of processing in the brain to estimate the size of an object. If you mean the size of the image on the retina, that is governed by the lens equation. You need the focal length of the lens system, including the natural lens in your eye and any accessory lenses you add, to be correct for the distance from the lens to the retina and the distance from the eye to the object. Added lenses are needed when the natural lens cannot reach the proper focal length. Myopic people have lenses that cannot get weak enough to focus at infinity, so need a diverging lens to achieve proper focus. To the extend we can collapse the lenses into one thin lens, the size of the image on the retina is determined by the diameter of the eye and the angular extent of the object. Someone with a larger eye will have a larger image

Answer 5

Yes, yes, yes.

Depending on the glasses and severity of the disorder they correct, they do make the world seem smaller. Due to shape of the lens, they also sometimes introduce aberrations in the image: For instance straight lines will look bent and so on.

The difference in size is readily perceptible. Due to various optical effects of the glass, depth perception is also affected. So when glasses are taken off objects will become smaller/larger, and even when one set of glasses is switched for another, some minor disorientation may occur for a brief time while the brain adjusts to the new distortion. Even if both glasses have the same optical characteristics, for instance one may sit farther away from the eyes.

You can also observe this by looking at people with glasses. Depending on what sort of lens they have, their eyes will look unusually large or small.

Answer 6

You are making two distinct and independent questions - 1) do eye glass(frames) affect the field of view of the person wearing them? 2) is the size of an object seen by people that wear glasses, different (larger/smaller) from the one seen by people who don't need glasses?
The answer to 1, is yes. The frame and its "legs" will restrict a person's field of view (a tunnel effect).
The answer to 2, is no. The purpose/effect of the eye glass, is to cause the object to be focused on the person's retina, thereby restoring normal vision.
If a person's eye focuses an object in front of its retina, it will see a slightly larger and blurry object. If it focuses the object behind the retina, it will see a slightly smaller and blurry object. If an eye glass is used to correct the problem, the eye will see the object sharp and in its "proper/normal" size.