Why is the magnetic field of a spherically symmetric current zero?

Question

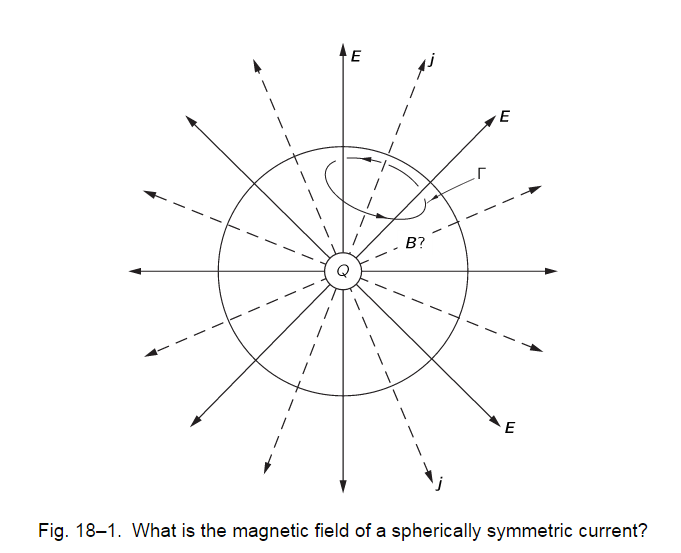
We now ask about the magnetic field produced by the currents in this situation. Suppose we draw some loop $\Gamma$ on a sphere of radius $r,$ as shown in Fig. 18–1. There is some current through this loop, so we might expect to find a magnetic field circulating in the direction shown.

But we are already in difficulty. How can the $\boldsymbol B$ have any particular direction on the sphere? A different choice of $\Gamma$ would allow us to conclude that its direction is exactly opposite to that shown. So how can there be any circulation of $\boldsymbol B$ around the currents?

This is an excerpt from Feynman's Lectures on Physics; here he explains how there exists no magnetic field for a spherically symmetric current & that he proves later using Maxwell's third equation including the term $\frac{\partial \boldsymbol E}{\partial t}$.

But I've not understood his reasoning; how by changing $\Gamma$, the direction of the magnetic field be different? I'm failing to visualise it.

So, could anyone please help me explain how by changing the loop, the direction of the magnetic field be reversed?

Answer 1

Let's assume (since you didn't say) that the charge in the middle is decreasing (getting more negative if negative, getting negative if zero, and decreasing if positive) and that the current density is radial outwards.

If you choose one orientation for the loop then the circulation of the magnetic field around that loop is proportional to the total current (electric and displacement) through the smaller enclosed surface, the one that looks like a small hat.

If you choose the opposite orientation for the loop then the circulation of the magnetic field around that loop is proportional to the total current (electric and displacement) through the larger enclosed surface the one that looks like all the other points on the surface except the small hat.

If the current is spherically symmetric and the electric field is spherically symmetric then it ($\vec J+\epsilon_0\partial \vec E/\partial t$) either has a positive outwards flux, a negative outwards flux, or a zero outwards flux.

If positive then when you take the flux of the small cap you get a positive flux and so a positive circulation. But same when you take the flux of the larger surface, the surface that is the whole sphere except the cap, so the circulation of the magnetic field in the opposite orientation also gives you a positive line integral. That's a contradiction because they should be negatives of each other.

The same happens if the flux is negative.

If the flux is zero, there isn't a problem. But this does not show that the magnetic field is zero. It shows that the displacement current and the electric current give a zero net flux.

You could place your spherical setup inside a huge solenoid and the magnetic field would not be zero. And anyway, these arguments about line integrals on a spherical surface tell you absolutely nothing about the radial component of the magnetic field.

Answer 2

This problem first comes up in the Feynman Lectures as an exercise for Chapter 3 (problem 39.3 in the blue edition). At that point Feynman is interested in getting a basic feel for the Maxwell equations and showing their consistency. From that point of view, the argument that the $\dot{\mathbf{E}}$ term cancels the $\mathbf{j}$ term in the curl $\mathbf{B}$ equation is interesting.

But Feynman failed to be clear about the reason why $\mathbf{B}=0$. The setup has rotational symmetry about $O$ the centre of the sphere. So $\mathbf{B}$ must be radial. [Take any point $P$ outside the sphere. Rotating about the axis $OP$ must leave $\mathbf{B}$ unchanged, so it must be radial.]

Now use the integral version of the grad $\mathbf{B}$ equation - integrating over the surface of the sphere radius $OP$ - to show that $\mathbf{B}=0$ (no magnetic monopoles).

[Incidentally, grad $\mathbf{B}$= curl $\mathbf{B}=0$ alone is not enough to prove that $\mathbf{B}=0$. ]

Answer 3

Let's break the magnetic field into a radial and angular part: $\vec{B}=B_r \hat{r}+\vec{B_\Omega}$. Let's also look at some sphere around the origin.

1. Why $B_r=0$:

$B_r$ is constant around the sphere, and thus the magnetic flux on it is $4\pi B_r$, but this must vanish due to $\nabla \cdot B=0$.

2. Why $\vec{B_\Omega}=0$:

$\vec{B_\Omega}$ is a vector field defined on the 2-sphere - meaning that it is always tangent to the sphere (by definition). According to the Hairy Ball Theorem, it vanishes somewhere. By spherical symmetry, it should vanish everywhere on the sphere. Thus $\vec{B_\Omega}=0$.