Magnetic field in a capacitor

Question

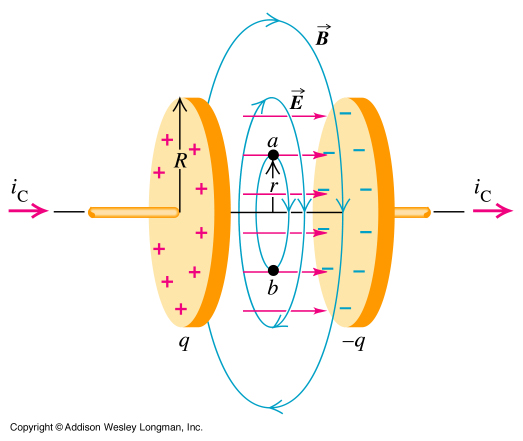
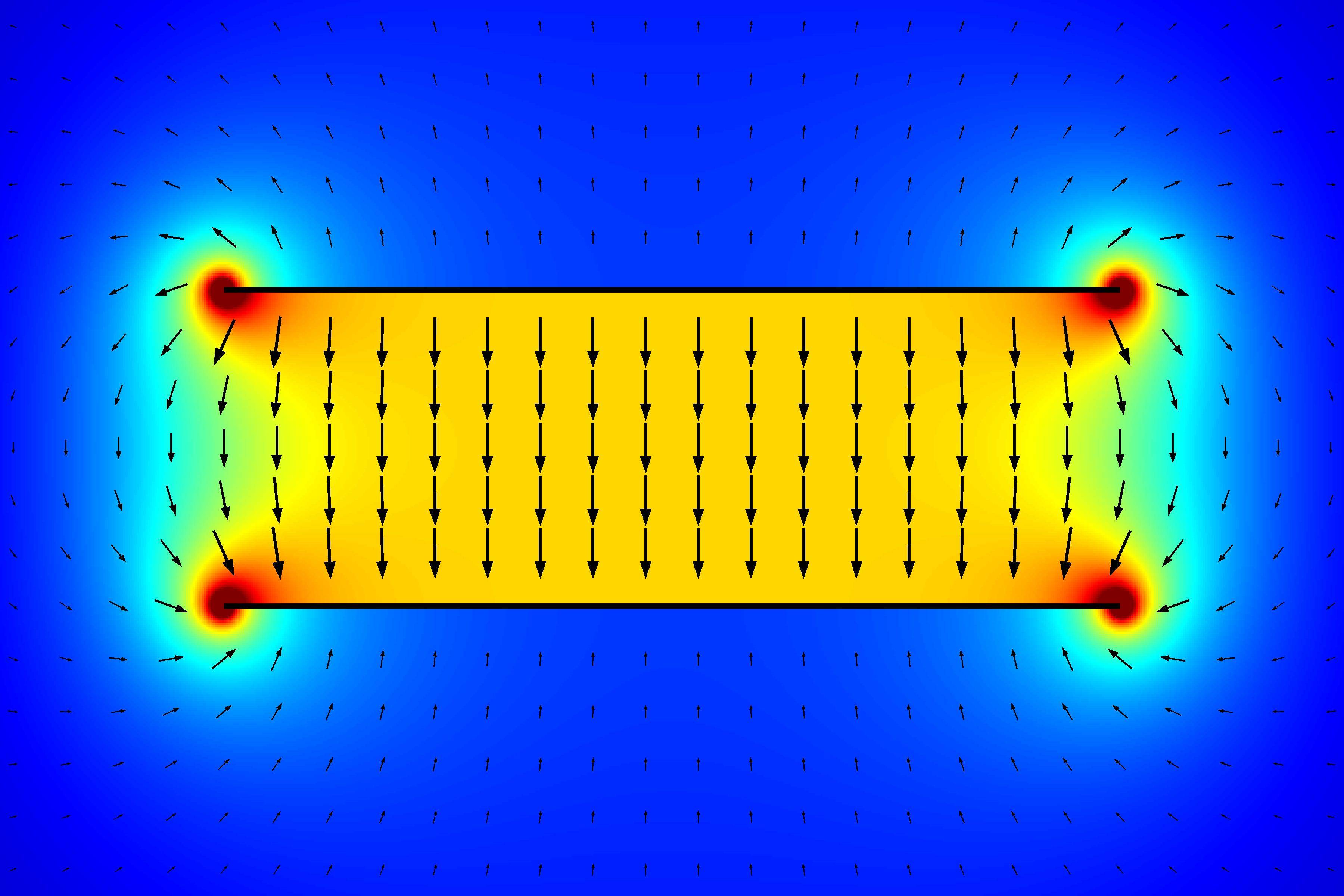
If in a flat capacitor, formed by two circular armatures of radius $R$, placed at a distance $d$, where $R$ and $d$ are expressed in metres (m), a variable potential difference is applied to the reinforcement over time and initially zero, a variable magnetic field $B$ is detected inside the capacitor. Neglecting the effects on board, at a distance $r$ from the symmetry axis of the capacitor, the intensity of $B$ varies with the law

$$\boxed{B(r)=\dfrac{kt}{\sqrt{(a^2+t^2)^3}}\,r} \quad r\leq R,\quad \tag{1}$$

where $a$ and $k$ are positive constants and $t$ is the time elapsed since the initial moment, expressed in seconds (s).

1. How can I prove the $(1)$?

2. Why is the direction of the electric field $E$ within the capacitor that of the symmetry axis and are the lines of the magnetic field generated by the displacement current concentric circular lines, with center on the symmetry axis, and lie on planes parallel to the reinforcements and perpendicular to the symmetry axis?

(The image is taken from this link: Magnetic field from displacement currents in a capacitor, and an applied exterior magnetic field)

Does exists a mathematical explanation?

3. What happens when $r>R$?

My considerations. Obviously from the previous condition $\boldsymbol{\mathrm{E}}$ and $\boldsymbol{\mathrm{B}}$ are perpendicular to each point.

I have thought that from the fourth Maxwell equation in differential form. Hence we have:

$$\boldsymbol{\nabla}\times \boldsymbol{\mathrm{B}}=\epsilon_0\mu_0\frac{\partial \boldsymbol{\mathrm{E}}}{\partial t}+\mu_0\boldsymbol{\mathrm{J}}$$

where the fourth equation of Maxwell in integral form becomes the theorem of Ampère-Maxwell:

$$\oint_\ell \boldsymbol{\mathrm{B}} \cdot d\boldsymbol{\mathrm{l}}=\mu_0 I_{\mathrm{enclosure}}=\sum_k\mu_0I_k=\mu_0(I_s+I_c)$$ where with $I_s$ we indicate the sum of all the displacement currents and with $I_c$ all the conduction currents. Remember that displacement current is given by

$$I_s=\epsilon_0\mu_0\frac{\partial \boldsymbol{\mathrm{E}}}{\partial t}$$ and being $\boldsymbol{\mathrm{J}}=\boldsymbol{\mathrm{0}}$ (because in the capacitor is it does it present a magnetic field even in the absence of magnets and conduction currents). In the region between the armatures, the theorem takes the following form:

$$\oint_\ell \boldsymbol{\mathrm{B}} \cdot d\boldsymbol{\mathrm{l}}=\epsilon_0\mu_0\frac{\partial \boldsymbol{\mathrm{E}}}{\partial t}$$ i.e. the circuitry $\Gamma(\boldsymbol{\mathrm{B}})$ is:

$$\oint_\ell \boldsymbol{\mathrm{B}} \cdot d\boldsymbol{\mathrm{l}}\equiv\Gamma(\boldsymbol{\mathrm{B}})=\epsilon_0\mu_0\frac{d\Phi(\boldsymbol{\mathrm{E})}}{\partial t}.$$

Answer 1

1. You can't without knowing the time dependence of the applied voltage. However I can work backwards and deduce the form of the voltage required to create such an magnetic field.

For a capacitor the charge density is $\sigma=\frac{Q}{A}$ where Q is the charge and A the area of a plate. The electric field is proportional to the charge density $E=\frac{\sigma}{\epsilon_0}$. This gives us $$\vec{E}=\frac{Q}{\epsilon_0 A}\vec{e}_z$$

If we substitute that into the maxwell equation (with current between plates = 0): $$\vec{\nabla} \times \vec{B}=\mu_0 \epsilon_0 \frac{\partial \vec{E}}{\partial t}=\frac{\mu_0}{A}\frac{d Q}{d t}\vec{e}_z$$

Due to the symmetry of the problem we can assume that the magnetic field has the form $$\vec{B}=B_\phi(r) \vec{e}_\phi$$

Therefore $$\vec{\nabla} \times \vec{B}=B_\phi(r)\vec{\nabla} \times \vec{e}_\phi=\frac{B_\phi(r)}{r} \vec{e}_z$$ (The easiest way to evaluate the curl is to look up curl in cylindrical coordinates.)

Then $$\Rightarrow B_\phi(r)=\frac{\mu_0 r}{A} \frac{dQ}{dt}$$ $$\vec{B}=\frac{\mu_0 r}{A} \frac{dQ}{dt}\vec{e}_z$$

To get the given magnetic field the voltage has to be $$U(t)=\frac{1}{C}Q(t)=\frac{1}{C}\int \frac{dQ}{dt}dt=\frac{1}{C}\int \frac{B(r)A}{\mu_0 r}dt=\frac{-k}{\sqrt{a^2+t^2}}\frac{A}{\mu_0}+\text{const.}$$

2. The electric field lines point from positive charges to negative charges. Remember that the electric field direction indicates the direction in which a positive test particle gets pushed. On the symmetry axis the contribution from all the charges on the plates cancel out in the direction perpendicular to the symmetry axis. Therefore on the symmetry axis the electric field is parallel to the axis. Away from the symmetry axis the electric field is only approximately parallel.

This is how the electric field looks like. The colors represent the electric field strength, with red being the strongest. (Source)

The magnetic field is circular, because a electric field which changes only its magnitude but not direction will produce a circular magnetic field around it. This is what the rotation in the maxwell equation is telling you.

3. Nothing special. You just can't use the approximation that the field lines are parallel anymore.