How can I determine a direction of force which is made by magnetic field and current with a wire? Fleming left hand rule seemingly not works at here

Question

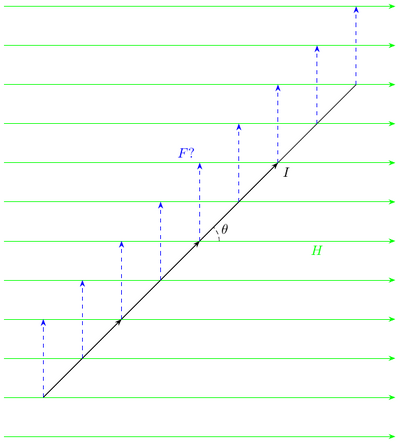
Please assume that the conductor of wire exists , and the current flows from below left to above right like shown in the above diagram.

As shown in the above diagram , the uniform magnetic fields are given .

I want to know the forces which act against the wire.

I tried to use the rule of Flemming left hand .

But this rule seemingly only be able to be used as an angle between a magnetic field and a current , is a right angle .

I know the below formula .

$$ \boldsymbol{f}= l \left( \boldsymbol{I}\times\boldsymbol{B} \right) $$

Hence, the below is held .

$$ \Vert \boldsymbol{f} \Vert = l \cdot \left(\Vert \boldsymbol{I} \Vert \cdot \Vert \boldsymbol{B} \Vert \cdot \sin^{}\left(\theta_{} \right) \right) $$

Just simply applying the above equation of magnitude can easily obtain the magnitude of total force which acts against the wire however how can I obtain the direction of the force vector?

I think the directions of it must be perpendicular against the directions of the magnetic fields but I don't know how to prove it so far.

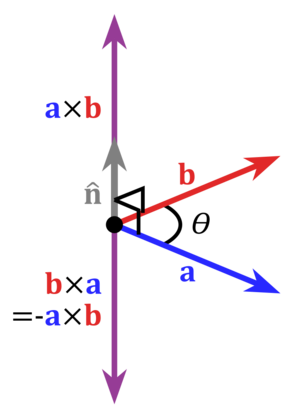
I looked the page of wikipidea of cross product and found the below image .

Cited it from here

Moreover how can I determine the vectors of forces as the directions of the magnetic fields are reversed?

Answer 1

I take the fingers of my right hand, and go from one vector into the other across the acute angle, often ninety degrees. My fingers would start pointing in the direction of the first vector I. Then curl into I becoming perpendicular to it and turning another ninety degrees to hit the next vector B. Then my thumb points down which is the resulting direction of cross product. You can experiment by realizing x direction cross y direction in standard 3D coordinates is in the z direction. (And z cross x is y, and z cross y is -x, and the opposite orders give opposite directions, ie y cross z is +x).

Yes the direction is always perpendicular to the plane of the two vectors being crossed. But that leaves two options for direction. Point fingers same as first vector. Curl them into being perpendicular to that first vector and heading toward the second vector, and right thumb points correct way.

---

Btw theres another situation where you know the axis, and the field or whatever circles around it. Like angular velocity vector, or angular acceleration, or the magnetic field made by a current in a wire (as distinct from force on a wire in a field).

In that case point your right thumb along the axis and your fingers curl around in the correct direction (the direction of the magnetic field encircling the wire, created by the current: or the way the thing is spinning if given angular velocity).