Turbulent spacetime from Einstein equation?

Question

It is well known that the fluid equations (Euler equation, Navier-Stokes, ...), being non-linear, may have highly turbulent solutions. Of course, these solutions are non-analytical. The laminar flow solutions (Couette flow for example) may be unstable to perturbations, depending on viscosity.

Also, low-viscosity fluids (water for example) are more turbulent than high-viscosity fluids (oil, for example).

I was wondering if something similar may happen with gravity and spacetime itself. The Einstein equations are highly non-linear: do turbulent solutions exist?

Or is gravity like some highly viscous fluid, i.e. without any turbulence?

What might a turbulent metric look like? Of course it would not be an analytic solution.

I imagine that spacetime turbulences may be relevant on a very large scale only (cosmological scales, or even at the Multiverse level). And maybe at the Planck scale too (quantum foam). But how could we define geometric turbulence?

The only reference I've found on this subject, which shows that the idea isn't crazy, is this :

https://www.perimeterinstitute.ca/news/turbulent-black-holes

---

EDIT : I have posted an answer below, which I think is very interesting. I don't know if this hypothesis was already studied before.

Answer 1

Gravity can, of course, become turbulent if it is coupled to a turbulent fluid. The interesting question is thus, as John Rennie points out, whether a vacuum solution can be "turbulent".

As far as I'm aware this is not known. If turbulence does occur in vacuum gravity, it is remarkably hard to stir up. Even in very extreme situations like colliding black hole binaries, which are now simulated pretty routinely, no turbulence has been observed.

EDIT: One approach one might take to study this is the "Post-Newtonian expansion", in which GR is formulated as an expansion in powers of some characteristic speed $\frac{v}{c}$. This has been conducted to extremely high order and the accuracy of the results at least for binary black holes rivals that of full nonlinear simulation. To all existing orders, the PN expansion is known to be exactly integrable. So if GR exhibits turbulent behaviour, it does so only in very extreme situations.

There are some theoretical reasons one might expect turbulence, which are hinted at in the press release you link to. Because of AdS/CFT one expects at least certain vacuum GR spacetimes to be equivalently modelled by a certain quantum field theory with a special symmetry. But that field theory, in some limit, should itself be approximately described by the Navier-Stokes equations. Therefore, again perhaps only in some weird and not-entirely-understood limit, the vacuum EFE's ought to described by the Navier-Stokes equations.

The point of the study you linked to was to investigate what sorts of behaviour in the gravitational theory one might get when the corresponding hydrodynamic theory is turbulent. The conclusion seems to be that certain turbulence-like behaviours appear in the gravitational theory. It seems to me a bit of an overstatement to say this group has discovered full-blown gravitational turbulence.

It's also, by the way, not yet known whether more pedestrian sorts of chaos can occur in the GR two-body problem. The Kerr spacetime is exactly integrable and geodesics are not chaotic. However, an actual particle will not move in the Kerr spacetime, but in a deformed spacetime including also its own gravitational field. An open question is if and when this perturbation can lead to chaotic motion.

EDIT2: There are also some theoretical reasons one might not expect turbulence. Basically what I'm imagining by turbulence is something like highly-nonlinear gravitational waves self-interacting strongly enough to excite vortex stretching, etc. But attempts to simulate such self-interactions (e.g. http://relativity.livingreviews.org/Articles/lrr-2007-5/) typically find that more or less generically, such strong gravitational fields either lead to rapid dispersal to infinity or the formation of a black hole. In closed spacetimes even small perturbations seem to eventually form a black hole more or less generically, although this is still unsettled. However, these studies are almost always done in high symmetry, so the question is far from resolved.

Answer 2

Thanks to holography, we now know that solutions to the Einstein equation in certain $d+1$ dimensional spaces are equivalent (dual) to solutions of the Navier-Stokes equation in $d$ dimensions. This is the fluid-gravity correspondence. As a result, turbulence can be studied using the Einstein equations, see, for example, http://arxiv.org/abs/1307.7267.

Answer 3

Update

Recently, there was a talk titled Turbulent gravity in asymptotically AdS spacetimes which may be of interest. In these papers, spacetimes which are anti-de Sitter asymptotically with reflecting boundary conditions are considered, and the notion of turbulence in this case is that small perturbations about these spacetimes exhibit 'turbulent behavior.'

The most relevant paper I think would be A Holographic Path to the Turbulent Side of Gravity which makes use of the gravity/fluid correspondence:

We study the dynamics of a 2+1 dimensional relativistic viscous conformal fluid in Minkowski spacetime. Such fluid solutions arise as duals, under the "gravity/fluid correspondence", to 3+1 dimensional asymptotically anti-de Sitter (AAdS) black brane solutions to the Einstein equation. We examine stability properties of shear flows, which correspond to hydrodynamic quasinormal modes of the black brane. We find that, for sufficiently high Reynolds number, the solution undergoes an inverse turbulent cascade to long wavelength modes.

This relates to the answer posted by Thomas.

---

There are indeed vacuum solutions to the Einstein field equations which are unstable under perturbations. A famous example is the result of Gregory and Laflamme for black strings, which essentially have the geometry of $\mathrm{Sch}_d \times \mathbb{R}$. For example, a five dimensional black string could have a metric,

$$ds^2 = \left( 1-\frac{2GM}{r}\right)dt^2 - \left( 1-\frac{2GM}{r}\right)^{-1}dr^2 - r^2 d\Omega_2^2 - d\sigma^2$$

where $\sigma$ is the additional fifth coordinate. Clearly, this metric will also satisfy the vacuum equations. Gregory and Laflamme showed that under a perturbation, $g_{ab} \to g_{ab} + h_{ab}$, the solution is unstable, and the instability itself is a tensor mode. (An argument is made to show there is no instability due to the scalar and vector modes.)

A subsequent paper by Lehner (who is in the article you linked) and Pretorius, Black Strings, Low Viscosity Fluids, and Violation of Cosmic Censorship (which I highly recommend) reveals that:

The [Gregory Laflamme] instability unfolds in a self-similar fashion, in which the horizon at any given time can be seen as thin strings connected by hyper-spherical black holes of different radii. As the evolution proceeds pieces of the string shrink while others give rise to further spherical black holes, and consequently the horizon develops a fractal structure. At this stage its overall topology is still $\mathbb R \times S^2$; the fractal geometry arises along $\mathbb R$...

Eventually, it shrinks to zero and you are left with a naked singularity. Of course, there are bound to be other unstable vacuum solutions, but this one in particular comes to mind, as it is also of relevance to cosmic censorship.

Answer 4

The fluid-gravity correspondence that Thomas referred to in his answer is a very concrete set-up where we can import intuition from fluid dynamics to suggest how we might get turbulence in vacuum GR (with negative cosmological constant). I thought it deserved more explanation.

First, fluid dynamics is a universal description, applicable in any system (e.g. water, the quark-gluon plasma, lump of metal,...), describing the regime of long wavelength fluctuations away from equilibrium. Its starting point is thermodynamics, which describes a system in equilibrium in terms of just a few variables (temperature T and chemical potential $\mu$), from which everything else (density, pressure, entropy density, ...) is determined. Fluids goes a step beyond that, by allowing the system to be far out of equilibrium, but still locally in equilibrium, so in any sufficiently small patch the system is well equilibrated, with some local temperature $T(x)$, chemical potential $\mu(x)$, and now velocity $\vec{u}(x)$ defining the local equilibrium rest frame. These functions must vary sufficiently slowly, for example over distances much longer than the molecular mean free path, so that thermodynamics is locally a good approximation. Technically, fluid dynamics is then a "derivative expansion", allowing terms in the equations of motion up to some given order. First order gives perfect fluids, second order introduces viscosity and gives Navier-Stokes and its generalisations. At each order, new `transport coefficients', like viscosities, must be introduced, but these are the only things that depend on the underlying theory.

This all applies to your favourite quantum field theory, and in particular to certain strongly interacting, relativistic, scale invariant theories that have alternative gravitational descriptions. In that context, equilibrium maps to a static, uniform black brane, and adding long-wavelength fluctuations of the horizon is equivalent to studying fluid dynamics in the field theory. In this approximation, Einstein's equations reduce exactly to relativistic Navier-Stokes, with some transport coefficients, and in particular very low viscosity (conjectured to be the lowest possible).

This means that much of what we're used to in fluids, like the turbulent cascade of energy to shorter and shorter length scales, appears in the fluctuations of black branes. Eventually, the turbulence will cause the structure to appear on shorter wavelengths than the mean free path, so the fluid approximation breaks down and the full glory of GR must take over. (This is the same in water or whatever else: the molecular dynamics become important when the turbulence gets to a small enough scale, so you can no longer treat it as a fluid).

Answer 5

The obvious example of a chaotic solution to the Einstein equations is the Mixmaster metric. However this is not a vacuum solution, and when matter is present it shouldn't be any surprise that it can evolve in a chaotic fashion.

The more interesting question is whether a vacuum solution can evolve in a chaotic fashion. I can offer only a vague recollection from the 1980s when a friend of mine was working on the interactions between gravitational waves i.e. scattering of one GW by another when the energy is high enough that the linear approximation breaks down. My recollection is that bizarre behaviour could result, but whether this counts as chaos I don't know.

Answer 6

I'm posting an hypothesis on dark matter to work with, that I'll call the "Turbulence Dark Matter" (TDM).

The universe is filled with matter clumped into stars inside galaxies, and galaxies into clusters and superclusters. There's also gaz and dust everywhere. Their distributions are mostly random and include voids and swiss cheese-like "holes".

Suppose that spacetime is already "turbulent" at a not-so-large scale in space and in time. It could even have been created that way from a very violent Big Bang, and turbulent matter would just make the things worst after that. The exact metric of that spacetime ; $g_{\mu \nu}(x)$, is so complicated that there's no hope in solving the exact Einstein equation : $$\tag{1} G_{\mu \nu}(g) + \Lambda \, g_{\mu \nu} = -\, \kappa \; T_{\mu \nu}(\phi, \, g). $$ The symbol $\phi$ represents all the matter and radiation fields. We could write the exact (turbulent) metric components like this : $$\tag{2} g_{\mu \nu}(x) = \bar{g}_{\mu \nu}(x) + \theta_{\mu \nu}(x), $$ where $\bar{g}_{\mu \nu}(x)$ is a smooth and regular metric, while $\theta_{\mu \nu} \equiv g_{\mu \nu} - \bar{g}_{\mu \nu}$ describes the turbulences. The matter fields could also be written as $\phi = \bar{\phi} + \delta\phi$. Then, the equation (1) can be written like this : $$\tag{3} G_{\mu \nu}(\bar{g}) + \Lambda \, \bar{g}_{\mu \nu} = -\, \kappa \, \big( \, T_{\mu \nu}(\bar{\phi}, \, \bar{g}) + \Theta_{\mu \nu} \big), $$ where I have defined $$\tag{4} \Theta_{\mu \nu} = T_{\mu \nu}(\phi, \, g) - T_{\mu \nu}(\bar{\phi}, \, \bar{g}) + \frac{1}{\kappa} \big( G_{\mu \nu}(g) - G_{\mu \nu}(\bar{g}) \big) + \frac{\Lambda}{\kappa} \, \theta_{\mu \nu}. $$ This tensor could be explicitely developped to first order in $\theta_{\mu \nu}$ and $\delta\phi$. it could be interpreted as the stress-tensor of the "dark matter", induced by the neglected turbulences.

In this interpretation, dark matter is just an artifact of some averaging procedure, using a smooth and regular metric on a large scale (homogeneous and isotropic on cosmological scale) plus a perturbation. By its definition, this dark matter doesn't interact directly with normal matter, and cannot be detected in any lab ! The TDM doesn't really exist, and yet it is out there as an effective field.

---

EDIT : Take note that since $\Theta_{\mu \nu}$ depends on $\Lambda$ (the cosmological constant, which has nothing to do with the turbulences), it may explain the "coincidence" in the DM and DE proportions in the universe (around 25% and 71%, respectively, plus 4% of normal matter).

Also, if the universe was empty ; $T_{\mu \nu} = 0$, you still could have TDM if the spacetime curvature is very lumpy and chaotic, filled with random primordial gravitational waves : $\Theta_{\mu \nu} \ne 0$ even without normal matter.

Answer 7

I would just like to add a few things to the answers already presented.

If we accept that both general relativity and quantum mechanics are valid in their own rights (or close approximations to, once a quantum-gravity marriage occurs) then we can get turbulent type behaviours on very small scales. The uncertainity principle suggests that particle anti-particle annihilations may occur on Planck length scales, and the energy of the virtual particles increases as we go to smaller and smaller scales. As a result, GR tells us that the spacetime may behave very wildly at these Planck-type scales, and would indeed be turbulent in this sense. See Quantum Foam.

On a slightly different note, there are many still inherently-classical theories of gravity that go behind GR. Instead of using the Einstein-Hilbert action one can say the spacetime abides by some different geometric relations, i.e. not just $R_{\mu \nu} = 0$ (e.g. f(R) gravity). These are introduced to avoid the dark matter and dark energy problems, as well as some others. It turns out that so much richness comes from these theories that you can imagine choices of $f$ that allow for pecuilar metrics to arise as vacuum solutions. Indeed it can be shown that the Mixmaster solution is a vacuum-$f(R)$ solution for a judicious choice of $f$. So the turbulent matter-filled solution of GR is a vacuum solution in $f(R)$.

As for classical GR, I expect one can still concoct a vacuum solution in a less crazy sense by using the inverse scattering method to generate N-soliton solutions. If you dump enough non-linear solitions (solitary-waves) into the space (Here's a good starting place -- other papers are behind paywall) and have freedom to place them at any position I am sure you can get turbulence! Physical or not -- you decide.

Answer 8

Turbulence has many properties, like chaos, diffusivity, rotation, and dissipation. In particular, energy tends to flow from large-scale patterns to ever smaller size scales in a cascade.

Obviously relativistic fluids can be turbulent, so they can make a "turbulent spacetime" if they are heavy. But defining turbulence in empty space may be more complex, as discussed earlier here. General relativity can have chaotic solutions. Perhaps the best sign of "real" turbulence is an energy cascade, and this has been simulated for gravitational waves and modelled in other spacetimes. These energy cascades can also be entropy cascades.

So it seems that there definitely can be turbulent spacetimes, although they do not look like fluid turbulence.

Answer 9

Turbulence of a liquid requires parcels of the liquid to have linear and angular momentum different from neighbouring parcels of the liquid and a continuous energy input that drives and sustains the turbulence. Turbulence of a gravitational field can only be the result of turbulence in the masses creating the gravitational field. The vacuum outside a gravitational body cannot have turbulence in its own right, where there is no turbulence inside the gravitational body. To endow the vacuum with turbulence in its own right, is to imply that the vacuum has momentum in its own right, which would strongly imply ether like properties for the vacuum. Do we want that?

Answer 10

Much of this discussion centers around whether a vacuum solution can be "turbulent". Such turbulence may be entering the realm of Quantum Gravity and as such both EFE and NS cannot be applied. And now, the Perimeter Institute makes a strong case for Turbulence.. https://www.perimeterinstitute.ca/news/turbulent-black-holes

Answer 11

As follows from the relationship between the Navier – Stokes equation and the Schrödinger equation, the vacuum has a kinematic viscosity ih/(2m) and low density. It is not empty space, but is the medium providing this kinematic viscosity. How to introduce Planck's constant into the GR equation is a separate conversation.

Answer 12

The turbulent solution is complex, where the real part is the average value, and the imaginary part is the rms value. Solving the non-linear Navier-Stokes equation is necessary in the complex plane. Similarly, the decision of GR should be complex in the case of a turbulent regime. But there is a problem how to recalculate the imaginary part of the solution in the real part, for this you need to use special methods. Moreover, in the case of a liquid medium, the roughness must be taken into account.

Answer 13

Turbulent spacetime from Einstein equation?

Hmmmn. Strictly speaking spacetime is an abstract mathematical "space" which models space at all time. It's the block universe. You can draw worldlines in it to represent motion through space over time, but there's nothing moving through it or in it. It's static. The wordlines aren't waving around like seaweed in the surf. However space can change over time. Gas clouds collapse to form stars and gravitational fields can become more pronounced. Since a gravitational field is "curved spacetime" we can reasonably say that spacetime changes. Let's call it space-time to distinguish it from the static block-universe spacetime. But can this change be a "turbulent" change? Hmmmn.

It is well known that the fluid equations (Euler equation, Navier-Stokes, ...), being non-linear, may have highly turbulent solutions. Of course, these solutions are non-analytical. The laminar flows solutions (Couette flow for example) may be unstable to perturbations, depending on viscosity. Also, fluids which have a low viscosity (water for example) are more turbulent than fluids with high viscosity (oil, for example).

No problem there. Apart from the fact that space-time isn't a fluid.

I was wondering if something similar may happen with gravity and spacetime itself. Einstein equation being highly non-linear, do turbulent solutions exist ?

No. Because space-time isn't a fluid. Instead it's a gin-clear ghostly elastic solid! This is why you can see a shear stress term in Einstein's stress-energy-momentum tensor:

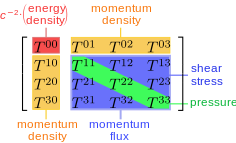_{Public domain image by Maschen, based on an image by created by Bamse see Wikipedia}

I kid ye not! Google on Einstein elastic. Then try to imagine a jelly on a plate. You can deform it, and you can curve it, and you can wibble it and you can wobble it. But you can't make it turbulent.

Or is gravity like some highly viscous fluid, i.e. without any turbulence ?

A gravitational field is where space is non uniform, this being modelled as curved space-time. Ah, here we go, this is what Einstein said:

"According to this theory the metrical qualities of the continuum of space-time differ in the environment of different points of space-time, and are partly conditioned by the matter existing outside of the territory under consideration. This space-time variability of the reciprocal relations of the standards of space and time, or, perhaps, the recognition of the fact that 'empty space' in its physical relation is neither homogeneous nor isotropic, compelling us to describe its state by ten functions (the gravitation potentials g$_{\mu\nu}$), has, I think, finally disposed of the view that space is physically empty."

While being non-analytical, how a turbulent metric may look like? I imagine that spacetime turbulences may be relevant on a very large scale only (cosmological scales, or even at the Multiverse level). And maybe at the Planck scale too (quantum foam). But how could we define geometric turbulence ?

I think it's reasonable to propose a chaotic metric, but turbulent doesn't seem to fit in with General Relativity. Which as I'm sure you're aware, is one of the best-tested theories we've got. Meanwhile multiverses and quantum foam remain speculative. IMHO it's good to speculate and ask what if? It's good to think for yourself. But I'd say you're in danger of wandering away from hard science here into pseudoscience, where you won't find any evidence or answers.

The only reference I've found on this subject, which shows that the idea isn't crazy, is this : https://www.perimeterinstitute.ca/news/turbulent-black-holes

The mention of the holographic conjecture does not auger well. And I'm afraid the fact that this comes from the Perimeter Institute doesn't mean it's correct. What we seem to have here is a rather speculative idea that looks as if it's at odds with general relativity. Ah, see the paper on the arXiv : Turbulent black holes. I've skimmed it, and I wonder if there could be some important issues. For example, the "coordinate" speed of light at the event horizon is zero. So if the black hole is spinning at half the speed of light, how fast is it spinning? What's half of zero? Anyway, it's late, and I have to go. You could try a new question asking for feedback on this paper. It's always better to refer to the actual paper rather than the reportage, because the latter can sometimes be misleading.