Anderson localization (AL) is, indeed, just a wave interference phenomenon, and can exist in completely classical systems. For example, it has been seen in ultrasound waves. The fact that it was first studied in the context of electrons is more of a historical accident than anything else, as far as I can tell. The required coherence and isolation of the system, as well as the strength of scattering normally required, just turn out to make it hard to see classically unless you are really looking for it.
Even though it is classical, the easiest way to understand AL is in a path-integral picture, so I will discuss it in those terms. Consider a particle in a system of fixed scatterers, like so:
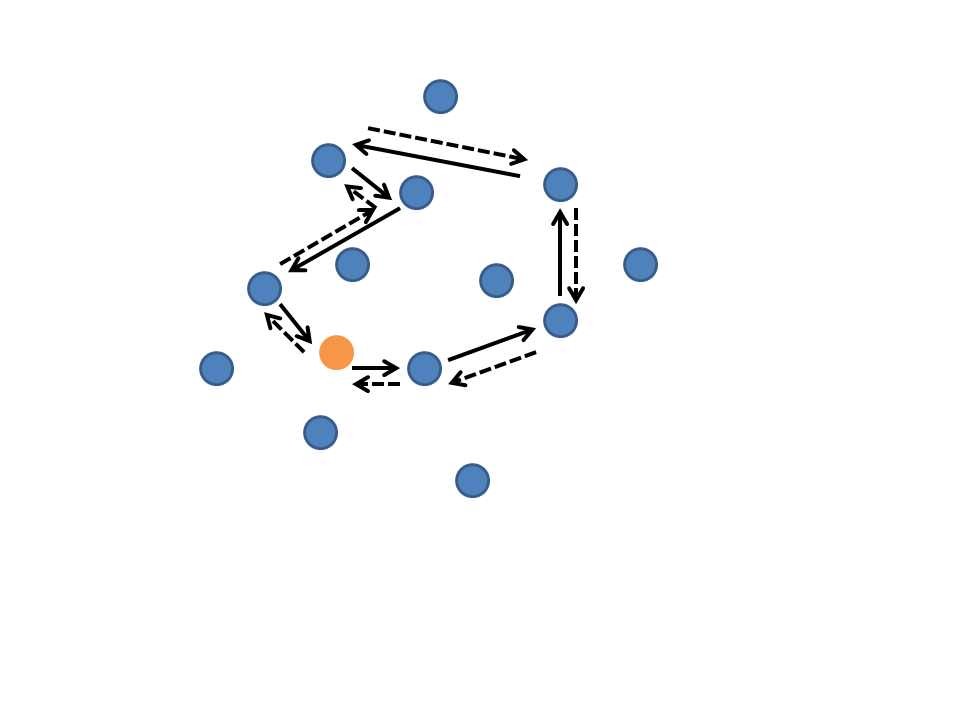
Orange is our particle, and blue are the fixed scatterers. The solid arrows denote one path through the system that returns to the original location, which in a path integral formalism should have some amplitude $\phi$. However, for every path that returns to the origin like this there is also a time-reversed path, shown in this case by the dashed arrows. Notice that such time-reversed pairs only exist for paths returning to the original point.
Now, in a path integral you would sum up all the amplitudes of the paths going anywhere, and see where they interfere constructively or destructively, and that determines where the particle is likely to go. But these time-reversed pairs will always have the same amplitude, so they will always add constructively with each other. Crudely, for a round-trip path $i$,
$P(return)_i=|\phi_{i,forward}+\phi_{i,backward}|^2=4|\phi|^2$.
This means the particle will always have a higher probability of staying at its initial location than classically expected. This isn't the same as saying it is fully localized- it could only result in weak localization- but under some circumstances this increased probability to stay near the origin can win out over the probability for it to wander indefinitely away.
This isn't enough for a quantitative analysis of AL, but it will get you a long ways. For example, it is clear from this example that localization will only occur when the two time-reversed paths have the same total phase. This implies that if inelastic collisions, which effectively scramble the particle's phase, are common enough, they will ruin localization. Specifically, inelastic collisions set a phase coherence length, $l_{\phi}$, and if this length is short enough such that a particle's phase is scrambled over a typical return path, localization will not occur. Something that breaks time-reversal invariance like a magnetic impurity will also ruin localization.
Finally, as this picture suggests, it is useful to compare Anderson localization to a (classical) random walk. Anderson localization can be thought of as a quantum random walk, which has the important features of interference between paths but is fundamentally similar. In unbiased classical random walks, it is well-known that the probability of return depends crucially on the spatial dimension of the problem. In one or two dimensions, the walker will return to its origin an infinite number of times as the number of steps goes to infinity, but in three or more dimensions this is no longer true. A similar dependence on dimensionality occurs in Anderson localization: a particle in a disordered one- or two-dimensional system turns out to always be localized (assuming perfect coherence and time-reversal symmetry), but in three or more dimensions only particles below a critical energy are localized. This is known as the mobility edge, and it is an important problem in AL to figure out exactly where it is for a given system.

