3
$\begingroup$

A Day and Night World Map shows which parts of the Earth are in daylight and which are in night at a given instant. At one side of the Daytime line they are in daylight and at the other side they are in twilight.

The shape of the day and night areas are different at the different days of the year.

For example, on UTC time = Wednesday, 23 September 2015, 08:20:00; during the September Equinox it was like: September 2015 Equinox

While on UTC time = Sunday, 21 June 2015, 16:38:00; during June Solstice it was like: June 2015 Solstice

And on UTC time = Friday, 20 March 2015, 22:46:00; during March Equinox it was like: March 2015 Equinox

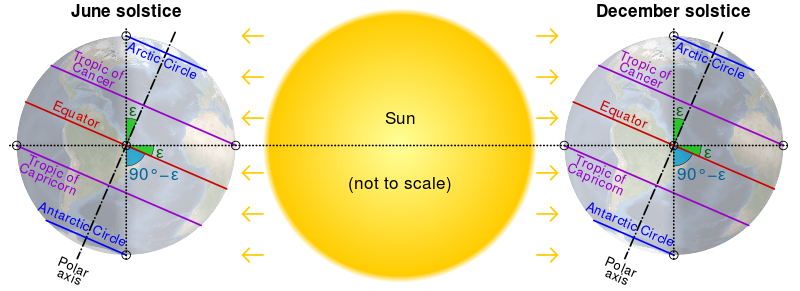
I believe that this has to do with the Earth's axial tilt with respect to the Ecliptic plane. Axial tilt.

What I would like to know is:

How is the calculation of the shape of the areas in a Day and Night World Map done?

What is the curve that describes the Daytime line in a Day and Night World Map?

I suspect it has to do with the projection of a great circle on an Equirectangular projection of the world map... but I would like to know if this is correct or not.

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ Perhaps this helps? edesign.nl/2009/05/14/math-behind-a-world-sunlight-map Or even: gamedev.stackexchange.com/q/30840 $\endgroup$
    – Řídící
    Commented Oct 26, 2015 at 17:56
  • $\begingroup$ In amateur radio this is called the "grey line", although I don't know if that term is used elsewhere... $\endgroup$
    – bitsmack
    Commented Oct 26, 2015 at 18:22
  • $\begingroup$ "I suspect it has to do with the projection of a great circle on an Equirectangular projection of the world map": yes, you are correct $\endgroup$
    – user83548
    Commented Oct 26, 2015 at 20:30

1 Answer 1

Reset to default
2
$\begingroup$

Absent a small amount of distortion from the oblateness of the Earth, yes: it is a great circle. To generate the corresponding curve on a flat map, you have to use the map's projection rules to project the coordinates of the circle's circumference onto the map. That completely depends on the projection rules for the specific map you're using.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Great! Thanks for your answer! Then what would be the projection rules to project the coordinates of the circle's circumference onto the map according to the Equirectangular projection? $\endgroup$
    – roy
    Commented Oct 27, 2015 at 17:39
  • $\begingroup$ Iterate through the latitude and longitude of the points of the circumference of the circle, and then convert that to points on the map. $\endgroup$
    – Daniel Griscom
    Commented Oct 27, 2015 at 18:16
  • $\begingroup$ I just found this answer related to this question in the Geographic Information Systems StackExchange. gis.stackexchange.com/questions/52949/… $\endgroup$
    – roy
    Commented Jan 19, 2016 at 15:04

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.