3
$\begingroup$

So thin film interference is when light is half-reflected half-refracted then the reflected and refracted wave interfere with each other to produce another color.

What I don't understand is that the waves are off-sync; not on top of each other. So why do they interfere? And doesn't interference change only amplitude? Why does it affect the wavelength?

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ Who says it affects the wavelength? $\endgroup$
    – Emilio Pisanty
    Commented Oct 8, 2015 at 12:29
  • 1
    $\begingroup$ What you describe as thin-film interference is, at best, confused. Generally, it's incorrect. Are you talking about monochromatic or non-monochromatic light? If monochromatic, there is no color "change" only intensity changes. Go back and re-read your source. $\endgroup$
    – Bill N
    Commented Oct 8, 2015 at 15:49

2 Answers 2

Reset to default
3
$\begingroup$

Everything is explained in wikipedia at "thin film interference". What do you mean by "off-sync" ? In classical images like the wikipedia one (see below) only one ray is drawn, but in practice there are an infinity of parallele rays, so superimposition do occurs. But if by "off-sync" you mean there is a phase difference in the "2" superimposed rays, this is the whole point: phases will positively or negatively (or intermediatly) interfere based on this phase difference. And since the phase corresponds to wavelength/offset, the phase difference varies with wavelength.

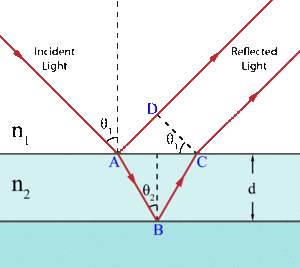
enter image description here

Improve this answer
$\endgroup$
2
$\begingroup$

All the rays are reflected at some point. One is reflected immediately and never enters the thin film. There, it is reflected at an angle $\alpha$, equal to the angle of incidence. Another ray might enter the thin film, being refracted to an angle $\beta$, is then reflected on the inside of the film, and then leaves again on the same side it entered, thereby again being refracted, so it too leaves at an angle $\alpha$. Any other rays might be reflected any (odd) number of times on the inside of the film (each time at an angle $\beta$), but in the end they all leave at the angle $\alpha$ they entered at. Thus, all those rays are indeed "on top of each other" in terms of angle (wave vector), but their relative "optical path lengths" differ depending on how often they have been reflected on the inside.

The color one sees depends on the thickness of the film. The two phase boundaries act as a resonance cavity. This effect is used in so-called Fabry-Perot interferometers. It is not that the color of the light changes, but rather light of certain wave lengths is enhanced by the interference (constructive, large amplitude) whilst all the other wave lengths interfere destructively (small amplitude). Thus, if you shine white light on such a film, you will only see the colors which resonate with the films thickness. The variety of color in, say, a soap bubble is due to variations in the thickness of the film.

Improve this answer
$\endgroup$
4
  • $\begingroup$ So the colors we don't see are destroyed by interference and the colors we do see are amplified by interference? Does that mean amplitude plays a role in which color we see? $\endgroup$
    – Loogai
    Commented Oct 9, 2015 at 8:18
  • $\begingroup$ Well, the color is determined by the wave length. But we don't usually deal with monochromatic light, but with a mixture of different wave length. The relative amplitude of the wave lengths determines our perception of the color of the whole thing. $\endgroup$
    – Jonas Greitemann
    Commented Oct 9, 2015 at 9:10
  • $\begingroup$ It's just the same with sound. You usually won't listen to a single wave length (pitch) but to a whole song composed of various wave length. When you crank up the bass on your stereo, you apply a filter which will enhance the long-wave length (low-pitch) amplitudes and deminish the small-wave length (high-pitch) amplitudes, changing your overall impression of the song. $\endgroup$
    – Jonas Greitemann
    Commented Oct 9, 2015 at 9:14
  • $\begingroup$ The thin film acts similarly, but for light. The more internal reflections you get in the film, the more selective is the film about what wave lengths it enhances and which one's it doesn't like. $\endgroup$
    – Jonas Greitemann
    Commented Oct 9, 2015 at 9:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.