How do you derive Fleming's left hand rule? What is the theoretical explanation for the directions of the magnetic field, current and the force on the current for being oriented in that way relative to one another?
$\begingroup$
$\endgroup$
9
-
1$\begingroup$ This is an experimental result, not a theoretical one. (Furthermore, it depends on the sign convention used for the field and the current). Theoretically these results are captured in the expression for the Lorentz force, specifically $\vec F = q \vec v \times \vec B$. $\endgroup$– Sebastian RieseCommented Sep 13, 2015 at 15:07
-
$\begingroup$ There is an explanation based on intrinsic spin of a particle and the related magnetic dipole moment. $\endgroup$– HolgerFiedlerCommented Sep 14, 2015 at 10:58
-
1$\begingroup$ @HolgerFiedler interesting similarity/analogy with angular momentum of a (macro size) spinning body, precession of its spin access and gyroscopic torque, all which also form an orthogonal system. $\endgroup$– docscienceCommented Sep 21, 2015 at 19:54
-
1$\begingroup$ A deeper question than right/left hand, and what HolgerFielder has commented, is Why an orthogonal arrangement of forces? $\endgroup$– docscienceCommented Sep 21, 2015 at 19:57
-
$\begingroup$ @docscience Due to my poor English it is possible for you to improve the paper? $\endgroup$– HolgerFiedlerCommented Sep 22, 2015 at 5:50
|
Show 4 more comments
2 Answers
$\begingroup$
$\endgroup$
2
The fact that Fleming's is a left-hand rule is an artifact of the completely arbitrary choice of the right-hand rule to define the direction of the magnetic field. If electromagnetic induction had been discovered by people who put South at the tops of their maps, we might well define the direction of a cross product using the left hand instead of the right. Since every prediction of an acceleration in electrodynamics involves an even number of right-hand rules, and complete and consistent switch to the left hand would be mathematically identical.
That said, Fleming's rule is a consequence of the Lorentz force between the fields and currents in the motor (or generator).
-
$\begingroup$ "Fleming's Rule is a consequence of the Lorentz force..."? Isn't that the wrong way round, as Sebastian Riese points out? The Lorentz force is modelled on (and therefore the consequence of) the observation within Fleming's Rule. $\endgroup$ Commented Jul 9, 2016 at 3:24
-
$\begingroup$ @sammygerbil I hadn't thought about the chronology; of course Fleming's observations came first and Lorentz's model is consistent with them. But there is still an arbitrary choice involved. The direction of a magnetic field --- a pseudovector quantity in a parity-conserving theory --- is not an observable. $\endgroup$– rob ♦Commented Jul 9, 2016 at 12:51
$\begingroup$
$\endgroup$
Let us consider the following experiment:
We place a stiff copper wire on a table. Parts of its length don’t touch the table. Above a wire section that does not touch the table we hold a strong cylindrical magnet with its plus-pole down, so that the wire lies exactly under the middle of the magnet (I have used a cylindrical neodymium magnet with 3cm diameter) (to understand which pole of the magnet I call “plus”, please read this answer https://www.quora.com/Is-positive-and-negative-electricity-nomenclature-arbitrary/answer/Mitko-Gorgiev. Then we connect a new battery to the ends of the wire so that the plus-pole of the battery is closer to us and the minus-pole further away from us. At the moment of connection we will notice that the wire makes a strong deflection to the left and up. As soon as we turn the magnet over and repeat the same, the wire will make a strong deflection to the right and up. If we hold the magnet again with the plus-pole down, now not directly over the wire, but left over it, however still close to it, we will notice that the wire after connecting to the battery makes a jerky movement to the right and down. How is this explained?
In the first variant, the permanent magnet “blows” down; the magnetic wind in the wire blows clockwise spirally from the plus- to the minus-pole of the battery (the wind in the wire spreads also around the wire); it blows down on the right side of the wire, up on the left side of it; on the right of the wire both magnetic winds coincide (the effect intensifies), and on the left of the wire they collide (the effect weakens); the wire moves to where the effect only intensifies, namely to the maximum, and that is to the left and up. In the third variant, in which both winds only collide, the wire deflects to where the adverse effect is maximally attenuated or quite ceased, namely to the right and down.
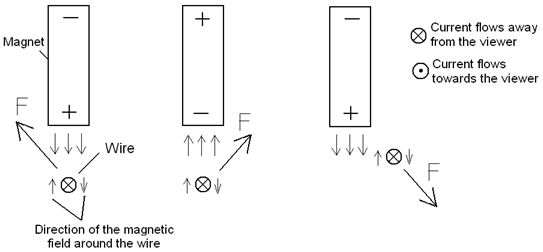Fleming’s rule is actually not true, because the current carrying wire does not deflect perpendicularly to the external magnetic field, but obliquely.
So, from now on you don’t have to remember Fleming’s rules and sprain your fingers. All you need to remember is that the magnetic wind through the wire blows clockwise from the plus- to the minus-pole of the battery and then to engage your logic.
More about this you can read in my book https://newtheories.info , pages 32–34.
