Path length difference from multiple sources?

Question

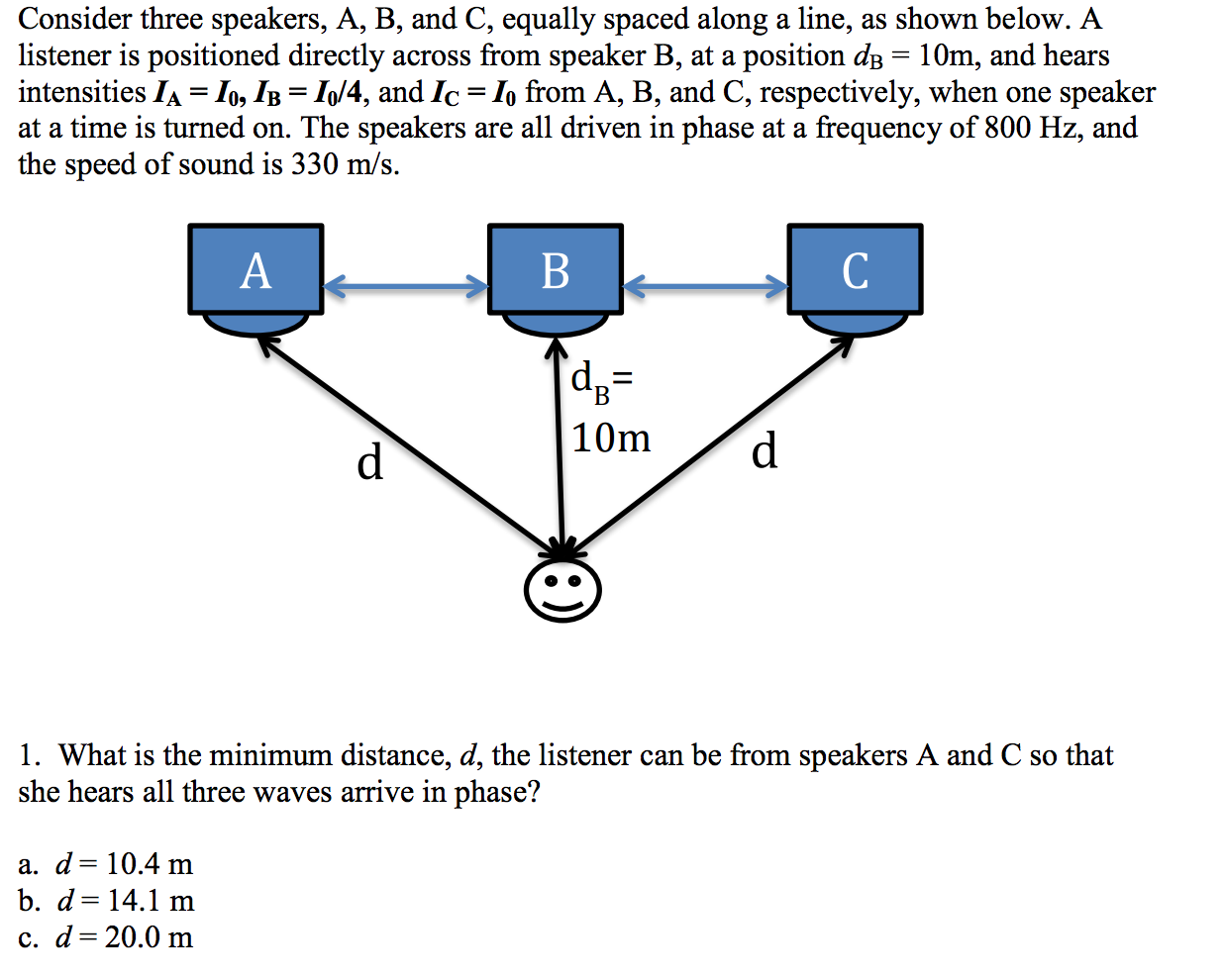
I know that path length difference is given by the difference in the distance from each source to the observer, but I feel like this doesn't apply when some of the distances are the same, like in the following question:

What would the path length difference here be? I know that `path length difference is a function of $d = m\lambda$ where $\lambda$ is wavelength. I know the wavelength is $0.4125$ $meters$ , and I'm pretty sure $m$ is $1$ so that all three waves constructively interfere. But simply subtracting the differences in each of the path lengths would yield $10$, so what am I missing?

(The answer is $d = 10.4$ $meters$ by the way, just don't know how to get that)

Answer 1

When you wrote that "path difference" d=m(wavelength), then this is not the 'd' which question is asking.

And now from your calculations ∆d=0.4125, so the distance 'd' is ∆d=(d-10) ( for sources A and B) => d=10.4125 is your answer!

Answer 2

You are pulling out an equation with an m for no reason.

Step 1 you need to understand the question. So consider a simpler problem. What if the speed of sound was 1m/s and the devices had a period 1s (they could be playing the same recording that repeats every second). What if you were 1m from the center one. And then you placed the other two speakers $\sqrt 3$m away from the center one.

Now when the center one starts playing you have to wait a second to hear it (one meter away and the speed of sound is 1m/s). And after a second it repeats itself (period is 1 second). Now what about the other two? How far away are they. When do you hear the sound get to you? Are the sounds lined up so you hear the same sounds at the same time?

How about if the other speakers were $\sqrt 8$m away from the center one? How about if the other speakers were $\sqrt 15$m, $\sqrt 24$m, $\sqrt 35$m, or $\sqrt 48$m away from the center one?

The same ideas apply, but with different numbers and different things you are looking for. You want to hear the same thing from each speaker. The speakers repeat themselves every so often. The speakers are a certain distance away and sound travels at a certain speed.