1
$\begingroup$

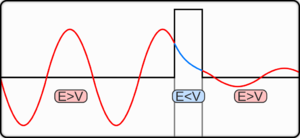
I'm assuming the 'amplitude' is kind of like the MeV it has on it, so could be seen as a product of the voltage applied to that electron in a field.

But how can it 'lose' volts when passing the barrier, but still have the same energy as a whole? Does it increase the frequency in proportion to the voltage decrease?

enter image description here

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ The amplitude of the wave function is just what gives you the probability of to find the electron at that position. I don't understand what you want to know. $\endgroup$
    – ACuriousMind ♦
    Commented May 2, 2015 at 19:41
  • 1
    $\begingroup$ The amplitude is not "kind of like MeV"... It is the probability amplitude. physics.stackexchange.com/questions/57595/… ..Or just google for it. $\endgroup$
    – Hasan
    Commented May 2, 2015 at 19:43
  • $\begingroup$ The probability of it tunneling is proportional to the energy of the tunneling electron in relation to the barrier. So why does the amplitude decrease, and yet energy remains constant? $\endgroup$
    – ARMATAV
    Commented May 2, 2015 at 19:45
  • 1
    $\begingroup$ The probability of tunneling is a different issue. The amplitude here refers to the probability of finding the electron, no matter what its energy, at a certain point. Low amplitude means that it's less likely to find the electron in that region. This decreased likeliness to find the electron is not related to the energy of the electron. $\endgroup$
    – Hasan
    Commented May 2, 2015 at 19:51

1 Answer 1

Reset to default
1
$\begingroup$

If you have a flux of electrons, the transmitted power is proportional to the number of tunneling electrons. The other electrons are reflected from the barrier. Thus, the barrier just redistributes the incident electron flux into two ones. It does not change the individual electron energy.

Improve this answer
$\endgroup$
3
  • $\begingroup$ So you must decrease the barrier or introduce more electrons to tunnel at a higher probability; increasing the individual levels of electrons does nothing? So a 10,000,000 volt field with a single electron in it has the same probability to permeate a barrier as a 1 volt field with a single electron? $\endgroup$
    – ARMATAV
    Commented May 2, 2015 at 20:29
  • $\begingroup$ According to this page hyperphysics.phy-astr.gsu.edu/hbase/quantum/barr.html the E level dictates whether the wave surmounts the barrier, and has an easier time crossing it. So I don't understand why the individual energy has no effect. $\endgroup$
    – ARMATAV
    Commented May 2, 2015 at 20:34
  • $\begingroup$ Decreasing the barrier increases the probability and does not change the electron energy. Introducing more electrons (by increasing the contact surface, for example) increases the electron flux, not the probability. Flux is a product of the number of tunneling electrons per second, their charge, etc. The probability depends on energy, but the energy after tunneling is the same as before. Presence of the barrier does not subtract the electron energy. $\endgroup$
    – Vladimir Kalitvianski
    Commented May 2, 2015 at 20:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.