Condition for formation of interference pattern

Question

I recently came across this in a textbook (NCERT class 12 , chapter: wave optics , pg:367 , example 10.4(d)) of mine while studying the Young's double slit experiment. It says a condition for the formation of interference pattern is

$$\frac{s}{S} < \frac{\lambda}{d}$$

Where $s$ is the size of source, $S$ is its distance from the plane of the 2 slits, $\lambda$ is the wavelength of light and $d$ is the separation between the 2 slits.

There is no justification given and I am wondering how this is so. Any help would be thoroughly appreciated.

Answer 1

The source is large, spatially incoherent. Each point $P$ of the source creates its own fringe system. For a non-centered point of the source, identified by ${{x}_{P}}$ the additional path is ${{\delta }_{P}}=\frac{{{x}_{P}}D}{S}$

The fringes remain visible if ${{\delta }_{P}}\ll \frac{\lambda }{2}$

At worst, for points at the edge of the source ${{x}_{P}}=\frac{s}{2}$ , which gives $\frac{s}{2}\frac{D}{S}\ll \frac{\lambda }{2}$ or $\frac{s}{S}\ll \frac{\lambda }{D}$

This criterion is simple $\frac{s}{S}$ because is the angle under which is seen the source if one is placed at the level of the two holes.

Sorry for my poor english !

Answer 2

Here is an elementary proof of your equality.

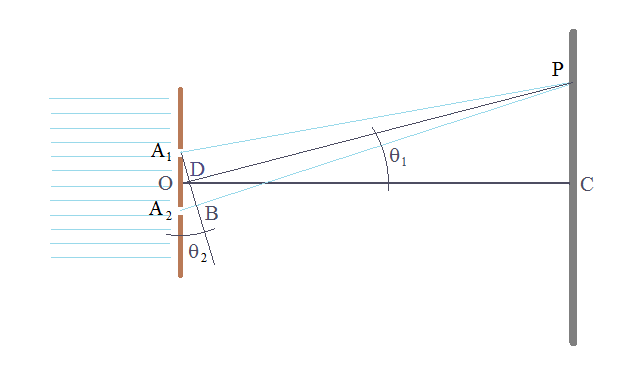
In the picture below,you see that the segment $A_2 P$ is the path-length difference between the ray reaching the point $P$ from the slit $A_2$ and the ray from the slit $A_1$ . I the triangle $A_1 PB$ the edges $PA_1$ and $PB$ are equal, and if the angle $A_1 PB$ is small, the line OP can be considered perpendicular on the line $A_1 B$. That means, since the segment $OA_1$ is also perpendicular to $OC$, that the angles $θ_1$ and $θ_2$ are equal. So, the triangles $OA_1 D$ and $A_1 PD$ are equivalent, and we have the relation $\dfrac {PC}{OD} = \dfrac{PD}{OA_1}$. Translating to your symbols, $$ \frac{1}{2} \dfrac{s}{OD} \approx 2\dfrac S d \tag{1} \, .$$ (When equating PD with S, I neglected OD in comparison with PD.) I wrote $\dfrac s2$ because you need the distance between two maxima, and PC is only half of this. Now, in order to have a maximum of intensity at the point P, the distance $A_2 B$ has to be an integer of $\dfrac \lambda 2$. Notice that, again for small angles $\theta$, $A_2 B \approx 2 \times OD$

Introducing in (1) we get $$ \dfrac{s}{A_2 B} \approx \dfrac{2S}{d} \tag{2} \, ,$$

which implies your equality $$ \dfrac s \lambda \approx \dfrac S d \, .$$

Answer 3

Source S of width s produces diffraction pattern, see fig.

Where α1 corresponds to first minima and given by

                 α1=λ/s

Two slits s1 and s2 are to be placed within first minima on either side of central maxima. See fig below.

Form fig for small angles α2 is given by

α2=d/S

where d separation between s1 and s2, S is separation between source and slits s1 ,s2

it is clear that α1>α2.

                        λ/s>d/S

                        λ/d>s/S