Heat loss using alternating current

Question

I am looking for somebody who can explain this to me. As I have read in physics books, the Joule-Lenz Law (Joule effect of heating) is represented by the formula:

$$ W = I^2Rt $$

which can be transformed into:

$$ W = VIt $$

(using V = IR). The when I have reached the lessons about alternating current, it was said that this formula can be used also for problems connected with it but it was said that in order to minimize the heat loss, the alternating current that is produced by the power stations is transformed into alternating current with high voltage. If we see the formula $W = I^2Rt$, that makes sense but as soon as we substitute with $V = IR$ and get $W = VIt$ this sounds strange (we already know that power maintains same when we use transformers). So where do I make a mistake and how does it work?

P.S. Why does all the energy of electricity is transformed into heat?

Answer 1

The confusion is because the $V$ in:

$$ W = IVt $$

is the voltage drop across the transmission line not the supply voltage.

If the power station generates some high voltage $V_0$, then at your local transformer the voltage will have fallen slightly to $V_1 = V_0 - \Delta V$ due to the resistance of the transmission lines. The voltage drop is:

$$ \Delta V = IR_{transmission} $$

where $R_{transmission}$ is the resistance of the transmission line. The power loss in the tranmission line is:

$$ W_{transmission} = I\Delta V t = I^2 R_{transmission} t $$

Response to comments:

I think it's worth working out what happens quantitatively. For simplicity consider the load to be just a single house and assume there's a single transformer to reduce the transmission voltage to domestic voltage.

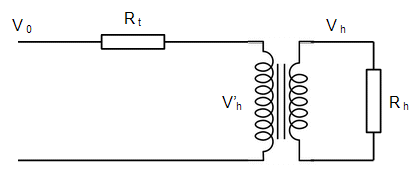
$V_0$ is the transmission voltage, e.g. 400kV in the UK, and $V_h$ is the voltage at the house, e.g. 240V in the UK. So the transformer separating the house from the grid has a ratio of $V_0/V_h$. I've represented the resistance of the transmission line as a single resistor, $R_t$, and we want to calculate how much power is lost in the transmission line.

Suppose the power being used by the house is $W$ - I would guess typical values for W would be a few kW - then:

$$ W = I_hV_h $$

or:

$$ I_h = \frac{W}{V_h} \tag{1} $$

For convenience we'll assume that the voltage drop across the transmission line is small so the voltage at the line side of the transformer, $V'h$, is just $V_0$. The power on both sides of the transformer is the same, so:

$$ V_0 I_0 = V_h I_h $$

Substituting for $I_h$ from equation (1) and rearranging we get:

$$ I_0 = \frac{V_h}{V_0} \frac{W}{V_h} = \frac{W}{V_0} $$

And the last step is to calculate the power dissipated in the transmission line using:

$$ W_t = I_0^2 R_t = \left(\frac{W}{V_0}\right)^2 R_t $$

The key result is that the power dissipated in the transmission line is inversely proportional to $V_0^2$. So increasing the transmission voltage $V_0$ reduces the power wasted.

Answer 2

First of all you should note that we use RMS value of $ I $ and $ V $ .

Now imagine that we have a transmission line with resistance of $ R $, now if we increase the potential difference ($ \Delta V $) across it or the current that goes through it, in both cases we get more heat loss.

But in actual transmission lines we do not increase the potential across the line. we decrease voltage across the line. How?
with the help of transformers we decrease the current through the line(right?), then if we use $ \Delta V = IR $ (across the line), you see that the voltage difference is decreasing across the line.

To show it with formulas, with or without transformers :

$ \Delta V^2 t/R = R I^2 t $    so both formulas are equal.

So the point is that $ \Delta V$ is decreased(not $V$) because $ I $ has been decreased.