For the jumping ring experiment (I think it is also called Thomson ring experiment) why does the ring float and not move up and down the iron core as would be expected?
I think by Lenz's law, since when the current through the coil is decreased (in a negative cycle of AC) , should the ring not fall back down to increase the magnetic flux (as near the coil the field is stronger) through it to compensate for the lowering due to the smaller current? So why does it not oscillate significantly?
The first thing to realize is that in order to have a vertical force acting on the ring, the magnetic field must have a radial component. Indeed, Laplace formula gives us:
$$ \overrightarrow{dF} = i_{ring}~ \overrightarrow{dl} \times \overrightarrow{B}$$
Expressing all the fields in cylindrical coordinates $\big( \overrightarrow{e_{r}}, \overrightarrow{e_{ \theta }},\overrightarrow{e_{z}}\big)$, one gets:
$$ \overrightarrow{dF} = i_{ring}~ \big(dl ~\overrightarrow{e_{ \theta }} \big) \times \big(B_{r} \overrightarrow{e_{r}} +B_{ \theta } \overrightarrow{e_{ \theta }}+B_{z} \overrightarrow{e_{z}}\big) $$
If the ring has a diameter "r", one finds:
$$ \overrightarrow{F} = 2 \pi r~ i_{ring}~\big(B_{z} \overrightarrow{e_{r}}-B_{r} \overrightarrow{e_{z} } \big)$$
The vertical z component of the electromagnetic force acting on the ring is thus:
$$ F_{z}=-2 \pi~ r ~i_{ring} ~B_{r} $$
In practice, having a magnetic field with a radial component means the magnetic field lines mushroom out:
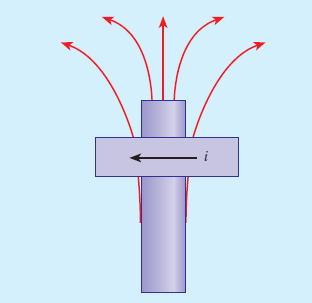
If we were to try the experiment with a continuous iron core, we would not observe any levitation effect.
Let's go back to the formula for the vertical force and identify the variables. Both the induced current $ i_{ring}$ and the radial magnetic field $ B_{r}$ depend on both the time t and on the vertical distance from the coil z.
$$ F_{z}(z,t)=-2 \pi~ r ~i_{ring}(z,t) ~B_{r}(z,t) $$
The above formula is sufficient to understand why there is a net vertical force acting on the ring. When the external magnetic field $B_{r}(z,t) $ changes direction, the induced current in the ring also changes direction but it is lagging relative to $B_{r}(z,t) $ by a factor $\Psi$. This phase difference is at the origin of the force.
Because of the iron core, there is no simple formula for magnetic field created by the coil. However, since the coil is fed with an AC current, we can precise the time dependence.
$$ \overrightarrow{B}(z,t)= \big(B_{r}(z) \overrightarrow{e_{r}} +B_{ \theta }(z) \overrightarrow{e_{ \theta }}+B_{z}(z) \overrightarrow{e_{z}}\big)~ sin( \omega t) $$
This magnetic field induces an e.m.f e into the ring, with:
$$e=- \frac{d \Phi }{dt}=- \pi r^{2} B_{z}(z) ~ \omega ~cos( \omega t)$$
The ring behaves like a (L,r) circuit. It is characterized by its impedance Z and phase shift $ \Psi$. With:
$$Z= \sqrt{r^{2}+ \omega ^{2}L^{2} }~~~~~~~~tan( \Psi )= \frac{ \omega ~L}{r} $$
This e.m.f drives the current $i_{ring}(z,t)$, with:
$$i_{ring}(z,t)=- \frac{\pi r^{2} B_{z}(z) ~ \omega}{Z} ~cos( \omega t- \Psi )$$
Plugin together all our results one finds for the vertical force acting of the ring:
$$ F_{z}(z,t)=2 ~\frac{\pi^{3} r^{3} B_{z}(z)~B_{r}(z) ~ \omega}{\sqrt{r^{2}+ \omega ^{2}L^{2} }} ~cos( \omega t- \Psi ) ~sin( \omega t) $$
It's time average (remember with AC current, one often time average):
$$\langle F(z)\rangle = \frac{ \omega }{2 \pi } \int_0^ \frac{2 \pi }{ \omega } F_{z}(z,t) dt=\frac{\pi^{3} r^{3} B_{z}(z)~B_{r}(z) ~ \omega}{\sqrt{r^{2}+ \omega ^{2}L^{2} }} ~sin( \Psi )$$
Note that for a non inductive ring, $\Psi=0$, and the force is null.
Using trigonometric transformation, we can calculate $sin(\Psi )$. It comes:
$$sin(\Psi )= \frac{L \omega }{Z}= \frac{L \omega }{\sqrt{r^{2}+ \omega ^{2}L^{2} }} $$
And we finally get for the average vertical force:
$$\langle F(z)\rangle = \frac{\pi^{3} r^{3} L~ \omega^{2}~ B_{z}(z)~B_{r}(z) }{r^{2}+ \omega ^{2}L^{2}}$$
That being said, the instantaneous force oscillates at $2 ~\omega$.
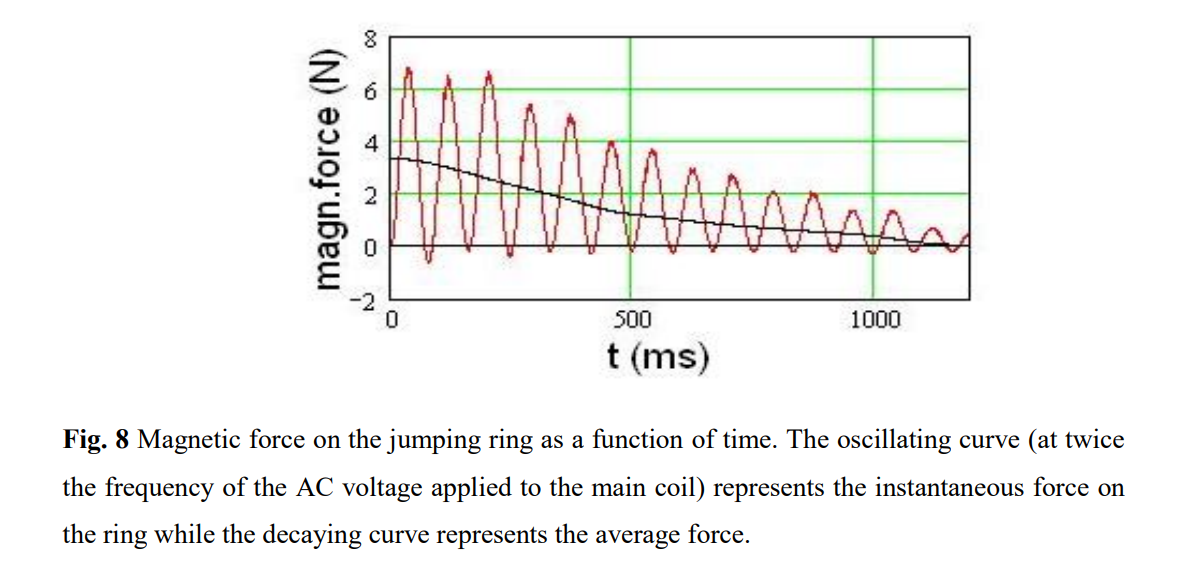
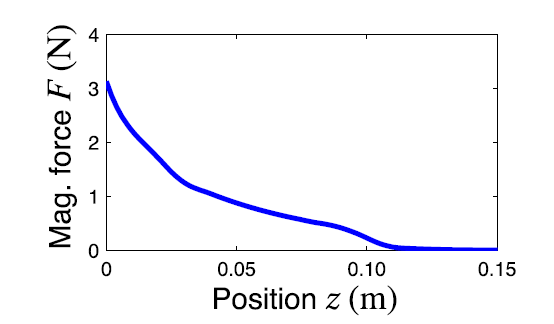
You can read: Unveiling the physics of the Thomson jumping ring for a more detailed description. I took the graph from this paper. This second paper is somewhat more readable: The jumping ring and Lenz's law—an analysis
Your intuition is correct. The ring develops a current, and thus a magnetic field only when the external magnetic field changes. A steady DC current would behave like a regular magnet: the ring would not move, and in fact resist motion (since that would entail changing magnetic field). However, it's takes a lot of current, which causes two problems: Power supplies don't give enough current and the resistive heat may melt the coil after a long enough time. People usually use capacitors that can supply the high current, but only for a brief pulse.
