1
$\begingroup$

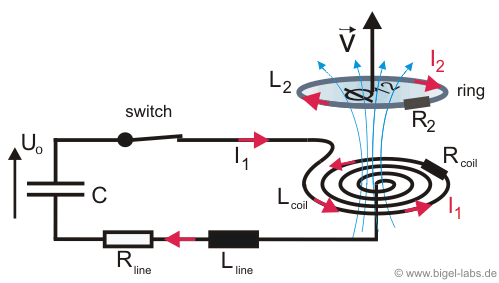
Consider Thomson's jumping ring experiment, where a conducting ring is repelled from a coil after you switched a current in the coil on. The following experiment shows a simplified schematics of the experiment (without an iron core for simplicity and by using a capacitor as energy source). For simplicity also assume that the experiment is done for example in outer space, without considering gravity.

enter image description here

(Source)

The question is about the details of the energy conservation in this context:

We start with the capacitor fully charged and open switch. Initially all the energy is stored in the electric field of the capacitor. If we close the switch the current starts to flow, and the energy in the capacitor decreases. Some energy is converted into heat due to the $R_{\mathrm{line}}$ and $R_{\mathrm{coil}}$ and due to the induced current and $R_2$. Another part of of the energy is converted into kinetic energy of the accelerated ring. The rest of the energy is stored in the magnetic field (produced by the coil and the current in the ring).

I think one may further assume that all resistance is zero so that there is no conversion to heat to consider (but I am not sure if it makes the analysis clearer).

Now if the ring moves away from the coil, the magnetic field decreases and such also $\dot \Phi$. Thus the current tends to decrease as well during a finite time interval $\Delta t$.

If (hypothetically) the ring wouldn't be repelled but attracted by the coil, the current would increase by the same reason, additionally the magnetic field produced by the ring would increase the net magnetic field which would also lead to an increased current during the time interval $\Delta t$.

Now it is often said that the second case wouldn't be compatible with the principle of conservation of energy. But I don't see clearly why.

So my question is the following: How can one make it much more clearer that the energy conservation principle would be violated in the attraction case and how can I express this with formulas more exactly? How can I see that energy is actually conserved in the repelling case?

Note that this is a follow up question to https://physics.stackexchange.com/a/401583/6581:

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

This is quite a complicated variably-coupled mutual inductor set-up, but we can still see how repulsion of the ring is consistent with Lenz's law and energy conservation, whereas attraction wouldn't be.

Repulsion occurs for $I_2$ in the sense you have shown. But $I_2$ in this sense produces a field opposing that due to $I_1$ and reduces the volume integral of $B^2$ in the vicinity of the coils, so therefore reduces the energy stored in the magnetic field, in agreement with energy conservation.

But, you argue, it's not as simple as that. The pulse of 'downward' flux produced by $I_2$ induces a 'anticlockwise (counterclockwise)' emf in the bottom coil, and so causes $I_1$ to increase in the anticlockwise sense, tending to restore the field energy. But the increase in $I_1$ also causes the capacitor to yield up energy more quickly. Again we have energy conservation.

Now run through what would happen if the ring were attracted.

Improve this answer
$\endgroup$
6
  • $\begingroup$ But why would the energy conservation violated if the $B^2$ volume integral would increase (in the attracting case) and thus the magnetic energy? So in the repulsive case you have some arguments that the energy in the magnetic field is less than in the attractive case. But how to conclude that the first case satisfies the energy conversion and the second not? One might think that in the first case that the energy in the magnetic field is too little to fullfill energy conservation. $\endgroup$
    – Julia
    Commented May 3, 2020 at 13:03
  • $\begingroup$ "But why would the energy conservation violated if the $B^2$ volume integral would increase (in the attracting case) and thus the magnetic energy?" The obvious answer is because you'd then be getting both kinetic energy (of the ring) 𝑎𝑛𝑑 extra magnetic field energy, instead of one at the expense of the other. Perhaps in my answer I should have concentrated on the attractive case, in which energy is fairly obviously $not$ conserved. I agree that in the repulsive case I have not shown the energy $is$ conserved, except by elimination of the attractive case! $\endgroup$
    – Philip Wood
    Commented May 3, 2020 at 14:20
  • $\begingroup$ I don't get your ("obvious") point because you get kinetic energy and magnetic energy from the electric energy of the capacitor in the initial state. So assume you have one unit of electric energy in the initial state (which is equal to the total energy). Maybe after some time you have 0,7 units of electric energy, 0,2 units of magnetic energy and 0,1 units of kinetic energy and conceive that the ring is attracted. In this hypothetical case the conservation of energy would be valid... $\endgroup$
    – Julia
    Commented May 3, 2020 at 19:17
  • $\begingroup$ ... If in this world it would be repelled, you would have say 0,7 units of electric energy, 0,1 units of magnetic energy and maybe 0,1 units of kinetic energy which would not be conserved (or maybe also 0,2 units of kinetic energy, then the energy conservation would be also compatible with it). $\endgroup$
    – Julia
    Commented May 3, 2020 at 19:18
  • $\begingroup$ No doubt you are familiar with the thought experiment of a conducting rod laid across two rails joined by a resistor at one end. A uniform magnetic field is applied at right angle to the plane of rod and rails, and the rod is moved steadily along the rails so that it cuts magnetic flux. Are you convinced by the energy conservation argument that predicts the direction of the induced emf in this case (which is simpler than the jumping ring)? $\endgroup$
    – Philip Wood
    Commented May 4, 2020 at 18:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.