horizontal motion inside a cone (cylindrical polars)

Question

I have a question from an example we done in lecture

Suppose we have a particle moving inside the surface of a cone given by $r = wz$ where $w$ is a constant,

and also suppose initially the particle is moving horizontally at height $b$ and speed $U$,the lecturer then stated these "facts"

$\underline{\dot r} = U \underline{e_\theta}$ so $\dot r = 0$, $\dot z = 0$

I understand where he got $\underline{\dot r} = U \underline{e_\theta}$, however, I do not understand why $\dot r = 0$ and $ \dot z = 0$, all we know is that $z(0) = b$, and $\dot r(0) = U$, but that does not mean general $\dot z, \dot r$ are $0$,

if someone could clear this up please

edit: !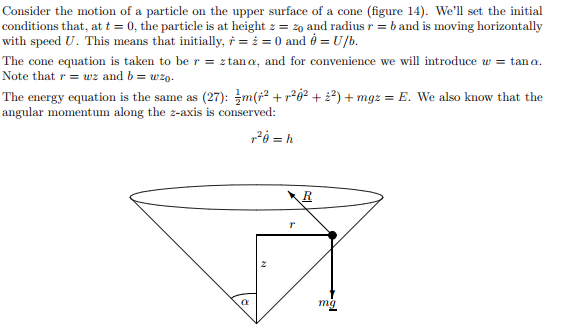

Answer 1

It says "initially, $\dot r = \dot z = 0$". This is synonymous with $\dot r(0) = \dot z(0) = 0$. Vague wording like that is usually to trick students.

Answer 2

You've missed the phrase "and is moving horizontally". Since the cone is oriented vertically, the particle will continue moving horizontally, that is, in a horizontal circle. Its height z will remain constant, and its radius r will also remain constant.