Which component shows spin squeezing under twisting Hamiltonian?

Question

Given a many body spin system, a collection of N spin-1/2 particles, under the interaction of the twisting Hamiltonian: $$H_{int} = \sum_{i,j=1}^Na_{i,j}\sigma_{z,i}\sigma_{z,j}= A J_{z}^{2}$$ assume all $a_{i,j}$ are equal and define: $$\mathbf{J} = \sum_{n=1}^{N} \mathbf{\sigma}_{n}$$ the collective spin operator, $\mathbf{\sigma}_{n}$ is the pauli spin operator for the $n$th spin, and $A$ characterizes the strength.

Which component of the spin system will display reduced variance/will be squeezed? What assumptions does this require regarding the initial state?

The context of the question is the notion of spin squeezed states, as originally put forward by (1) Wineland et al. and (2) Kitagawa & Ueda

EDIT

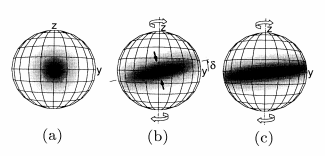
In particular in figure 2 of (2) the evolution of the coherent spin state $|\pi/2,0\rangle$ (pointing along $\hat{x}$) is described, they show that squeezing occurs along the y- and z-axes. As I see it these variances seem to oscillate out of phase.

What would be helpful is if someone could explain how to visualize this so-called twisting dynamics. So far i thought that, starting with an initial (Q-)distribution in phase space, the distribution evolves with precession frequency proportional to $J_{z}$. But from there I do not see how the variance along $\hat{z}$ would change...

Also as a side note: to my knowledge these type of quadratic in angular momentum terms are not very common, but also appear in nuclear physics as the Lipkin model.

Answer 1

The hamiltonian $$H=AJ_z^2=A\left(\sum_j S_z^{(j)}\right)^2\tag1$$ is a function of the individual $z$ spin projections $S_z^{(j)}$, and all of those commute. Therefore, the eigenstates will be product states of the form $$|\Psi\rangle=|a_1\rangle\otimes\cdots\otimes|a_N\rangle,\tag2$$ where each $|a_j\rangle$ is either $|\!\uparrow\rangle$ or $|\!\downarrow\rangle$. As such, the system is easily solvable, and you simply need to phrase your questions correctly. The expectation value $\langle J_x^2\rangle$, for example, is constant and equal to $N$ for all eigenstates of $H$ of the form (2) (though there are degeneracies and superpositions of such eigenstates may yet be squeezed).

---

Edit:

OK, I think I know what's confusing you. In particular, from your question edit:

Starting with an initial (Q-)distribution in phase space, the distribution evolves with precession frequency proportional to $J_z$. But from there I do not see how the variance along $\hat z$ would change.

The second paper starts off the atoms in a spin-coherent-state cloud along the $+x$ pole on the Bloch sphere, and then lets them evolve according to the hamiltonian (1).

This means that points closer to the $+z$ pole have more positive energy, accumulate more phase on their up components than their down ones, and therefore rotate towards the right. Similarly, points closer to the $-z$ pole have more negative energy, accumulate more phase on their down components, and rotate towards the left.

The net effect of this is to shear the cloud on the Bloch sphere, whilst preserving its height. In particular, the $z$ marginal, i.e. the distribution of ups and downs in a $z$ measurement, is not affected, as it should. As a consequence, the variance in $z$ is not (yet) affected.

You can see, of course, that the cloud is now longer (in that its variance in $y$ is now much greater), and also thinner, in a slightly off-diagonal direction. Thus, to obtain squeezing in the $z$ direction, you need to rotate slightly about the $x$ direction. By how much, of course, is a question of exactly what the circumstances are, and it's a function of the product of $A$ and the interaction time; if you want the details, you should first give Kitagawa and Ueda's paper a thorough read, I think.

This is quite a popular protocol for obtaining spin-squeezed states, I think:

1. Prepare a spin coherent state,
2. shear it using interactions, and finally
3. rotate it to the measurement direction.

The third step is crucial, because the shear does not affect the width along the interaction direction, which is what I was nagging you about in the first part of this answer. Once you rotate it, though, you can get reduced (or vastly increased) variances in any component.