How can I derive the stress tensor for a Newtonian fluid in more physical terms?

Question

The question is quite fundamental and more on a beginner's level (not sure if good in this high-level-forum, but I try): I have big problems in understanding the stress tensor for Newtonian fluids in terms of velocities u.

The result (assuming $\mu'=0$) is (according to my text book)

$\tau_{ij} = \mu (\partial _j u_i + \partial _i u_j -\frac{2}{3}\delta_{ij} \partial _k u_k)$

I know the derivation of that, but it is not very intuitive from a physical perspective. I would like to understand in particular, why we have the term $\partial _j u_i$

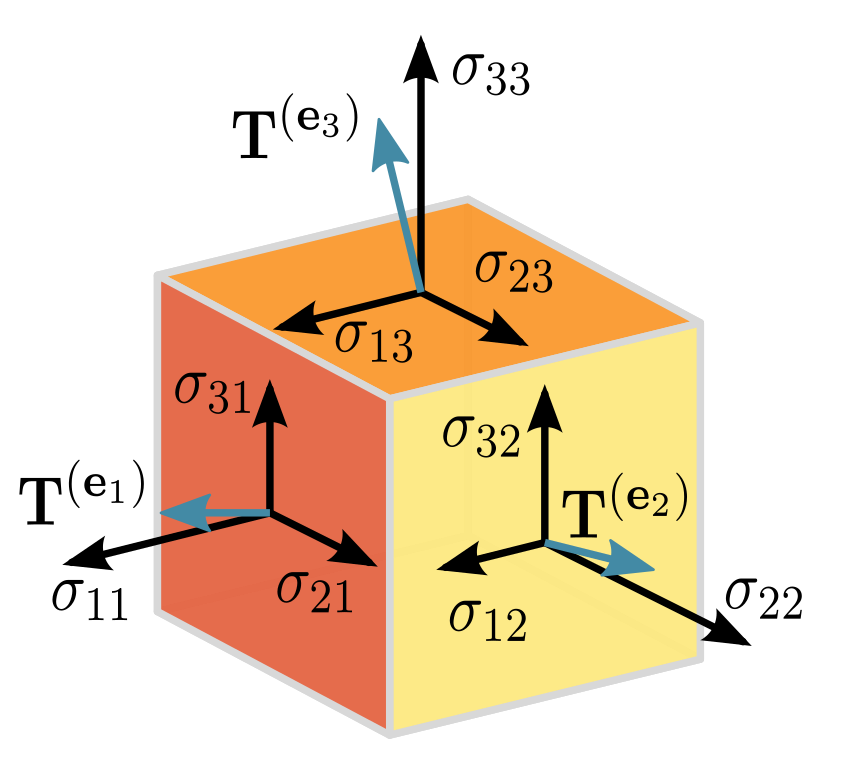
From the following image and the definition of viscous stresses I would naively expect the stress in 1-direction on surface-2 just to be

$\sigma_{12} = \mu \partial _2 u_1$

Why is there also the contribution $\partial _1 u_2$ ?

And where does the symmetrical additional 2/3-term come from?

I understand readily the derivation of my textbook, but this is rather mathematically and I cannot see physics behind (yet).

EDIT:

I have seen that asymmetry is a consequence of having zero momentum along all axes. I didn't recognize that, but now its clear.

Answer 1

It's subjective to say what's "intuitive" for one person, but here's one way to think about it based on three physical assumptions.

You could a priori say that the stress tensor $\bar{\bar{\sigma}}$ is a linear function of the velocity gradient tensor $\nabla\vec{v}$. There are a couple of physical ways to justify this: the current state of stress is independent of the previous states of stress, the relationship is invariant with respect to classical inertial frames so no dependence on $\vec{v}$, etcetra.

This means you can represent the stress-strain relationship as

$$\bar{\bar{\sigma}} = \mathcal{C}\nabla\vec{v}$$

where $\mathcal{C}$ is a fourth-order tensor. Now it's a question of figuring out what elements of the tensor $\mathcal{C}$ are zero.

We can then assume that the stress-strain relationship doesn't change with respect to the orientation of the way you look at it, which mathematically translates to the $\mathcal{C}$ tensor being isotropic. This is certainly true for simple liquids, where we assume the constituent atoms/molecules are approximately spherical for all intended purposes, but isn't true if we have rod-like polymers/molecules making up our fluid. (Doing the math, this kills off a great deal of elements in the above tensor.)

This isotropy, combined with the assumption that the fluid does not carry surface couples or internal torques, will kill off any remaining extra terms and immediately generates the Newtonian fluid model. (This assumption is almost always true expect for materials that have nontrivial magnetic coupling or some other exotic effects.)

Hope this helps!

Answer 2

We know that, in general, velocity gradients in a fluid give rise to viscous forces.

There is, however, one case (at least) where velocity gradients exist but no viscous forces exist. That is when the fluid rotates as a rigid body. That second term, I think, is needed to ensure this.