My professor said that the electric field is zero wherever two equipotential surfaces intersect. I can't come up with a reason why.
He also claimed two equipotential surfaces cannot intersect as that would give two different potentials at the same point. Why can't there just be two different equipotential surfaces with the same potential that intersect or touch?
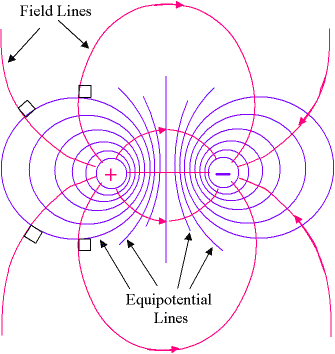
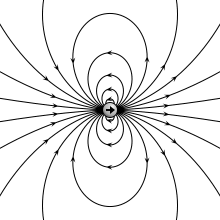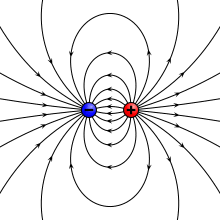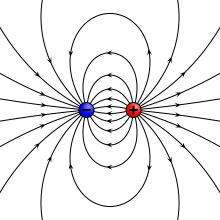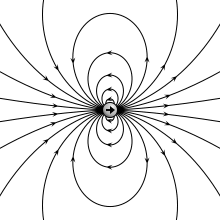
First of all, let's clear the air with a simple example that showcases the desired behaviour (and which is essentially isomorphic to most nontrivial cases). Consider in particular, the following claim:
The potential $V(x,y,z) = V_0 \, xy$ is a perfectly valid electrostatic potential, and it can very naturally be seen as having two equipotential surfaces (the $yz$ plane and the $xz$ plane) that intersect along a line.
That example can be jarring to the usual intuition that equipotential surfaces, like field lines, never cross, but it checks out perfectly - and it is consistent with your professor's claim that the electric field, $$\mathbf E = -\nabla V = -V_0(y\,\hat{\mathbf x} + x\,\hat{\mathbf y}),$$ vanishes at the intersection $x=y=0$.
(For those that would like to extend the envelope a bit further: this naturally generalizes to the intersection of any number $n$ of equipotential surfaces along a line, by simply changing to the $n$-polar potential $V(x,y,z) = V_0 \,\mathrm{Re}\mathopen{}\left[\left(x+i y\right)^n\right]\mathclose{}$.)
So, what's going on, or how do we provide some real mathematical meat to the statement at hand?
Well, let's start by defining equipotential surfaces: a surface $S:(D\subseteq\mathbb R^2)\to\mathbb R^3$ is an equipotential of the electrostatic potential $V:\mathbb R^3\to\mathbb R$ iff $V(S(u,v))=V_0$ is constant for all $(u,v)\in D$. Moreover, we know that at any point $\mathbf r=S(u,v)$ on the surface, the electric field $\mathbf E = -\nabla V$ has a zero inner product with any vector that lies inside the tangent plane $TS_\mathbf r$ to the surface at $\mathbf r$, as a consequence of taking curves $\gamma:(a,b)\to D$ and differentiating the constancy relation $V(S(\gamma(t)))\equiv V_0$ with respect to the parameter $t$, giving $$ -\dot\gamma(t)\cdot \nabla V = \dot\gamma(t)\cdot \mathbf E = 0 $$ for all vectors $\dot \gamma\in TS_\mathbf r$. Since that plane is two-dimensional and space is three-dimensional, we infer that there is a unique normal direction $\hat{\mathbf n}$ to the surface and that $\mathbf E$ needs to be parallel to that normal (or, possibly, zero), but the core result is that $\mathbf E$'s component along any direction inside the tangent plane must vanish.
OK, so now let's up the ante and consider two different surfaces $S_i:D_i\to \mathbb R^3$, $i=1,2$, which intersect at some point $\mathbf r_0$, and let's also stipulate that both surfaces are equipotentials of $V$.
Right off the bat, we can infer that the potential at all points on both surfaces must equal the same constant, because $V=V(\mathbf r)$ is a (single-valued) function. If it equals $V(\mathbf r_0)=V_1$ for $\mathbf r_0\in S_1$, then it must equal $V_1$ throughout $S_1$ - but $\mathbf r_0$ is also in $S_2$, so $V$ must also equal $V_1$ throughout $S_2$. This is probably what your professor was talking about in the claim that you report as
He also claimed two equipotential surfaces cannot intersect as that would give two different potentials at the same point,
but which was quite likely to be much closer to
two equipotential surfaces with a different potential cannot intersect as that would give two different potentials at the same point.
That's the easy bit. Let's now say something nontrivial: what about the electric field at the intersection?
Let's start with the easy case first, though, and assume that the equipotentials have a proper dimension-one intersection along a curve, which implies that, at any point $\mathbf r$ along the intersection, the tangent planes to the two surfaces will intersect on a line, and each of them will have a separate, linearly independent direction that does not belong to the other plane.
This then lets us bring in the tools we developed earlier: we know that $\mathbf E$ needs to have vanishing inner product with any vector that lies inside of either tangent plane, except that now we have three linearly independent vectors $\mathbf e_1, \mathbf e_2$, and $\mathbf e_3$ to vanish against, one along the intersection and one other independent vector along each plane. The only way that any vector $\mathbf v\mathbb R^3$ can satisfy $\mathbf v\cdot \mathbf e_i=0,$ for linearly-independent $\mathbf e_i,$ is for $\mathbf v=0$. This is where your professor's claim comes from.
Finally, let's address the slightly more pathological case you mention at the end of your question:
Why can't there just be two different equipotential surfaces with the same potential that [...] touch?
This isn't a bad question, and the answer is essentially that this can happen, but the circumstances in which it does happen are so pathological that we're mostly ready to throw that baby out with the bathwater. When we say "two surfaces intersect", we normally mean that they have a dimension-one intersection along a curve; if we want to allow the surfaces to touch, or have some similarly pathological behaviour, then we'll explicitly note that. (Mathematicians are a bit more careful with their language, but then again physicists do more interesting stuff and you can't waste time fiddling with minor details.)
Anyways, if you want a potential with two equipotentials that touch at a single point, the cleanest example I can think of is $$ V(x,y,z) = z^2-(x^2+y^2)^2, $$ where the equipotentials $V(\mathbf r)=0$ are two circular paraboloids that touch at their apex. This isn't a solution of the Laplace equation, which means that it's not a reasonable potential in free space, but you can just set the charge density $\rho \propto \nabla^2 V$, and you'll get some reasonable distribution. If you want to economize on that, then it's better to choose $$ V(x,y,z) = z^2-(x^2+y^2)z, $$ for which the charge density $\rho \propto \nabla^2 V = 2-4z$ is extremely reasonable, and which swaps out one of the paraboloids for the $z=0$ plane.
Now, for both of these examples, you have a pretty high-order polynomial as your potential, and the electric field vanishes at the equipotentials' intersection point. If you want to have something with touching equipotentials and a nonzero electric field there, the closest that I come up with in a clean way is to combine the two examples above, giving three equipotentials (the two paraboloids and the $xy$ plane) meeting at a point, $$ V(x,y,z) = \left(z^2-(x^2+y^2)^2\right)z, $$ with a $V(0,0,z)=z^3$ dependence along the $z$ axis, and then to factor that out by taking a cube root, giving $$ V(x,y,z) = \left[\left(z^2-(x^2+y^2)^2\right)z\right]^{1/3}, $$ which has the same touching equipotentials as above but now it has a constant electric field $\nabla V = (0,0,1)$ on all points $(0,0,z)$ with $z\neq 0$. Unfortunately, however, you can't really conclude that the electric field there is nonzero, because the limits to $\mathbf r\to0$ along the $z$ axis and along the $xy$ plane don't commute - and, indeed, $\nabla V$ diverges everywhere on the $xy$ plane.
I'll draw here the equipotential landscape when cut along the $xz$ plane, to give an idea of the type of pathological structure that you'll be pushed to by considering this type of cases:
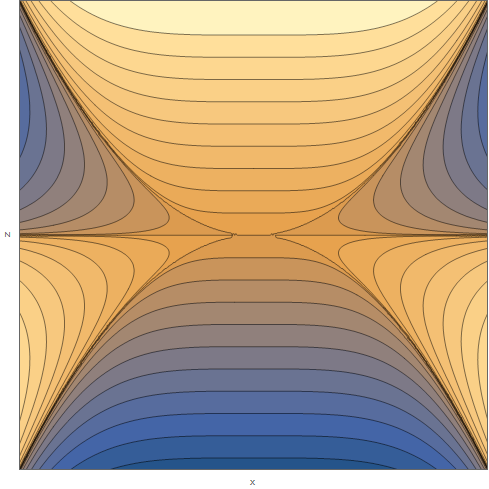
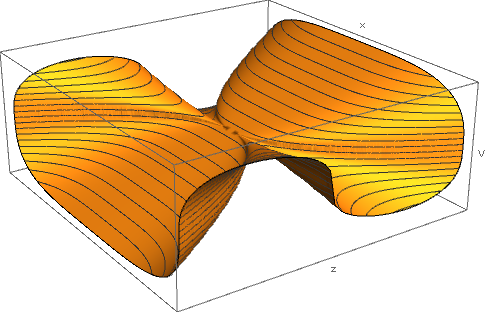
Source: Import["http://halirutan.github.io/Mathematica-SE-Tools/decode.m"]["https://i.sstatic.net/0snLs.png"]
The sharp cliff faces at the equipotentials on the 3D view of $V(x,0,z)$ are clear markers of the fact that the electric field is infinite everywhere at the $V=0$ equipotentials, with the lone exception of the origin when approached from the $z$ axis.
Anyways, that's the kind of price you need to pay to have equipotentials that touch without that requiring a zero electric field at the touching point to keep everything nice and smooth. In general, though, you just throw those cases out by decree by requiring a regular intersection.
Electric field is defined as the (negative) gradient of electrostatic potential. There can therefore be no electric field along the line/surface defined by an equipotential.
That means that the only electric field allowed at a point on an equipotential must be perpendicular to the equipotential surface, otherwise it would have a non-zero component along the surface.
If there are two different intersecting equipotentials, then the only valid electric field is zero, since any non-zero field would have a non-zero component along at least one of the equipotentials.
An exception would appear to be where the equipotential surfaces are parallel at their intersection.
Two equipotential surfaces can't intersect. The direction of the electric field at any point on an equipotential surface is perpendicular to the surface at that point. If two equipoential surfaces were to intersect, then the electric field at the points of intersection would be perpendicular to both the first surface and second surface at those points...in other words, if two equipotential surfaces could intersect, you'd have the electric field pointing in two directions at each point of intersection.... one pointing perpendicular to the first surface, the other pointing perpendicular to the second surface. This is impossible.
Because if they were to intersect then direction of electric field is ambiguous so it is not possible.
He also claimed two equipotential surfaces cannot intersect as that would give two different potentials at the same point.
Consider the electric field and equipotential surfaces of an electric dipole
None of the equipotential surfaces intersect. Also, the density of the surfaces is greatest along the line between and through the two charges.
Now, consider those equipotential surfaces in the limit of an ideal electric dipole.
For constant dipole moment, the (plus/minus) charge must increase as the separation distance decreases, the density of the equipotential surfaces along the line through the surface must diverge in the limit; it seems that all of the equipotential surfaces must intersect at the location of the ideal dipole and the electric field is singular there.
