Relativistic aberration paradox

Question

I'm racking my brain over what should be a simple problem in special relativity. Consider the angle $f$ between two stars, as measured by a an observer at rest:

The observed angle should be the same whether the observer is moving towards the stars, or the stars are moving towards the observer. In the first case, we have the classic formula for stellar aberration: $\cos f' = \frac{v/c + \cos f}{1+v/c \cos f}$

But in the latter case, it seems to me that the star can simply emit light at the angle $f''$ with $\cos f''=\frac{\cos f - v/c}{1-v/c \cos f}$ and still reach the observer:

So in the first case, the observer measures the stars to be $f'$ apart, but in the second case, they appear to be $f$ apart. What am I missing?

Answer 1

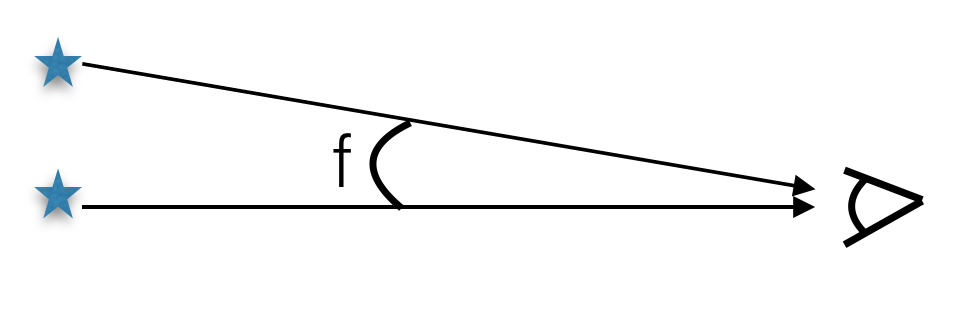
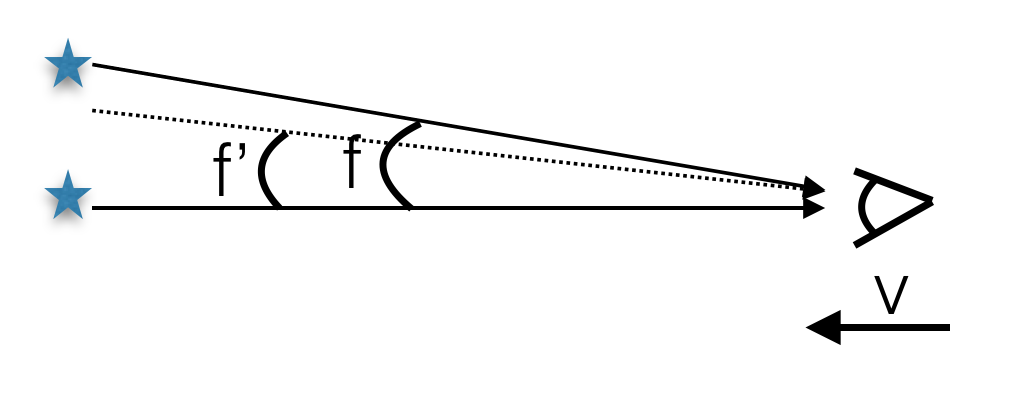
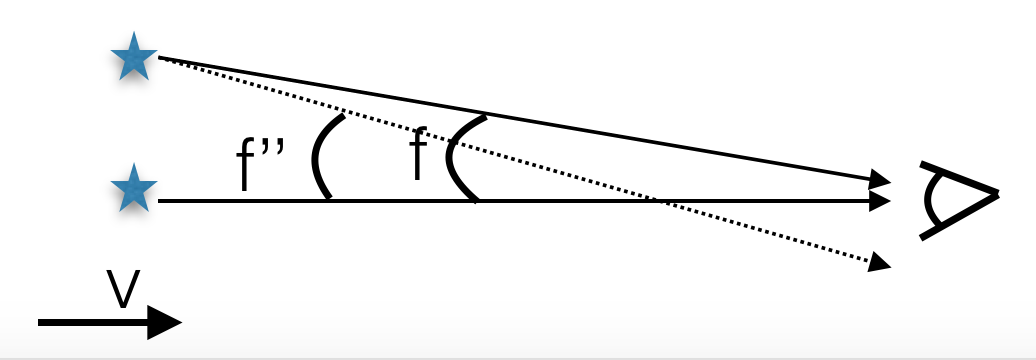
It is hard to tell exactly what the question is asking. The best I can do is demonstrate how relativistic aberration actually works. This is very closely related to relativistic beaming. (Aberration is the change in apparent angle and beaming is the change in apparent brightness.)

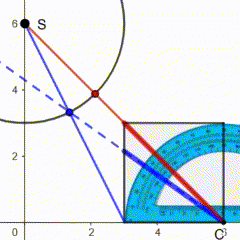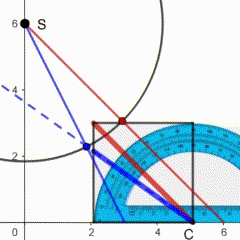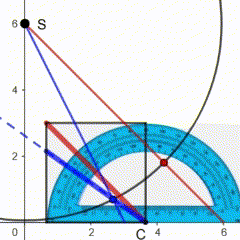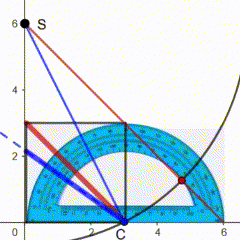
In the above animation, the box contains two telescopes pointed at star S. The red telescope is at 45 degrees and points directly at the star when the box is stationary. When the box is moving towards the star, the light appears to be coming from a shallower angle (now about 36 degrees with the observer moving at 0.8c towards the source) and the blue telescope appears to be pointing directly at the star. It is a bit like running in the rain. Raindrops that appeared to be coming straight down when we were standing still now appears to be coming from somewhere in front when running. Note that the light we see when moving, left the star at a wider angle and when it is received it appears to come from a narrower angle. The end result is the star appears brighter and nearer. In other words, when the observer is moving, we have to rotate the telescope from the red position to the blue position. We can of course mentally reverse the situation and imagine the box represent the star and S represents the the observer. When the star is stationary the light radiates energy evenly in all directions. When the star is moving towards the observer, light that was originally emitted in a 45 degree wide cone, is now emitted in a narrower cone. Whichever way you look at it the light appears to be focused into a tighter beam and the star looks brighter when the observer is moving towards the star.

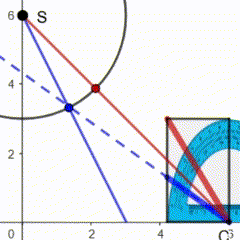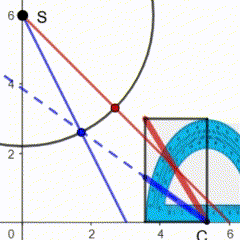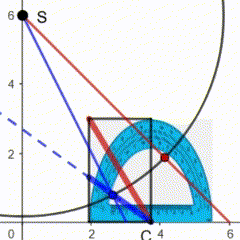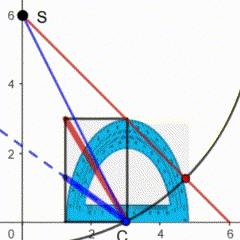
This second animation takes relativistic length contraction into account. This alters the geometry again and the light appears to be arriving with an even shallower angle (about 24 degrees). This makes the star look even brighter from their point of view. We also have to take time dilation into account. Time dilation causes the number of photons received per second to be higher, so the apparent brightness of the star is even brighter than the classical expectation. All this can be reversed, so when the source is moving away from the observer, the brightness is greatly diminished.