1
$\begingroup$

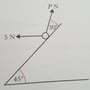
enter image description here

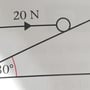
I'm practicing mechanics, and I had to resolve the following forces perpendicularly to the inclined plane in order to work out the reaction force (plus the weight of the ball)

But I cannot tell whether the 5N force is going up or down when resolved perpendicularly to the inclined pline. According to the book, the answer is up. I thought maybe because the force acts at 45 degrees to the plane, and so therefore if it's 45 degrees or more, then the force has to be acting upwards

However, for this question (the angle of the plane to the horizontal is 30 degrees, it's not very clear in the picture)

The angle of the plane to the horizontal is 30 degrees

the 20N force acts downwards in the component perpendicular to the plane, whereas if I use my aforementioned reasoning, I would expect it to act upwards, since it would have to be more than 45 degrees to the plane to act downwards.

So I don't really understand how I am to determine whether a force's perpendicular component to a plane is up or down when it is acting on an inclined object, and my attempts to do so have contradictions.

Improve this question
$\endgroup$
4
  • $\begingroup$ The 5N and 20N forces look like they are horizontal, and therefore would have no vertical component. $\endgroup$
    – mbeckish
    Commented Feb 16, 2016 at 15:14
  • $\begingroup$ @mbeckish The forces are being resolved relative to the plane $\endgroup$
    – 83457
    Commented Feb 16, 2016 at 15:16
  • $\begingroup$ What do you mean by that? Maybe you need to define "vertical" for us. Do you mean: perpendicular to the inclined plane, parallel to the inclined plane (i.e. "uphill"), or parallel to gravity? $\endgroup$
    – mbeckish
    Commented Feb 16, 2016 at 15:19
  • $\begingroup$ @mbeckish my bad, yes I meant perpendicular to the inclined plane $\endgroup$
    – 83457
    Commented Feb 16, 2016 at 15:22

2 Answers 2

Reset to default
0
$\begingroup$

Definition: The "tail" of a vector is the end that is straight (no arrow). Definition: The "head" of a vector is the end with the arrowhead.

For each force, draw a right triangle such that:

  1. The original force line is the hypotenuse
  2. Draw a vector with the tail starting at the tail of your original force, pointing parallel to the plane.
  3. Draw a vector with the tail starting at the head of the vector from step 2, perpendicular to the plane, ending at the head of the original vector.

The direction of the vector from step 3 gives you your answer.

Improve this answer
$\endgroup$
4
  • $\begingroup$ I am not near a pen and paper right now, so could you briefly explain your reasoning behind this, and why it would work for every situation $\endgroup$
    – 83457
    Commented Feb 16, 2016 at 15:33
  • $\begingroup$ Physically, the meaning of the triangle is: You can remove the original force (the hypotenuse), replace it with the two perpendicular components, and the object won't know the difference - everything would behave exactly the same. So, when trying to calculate things like normal force and acceleration along the plane, you can either 1) just keep the original force as drawn, and use sine, cosine, etc. to try to calculate the normal force, etc., or 2) replace the original force with its parallel and perpendicular components, and then use them to reason about the normal force, etc. $\endgroup$
    – mbeckish
    Commented Feb 16, 2016 at 15:41
  • $\begingroup$ The advantage to the second technique is that the perpendicular forces have no effect on each other. So when calculating the normal force, you only have to look at the forces perpendicular to the plane, and can ignore the forces parallel to the plane. When calculating acceleration along the plane, you can ignore the perpendicular forces, and only look at the forces parallel to the plane. $\endgroup$
    – mbeckish
    Commented Feb 16, 2016 at 15:41
  • $\begingroup$ By the way - you will do the same thing to gravity. You will make the original force of gravity the hypotenuse, and replace it with components parallel and perpendicular to the plane. This will show you how much gravity (the weight of the object) contributes to the normal force, and how much of the weight contributes to it accelerating down the plane. $\endgroup$
    – mbeckish
    Commented Feb 16, 2016 at 15:43
0
$\begingroup$

There is a trick that every mechanical engineer uses to solve the problems on mechanics

firstly draw a perpendicular at the point of application of the force on the plane.

then make an arrow along this line which you have constructed and along the plane. this arrow will point to the gross direction of the vector .

like in your first example the arrow on the construction line will be in the upward direction

but in the second case the arrow direction will be in the downward direction. this is a homework question so according to the policy of the cite I can guide and not give the exact solution.

Hopw you can find the hit useful

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.