Current has both magnitude and direction. As per the definition of vector defined in encyclopedia, current should be a vector quantity. But, we know that current is a scalar quantity. What is the reason behind it?
-
3$\begingroup$ THe direction should really be considered to be "sign" more than a proper vector direction. In particular, Current is defined by defining a surface, and then counting the number of particles that cross that surface per unit time. It only depends on the relative orientation of the surface and the charges, it has no absolute notion of distance. More mathematically, the vector nature of these things is dotted out: $I=\int{\vec j} \cdot d{\vec A}$ $\endgroup$– Zo the RelativistCommented Dec 5, 2014 at 17:52
-
1$\begingroup$ @AntoniosSarikas, no, because net charge is crossing the surface in question. The current is zero if an equal number density of protons and electrons are moving in the <i>same</i> direction at the same speed.. $\endgroup$– Zo the RelativistCommented Sep 10, 2020 at 15:49
-
1$\begingroup$ @AntoniosSarikas as far as current is concerned, the flow of an electron is just the same thing as the flow of an antiproton. So, if you have 1 C/s of rightward flow of electrons and 1 C/s of leftward flow of protons, you just have a current of 2 C/s leftward $\endgroup$– Zo the RelativistCommented Sep 10, 2020 at 17:44
-
1$\begingroup$ Another way of thinking of it: I have a box. The box contains 20 C of charge. The current is how much charge is entering or leaving the box per unit time. I can get to 21 C by either adding 1 C worth protons, or removing 1 C worth of electrons, but either way, the net charge of the box is 21 C, and so the rate of change of that charge won't care whether I'm adding protons, removing electrons, or some combination of the two. It just cares about my net charge. $\endgroup$– Zo the RelativistCommented Sep 10, 2020 at 22:18
-
1$\begingroup$ @JerrySchirmer Your two previous answers covered my doubts. It is the net charge therefore and also the direction is a matter of convention. If we think two boxes and a net charge flowing from one box to the other then at every moment we can calculate their amount of charge. If we have a positive flux (convention from left to right) of 5 C/s then the left box will decrease its amount by 5 C/s whereas the right will increase it by 5. $\endgroup$– Antonios SarikasCommented Sep 17, 2020 at 19:15
5 Answers
To be precise, current is not a vector quantity. Although current has a specific direction and magnitude, it does not obey the law of vector addition. Let me show you.
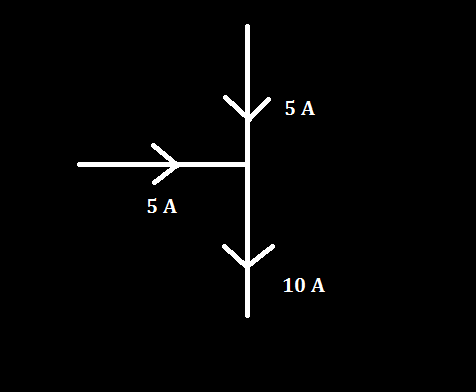
Take a look at the above picture. According to Kirchhoff's current law, the sum of the currents entering the junction should be equal to sum of the currents leaving the junction (no charge accumulation and discharges). So, a current of 10 A leaves the junction.
Now take a look at the picture below.
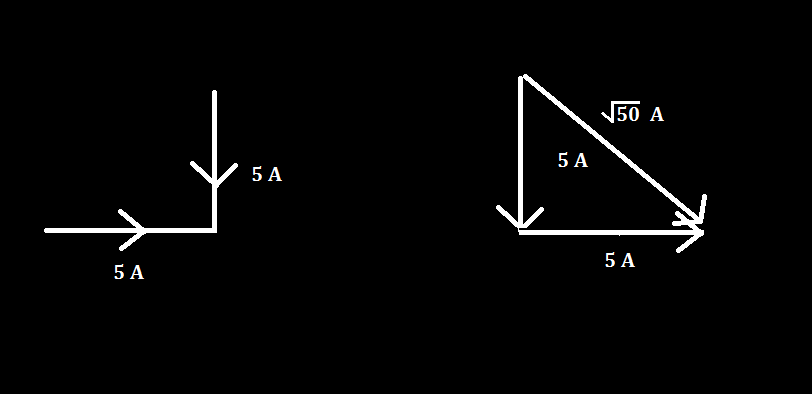
Here, I have considered current to be a vector quantity. The resultant current is less than that obtained in the previous situation. This result gives us a few implications and I would like to go through some of them. This could take place due to charge accumulation at some parts of the conductor. This could also take place due to charge leakage. In our daily routine, we use materials that are approximately ideal and so these phenomena can be neglected. In this case, the difference in the situations is distinguishable and we cannot neglect it.
If you are not convinced, let me tell you more. In the above description (current as a vector), I have talked about the difference in magnitudes alone. The direction of the resultant current (as shown) is subtle. That's because in practical reality, we do not observe the current flowing along the direction shown above. You may argue that in the presence of the conductor, the electrons are restricted to move along the inside and hence it follows the available path. You may also argue that the electric field inside the conductor will impose a few restrictions. I appreciate the try but what if I remove the conductors? And I also incorporate particle accelerators that say shoot out proton beams thereby, neglecting the presence of an electric field in space.
Let me now consider two proton beams (currents), each carrying a current of 5 A as shown below. These beams are isolated and we shall not include any external influences.
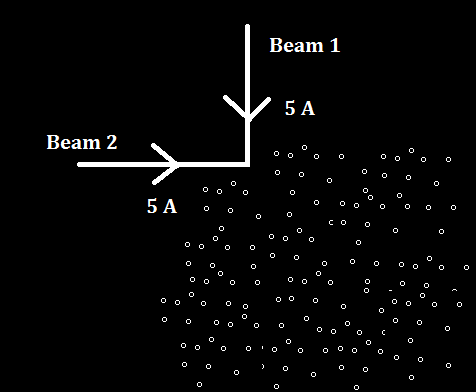
Now that there is no restriction to the flow of protons, the protons meeting at the junction will exchange momentum and this will result in scattering (protons represented by small circles). You would have a situation where two beams give rise to several beams as shown below. Our vector addition law does not say this.

I have represented a few in the picture above. In reality, one will observe a chaotic motion. Representation of the beams (as shown right above) will become a very difficult task because the protons do not follow a fixed path. I have just shown you an unlikely, but a possible situation.
All this clearly tells us that current is not a vector quantity.
Another point I would like to mention is, current cannot be resolved into components unlike other vector quantities. Current flowing in a particular direction will always have an effect along the direction of flow alone over an infinite period of time (excluding external influences such as electric or magnetic fields).
-
11$\begingroup$ If a quantity is not a vector, this doesn't make it scalar. $\endgroup$– RuslanCommented Oct 30, 2014 at 9:14
-
1$\begingroup$ Scalar quantity is a quantity, which is invariant under rotations. Current is not invariant under rotations. Also, the physical quantities can include pseudotensors (including pseudoscalars), and even things like sum of tensor and pseudotensor - such quantities are definitely not among those you listed. $\endgroup$– RuslanCommented Oct 30, 2014 at 16:15
-
1$\begingroup$ In fact, it may appear scalar, depending how you define differential cross-section vector $d\vec A$ in definition of current $I=\int\vec Jd\vec A$. If you define it so that it changes direction under parity inversion, then $I$ is pseudoscalar. Otherwise, it's scalar. Or I may be wrong, and $d\vec A$ has some unambiguous definition. $\endgroup$– RuslanCommented Oct 30, 2014 at 18:02
-
1$\begingroup$ Wikipedia talks about a four dimensional analog of 'electric current density'( a vector quantity well known to us in our three dimensions ). I'm talking about electric current. I hope it is clear. $\endgroup$– R004Commented Feb 4, 2015 at 16:24
-
2$\begingroup$ @Aron the 4-current in special relativity is current density, not current. The two things are different, related, concepts. Current density is a vector, current is a scalar, defined by $I = \int d{\vec A}\cdot {\vec j}$, where I is the current, and $\vec j$ is the current density, and $A$, is, say, the cross section of the wire in question. $\endgroup$ Commented Sep 10, 2020 at 17:48
I think there might be a contextual issue.
If you're passing DC current in a circuit, it makes sense to treat it as a scalar, because it flows along the wires and you're usually designing the circuit so that its components don't appreciably interact in ways where the wire geometry matters... or rather treating ones that do as separate sub-units, e.g., inductors.
In other words, if your current is constrained to go in one dimension, such as along wires, then it makes sense for it to be treated as a scalar, because a $1$-dimensional vector is a scalar.
But as the case of inductors shows, the direction in space the current is flowing can make a lot of difference electromagnetically. As BMS suggested, more fundamentally the conservation of charge is expressed by a continuity equation $$\frac{\partial\rho}{\partial t} + \nabla\cdot\mathbf{J} = 0\text{,}$$ where $\rho$ and $\mathbf{J}$ are charge and current densities, respectively, quantities that also appear in Maxwell's equations.
Here's some context that may make the original post clear. The force on a current-carrying wire ... $\vec{F}=I\vec{L}\times\vec{B},$ ... As another example, the Biot-Savart law is $d\vec{B}=\frac{\mu_o}{4\pi} \frac{Id\vec{l}\times\hat{r}}{r^2},$ where $d\vec{l}$ is in the same direction as the "current" $I$.
One can obviously rewrite that as $L\vec{I}\times\vec{B}$ and $dl(\vec{I}\times\vec{r})$ if one wished to think of a vector current. The only reason not to would be the fact that your amp-meter tells you directionality along a wire (hence $\pm$) rather than directionality in space, so the fact that it's more convenient the other way.
-
4$\begingroup$ The reason current in a circuit is treated as a scalar is that it does not change just because the wire it is flowing along bends. As you say this is do the context in which you are working. $\endgroup$ Commented Dec 22, 2013 at 1:54
-
$\begingroup$ So then can you use $J$ to describe currents in circuits if you wanted to, even if it was unnecessary? I am confused whether $I$ and $J$ are referring to the same physical thing. $\endgroup$ Commented Feb 8, 2015 at 2:31
Current doesn't follow vector addition and decomposition law, and so it is not a vector quantity. Current density is a vector quantity.
I think a cleaner idea is to show that current has a sign, but it does not have a direction.
In particular, current is the amount of charge crossing a surface per unit time. This can be shown to be equal to the quantity:
$$I = \int d{\vec A} \cdot \rho {\vec v}$$
Where $d{\vec A}$ is the surface element of the surface in question, $\rho$ is the charge density of the fluid, and $\vec v$ is the flow rate of the fluid. This is obviously a scalar quantity, and now it should be clear why it's possible to have positive and negative currents -- it just depends on whether the charge is flowing to the "right" or to the "left" through the surface.
-
$\begingroup$ Griffith clearly says again n again the current is actually a vector. It is clear that current is vector if one talks of surface currents . Only reason the author ignore vector nature of current in wire is because j is constant throughout. But actually current is a perfect vector. Griffith says this at 2 places. Why then he say this ? Is he wrong or the answers here wrong. If you want I can also tell you the pages where Griffith says all this or can post a picture ! $\endgroup$ Commented Mar 23, 2017 at 21:08
-
$\begingroup$ $I = \int {\vec j} \cdot d{\vec A}$. When you say "current", you mean $I$, and when you say "current density", you mean $\vec j$. One is a scalar, and one is a vector. $\endgroup$ Commented Mar 24, 2017 at 14:27
-
1$\begingroup$ @Shashaank: but the key point is that current density is defined at any point, but current is only defined relative to some surface or physical object. I can talk about the "current through this wire", and that is a scalr, but I can't meaningfully talk about the "current at $(x,y,z)$, unless I'm talking about current density. $\endgroup$ Commented Mar 24, 2017 at 14:29
current is definitely a vector quantity as flow of electrons leads to formation of electric force and current also and force is a vector quantity and secondly flow of electrons in per unit time called current and flow of electrons literally means moving in a definite direction
