Let's address your second question.
Suppose there is a neutral conductor in the beginning. Now you transfer some positive charge to it. Lets zoom in to see what happens to a small charge element.
- It experiences the electric field of the constituent electrons and protons.
- It experiences electric repulsion from the other "charge elements".
Since the conductor is neutral, we can say that (on an average) the positive charge density (due to the constituent protons) and negative charge density (due to the continuously moving electrons) cancel each other at each and every point on the bulk of the conductor. So the net effect of [1] is zero.
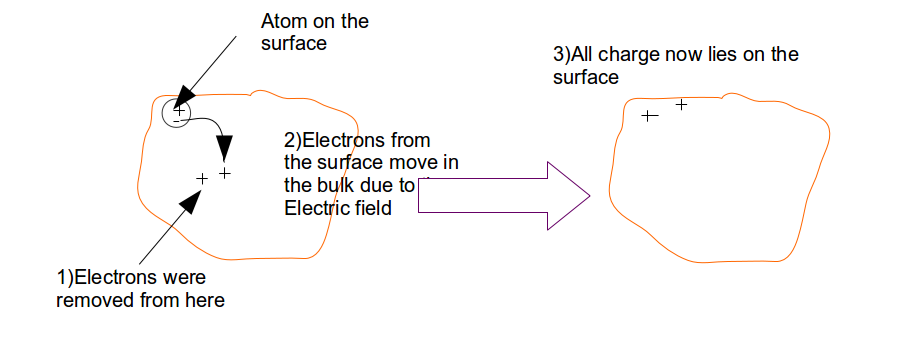
Now lets see how the remaining portion of the excess charge (free charge) affects the charge element in question. Because these are like charges, they induce repulsion. The infinitesimal charge element feels a repulsive force and tries to move away from the initial position. But remember: this is happening with each "charge element". So, it is a nonlinear process: each element moves away to under the repulsive force, this redistributes the remaining charge distribution, which in turn modifies the repulsive force on each individual element, etc. How long does this continue? Until the free charge has redistributed itself (depending upon the shape of the conductor) so as to attain a minimum energy configuration. Once this configuration is reached, we may deduce the following:
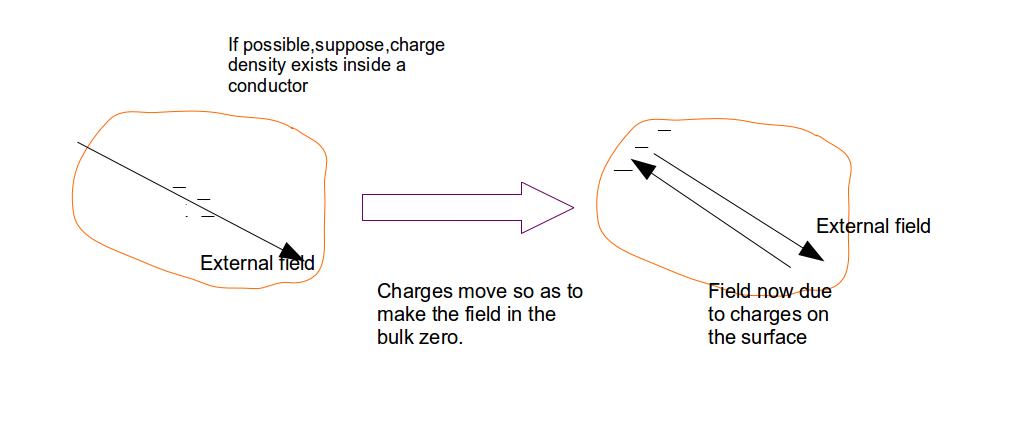
- There is no free charge in the bulk of the conductor.
- There is no net electric field at ANY point (not just the center) inside the conductor.
If there were any non-constant free charge distribution inside, the potential inside would vary from point to point. And it would be possible to lower the energy of the configuration further by transferring charge from some point at higher potential to a point at a lower potential. Since, energy COULD be lowered, this suggests that there WOULD be a tendency for doing the same: i.e. there would be a non-zero electric field at each point. Hence, this particular configuration would be prone to redistribution. Actually it is really simple, as long as there is an unbalanced force at any point of a particular charge distribution, the charge will rearrange itself. And it will continue to do so until none of the points have a finite electric field: i.e. it reaches the minimum energy configuration.