6
$\begingroup$

Let's say we've got a nice, negatively charged ring around a positively charged center disc. These objects are both 2D and reside on the same "plane".

From the side, the field and equipotential lines would look something like this, I think:

enter image description here

Now, I'm struggling to figure out how this would look from the "top". I'm thinking straight, radial field lines which point outwards from the center disc towards the ring and beyond. Conversely, the equipotential lines would just be increasingly larger circles around the center disc.

Am I thinking correctly? Is there an intuitive way of visualizing electric fields?

Improve this question
New contributor
Tsiolkovsky is a new contributor to this site. Take care in asking for clarification, commenting, and answering. Check out our Code of Conduct.
$\endgroup$
2
  • $\begingroup$ Could you clarify how these objects are oriented in space? E.g. the disk is parallel to the ring or perpendicular to it, etc $\endgroup$
    – Ruffolo
    Commented 22 hours ago
  • $\begingroup$ I edited my post. It's a simple 2D plane type of situation... Although it would be interesting to know how the other alternatives would look like too of course. $\endgroup$
    – Tsiolkovsky
    Commented 22 hours ago

1 Answer 1

Reset to default
6
$\begingroup$

Tsiolkovsky wrote: "Now, I'm struggling to figure out how this would look from the "top". I'm thinking straight, radial field lines which point outwards from the center disc towards the ring and beyond."

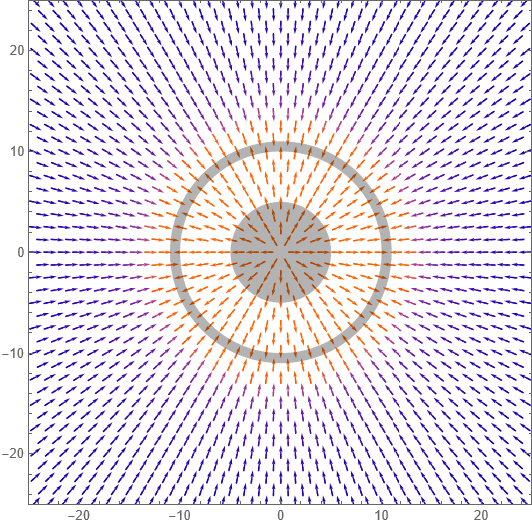
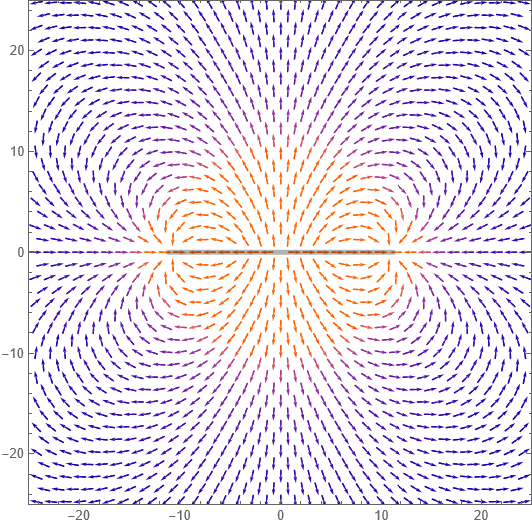
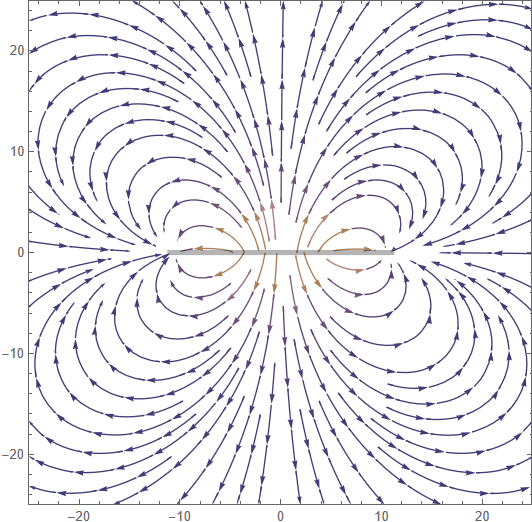
This is correct. In the first example below where the disk and the ring have opposite charges of equal magnitude the outward lines run from the disk to the ring, while the inward lines run from infinity to the ring.

Tsiolkovsky wrote: "Is there an intuitive way of visualizing electric fields?"

When viewed from the top you can draw the equatorial lines by intuition, from the side it is a little bit more complicated.

In the example below the outer ring has an inner radius of 10 and an outer radius of 11, while the inner disk has a radius of 5.

The code used is the one from here with a positive and a negative mass, which would give the same field lines like opposite electric charges. The equations in this case are elliptic integrals, they are too long to post them here but you can find them in the link above and in the references therein.

Ring and disk have opposite charges (Qʀ=-Qᴅ)

Top view of the field vectors:

enter image description here

Side view of the field vectors:

enter image description here

Side view stream plot:

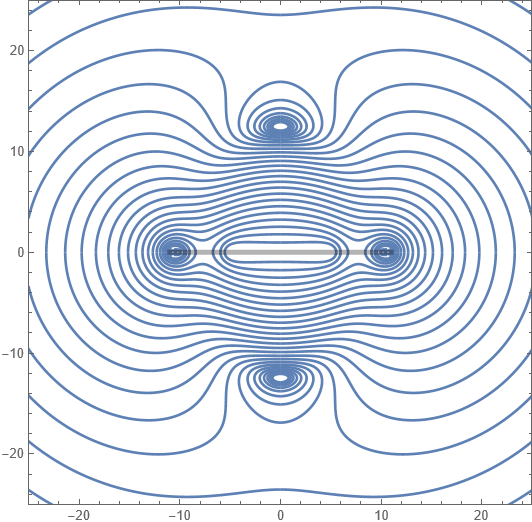
enter image description here

Side view of constant field strength contours:

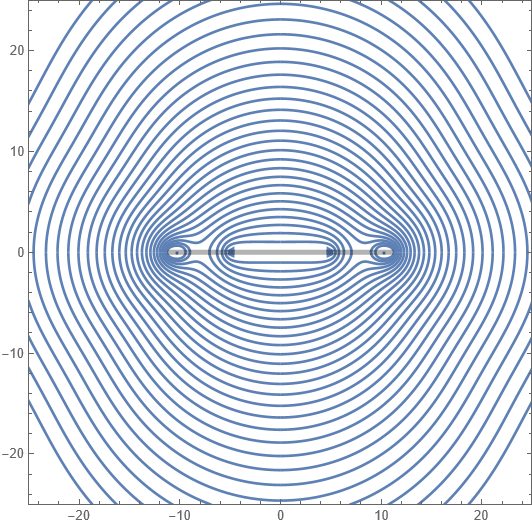
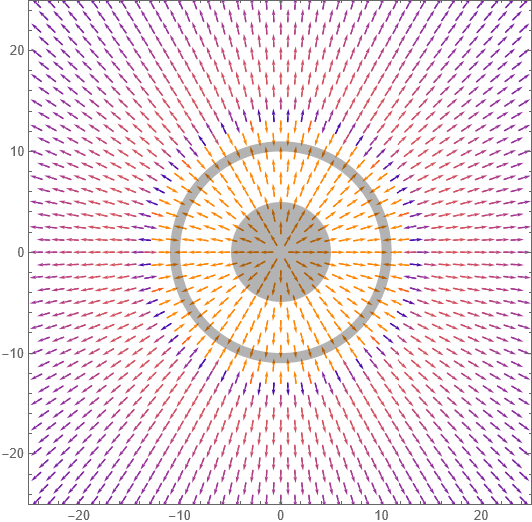
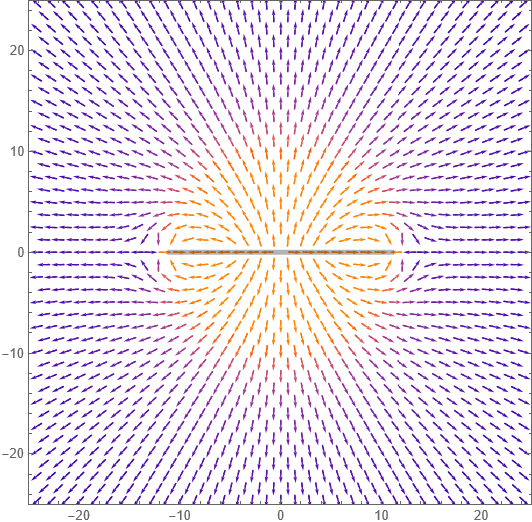
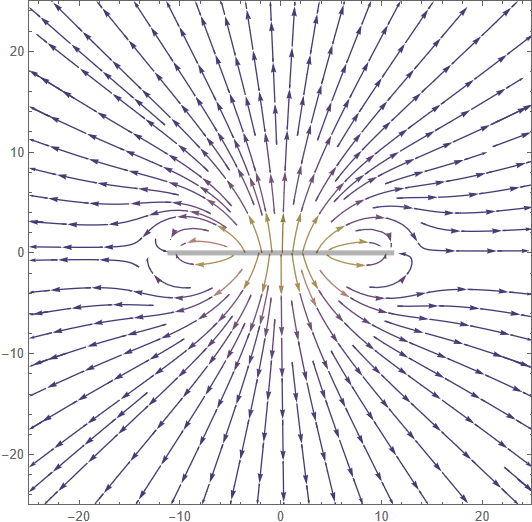
enter image description here

Ring charge is half the negative disk charge (Qʀ=-Qᴅ/2)

Top view of the field vectors:

enter image description here

Side view of the field vectors:

enter image description here

Side view stream plot:

enter image description here

Side view of constant field strength contours:

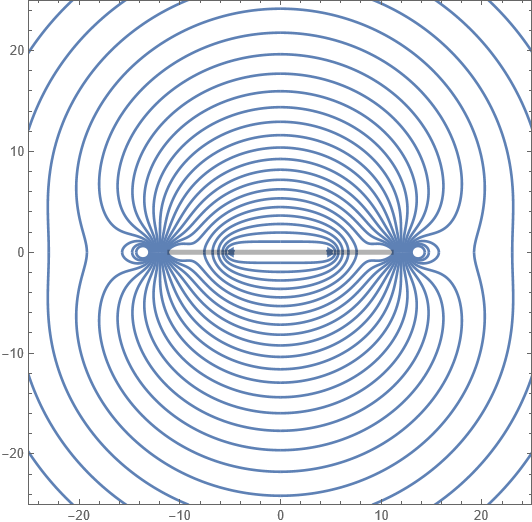
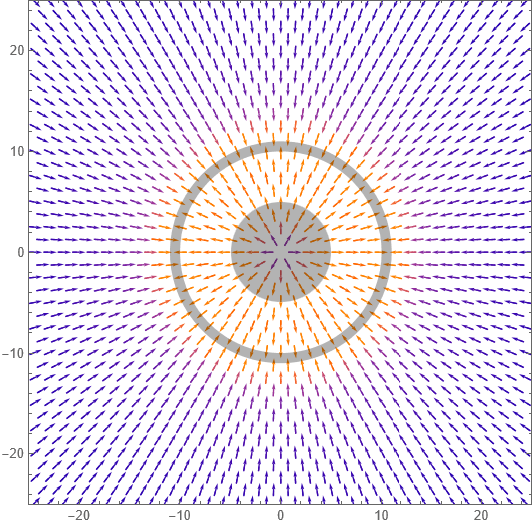
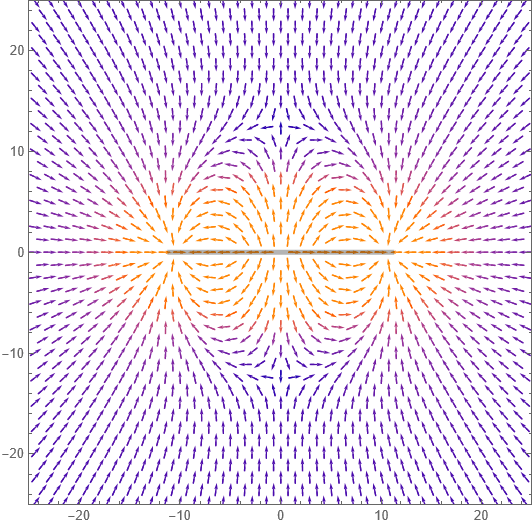
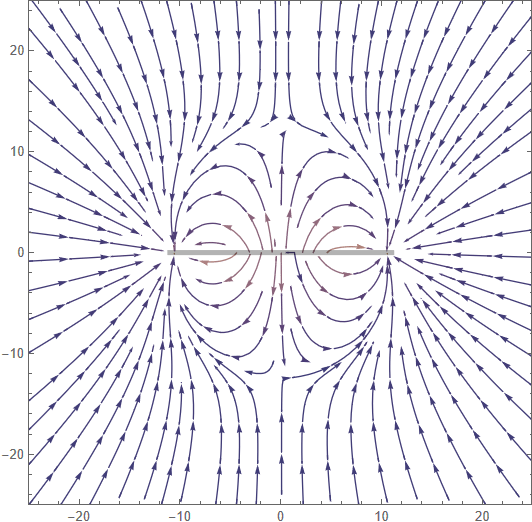
enter image description here

Ring charge is twice the negative disk charge (Qʀ=-2Qᴅ)

Top view of the field vectors:

enter image description here

Side view of the field vectors:

enter image description here

Side view stream plot:

enter image description here

Side view of constant field strength contours:

enter image description here

The code for the plots is here.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you! Just to clarify, the constant field strength contours are not the same as the equipotential lines, right? $\endgroup$
    – Tsiolkovsky
    Commented 20 hours ago
  • 2
    $\begingroup$ Correct, that is equal force, but you can find the equation for the potential in the link. Maybe I'm gonna update my site to show equipotential curves as well one day, but not today. I'll update this answer in a few days or so to show the equipotential curves, but if it's not about the pictures but about the equations you already have them. $\endgroup$
    – Yukterez
    Commented 20 hours ago

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.