Problems with equipotential lines of electric dipole with different charges

Question

First of all, sorry for the mistakes I may have in my post, I am not very good in English.

I just had an exam and a homework where I was asked the following problem and I attach my solution with my doubt about it.

---

Question

*Two point charges $-q$ and $q/2$, are located at the origin and at the point $(a,0,0)$ respectively. *

1. At what point on the $x$-axis does the electric field cancel?
2. Make a graph in the $xy$ plane of the equipotential surface passing through the point just mentioned.
3. Is this a true minimum potential point?

---

My solution

1. First i assumed $a>0$, then used the expression of the E-field, with the Griffhiths r notation, here I will use it as $\vec{\mathfrak{r}}_i=\vec{r}-\vec{r}_i '$ and $\mathfrak{r}_i=r-r_i '$ So I have:

$$\vec{E}(\vec{r})=\frac{1}{4\pi\varepsilon_0}\left(\frac{q_1}{\mathfrak{r}_1 ^2}\vec{\mathfrak{r}}_1+\frac{q_2}{\mathfrak{r}_2 ^2}\vec{\mathfrak{r}}_2\right)$$ $$\vec{E}(\vec{r})=\frac{q}{4\pi\varepsilon_0}\left(-\frac{x\hat{i}+y\hat{j}+z\hat{k}}{(x^2+y^2+z^2)^\frac{3}{2}}+\frac{(x-a)\hat{i}+y\hat{j}+z\hat{k}}{2((x-a)^2+y^2+z^2)^\frac{3}{2}}\right)$$

I chose the $x$-axis so that $y=z=0$ therefore I obtained: $$\vec{E}(x\hat{i})=\frac{q}{4\pi\varepsilon_0}\left(-\frac{x}{(x^2)^\frac{3}{2}}+\frac{(x-a)}{2((x-a)^2)^\frac{3}{2}}\right)\hat{i}$$

Here I have some doubts, in $(x^2)^\frac{3}{2}$ should I simplify as $x^3$ or as $|x|^3$?

To find the point where E-field is zero on the $x$-axis I have solved the expression and obtained:

1. My answer $$x_0=2a+ a\sqrt{2}$$

---

2. To make the graph i used the expression of potential:

$$\varphi(\vec{r})=\frac{1}{4\pi\varepsilon_0}\left(\frac{q_1}{\mathfrak{r}_1}+\frac{q_2}{\mathfrak{r}_2}\right)$$ $$\varphi(\vec{r})=\frac{q}{4\pi\varepsilon_0}\left(-\frac{1}{\mathfrak{r}_1}+\frac{1}{2\mathfrak{r}_2}\right)$$

I evaluated on $\vec{r}=x_0=a(2+\sqrt{2})$ so I got:

$$\varphi(x_0\hat{i})=\frac{q}{4\pi\varepsilon_0}\left(-\frac{1}{2a+a\sqrt{2}}+\frac{q}{2(a+a\sqrt{2})}\right)$$

Simplifying:

$$\varphi(x_0\hat{i})=\frac{q}{4\pi\varepsilon_0}\left(\frac{-3+2\sqrt{2}}{2a}\right)$$

This value is the potential at the equipotential surface. I equated it with the expression of the potential at $z=0$$. So, I have:

\begin{align} \frac{q}{4\pi\varepsilon_0}\left(\frac{-3+2\sqrt{2}}{2a}\right)=&\frac{q}{4\pi\varepsilon_0}\left(-\frac{1}{\mathfrak{r}_1}+\frac{1}{2\mathfrak{r}_2}\right)\\ \frac{-3+2\sqrt{2}}{2a}=&-\frac{1}{\sqrt{x^2+y^2}}+\frac{1}{2\sqrt{(x-a)^2+y^2}}\\ \frac{-3+2\sqrt{2}}{a}=&-\frac{2}{\sqrt{x^2+y^2}}+\frac{1}{\sqrt{(x-a)^2+y^2}}\\ \frac{2}{\sqrt{x^2+y^2}}+\frac{1}{\sqrt{(x-a)^2+y^2}}-\frac{-3+2\sqrt{2}}{a}=&0 \end{align}

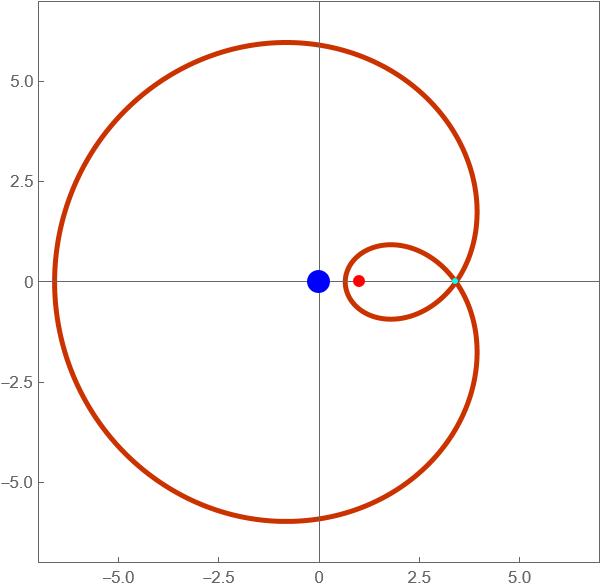
I fixed the constant $a=1$ , and i plotted this expression with Wolfram Mathematica, (I will add the code if it is requested).

I have several doubts about the graph.

1. Is it correct? I think all steps are correct, but idk.
2. If it is correct, why does it have a loop inside it?
3. What is the physical interpretation of the loop inside of it?
4. Can an equipotential line intersect with itself?
5. What would this imply in the electromagnetic field?

---

3. I used the Gradient of the potential. $$\vec{E}=-\nabla \varphi$$ As $\quad \vec{E}(x_0\hat{i})=0\quad$ then $\nabla \varphi=0$ So it is a maximum, minimum or a saddle point. But i have no idea of how to determine which one is.

---

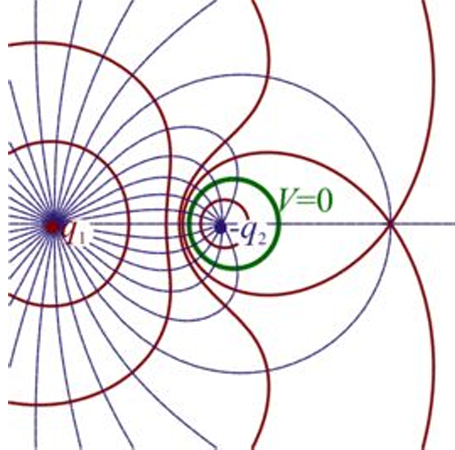
I think this is an easy problem, but it is very confusing to me because of the shape, I have searched, but I found an image with this figure on the web, so I have doubts if it is my mistake, or if it is correct.