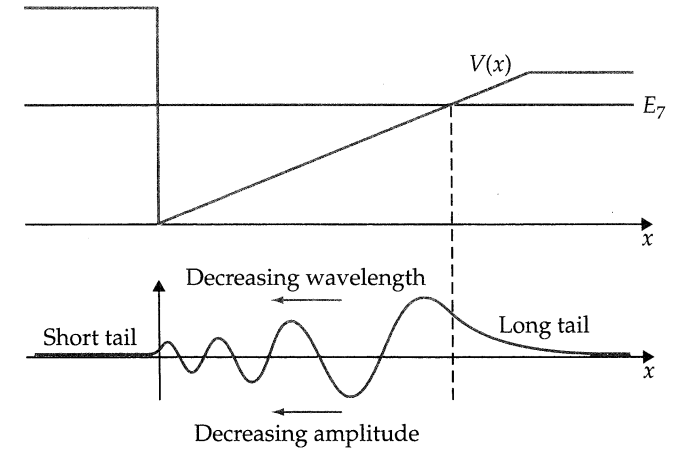
You are probably looking at an energy eigenfunction, a wavefunction which has a definite energy. The statement "$E-V$ is the kinetic energy" does not apply to a single position but to the state as a whole.
This might be a confusing statement so let's compare this to the classical case of a similar well given by
$$U(x)=\cases{\infty&$x\leq0$\\\frac{20}{50}x&$0<x\leq 50$\\20& $x<50$}$$
See picture below. I used large values for the width/depth of this well because for the quantum version some effects are shown more clearly.
Imagine you release a particle in this well and you forget about it. What is the probability (density) to find the particle at a position $x$? Well, it is proportional to the time it has spent in the region $[x,x+dx)$ so $$p(x)\propto dt=\frac{dt}{dx}dx=\frac{dx}{v(x)}$$
For a better explanation check out the first link.
Like you mentioned, you can easily calculate $v(x)$ using $$v(x)=\sqrt{2(E-U(x))/m}$$. This is shown here:
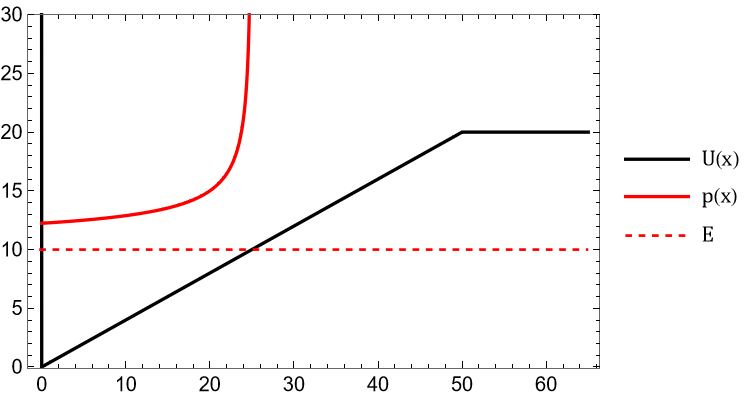
As you can see, the probability density has a sharp peak (vertical asymptote) near the turning point, so it should be of no surprise that the quantum slanted well shows higher probability near the turning point.
Let's now compare this to the quantum well.
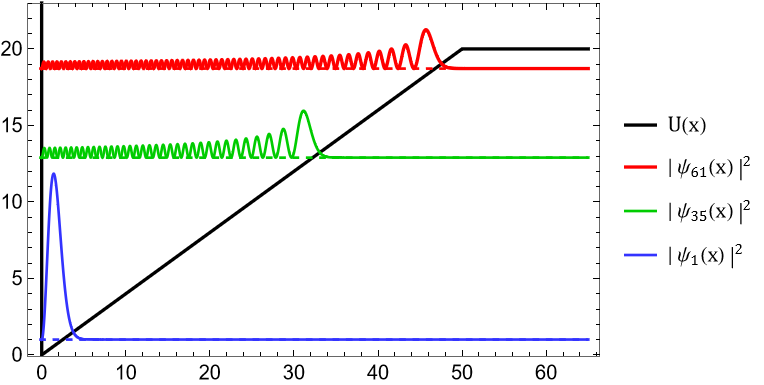
As you can see the wave functions are much better behaved compare to the classical probability function, very nice! Notice that only the ground state "minimizes" the energy. What is shown here are some of the eigenfunction which are all the possible states with definite energy. Since this is just a list there is no reason one of these states should minimize the energy. The energy eigenfunctions are special because they are the only wavefunctions whose probability amplitude is stationary in time.
A state you would find in nature would typically be many of these eigenfunctions added on top of each other, a "superposition" of different eigenfunctions. The probability density of such a state is not stationary and can slosh around in the well.