Should this object float?

Question

I was learning buoyancy on Khan Academy and it was going well until I thought of this thought experiment.

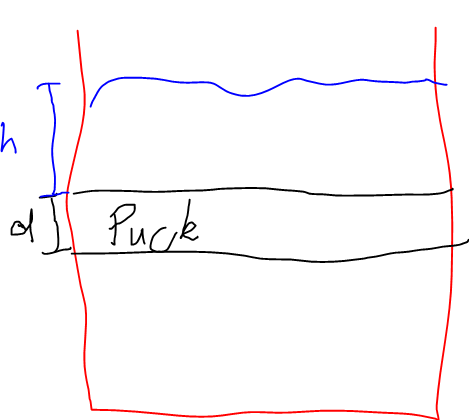
Consider if you had a tube of water with a hockey puck that completely touches the sides (but can still move freely - bit like a piston).

Normally, the argument goes: the pressure at the top is less than the pressure at the bottom because of $P = \rho gh$

Thus with the force being bigger at the bottom than the top, the hockey puck should experience a buoyancy force.

However, I think this wouldn't have to be true in this case where the hockey puck touches the sides. We derived $P = \rho gh$ from assuming that the pressure at a height, $h$, was from the weight of the water above that height.

This assumption is violated here because the hockey puck takes up a complete section of the entire pipe. This means that the total pressure at the bottom of the puck is not $=\rho g (h+d)$ but rather: $$P_{total}=P_{water} + P_{puck}$$ $$= \rho gh + \frac{m_{puck}g}{A}$$

Using $\rho g (h+d)$ assumes that the puck has the mass of water, which is not true.

Now, at the top of the puck we have $P = \rho gh$.

Thus the net pressure on the puck is $P=\rho gh + \frac{m_{puck}g}{A} - \rho gh = \frac{m_{puck}g}{A}$

That must mean that the force up on the puck at the bottom is $mg$ which only cancels out the weight of the puck. By this logic, all pucks like this should just stay static. I feel like this is not intuitively true. Where did I go wrong?

Answer 1

You have assumed that the fluid beneath the puck is exactly supporting the weight of the puck and the fluid above the puck, so of course you will find the puck to be supported.

You have assumed an incompressible fluid, so in this case the puck will not move, because if it did the fluid would have to compress as it has nowhere to go.

Answer 2

As I can make out from your questions you are assuming that water cannot pass from the sides of the puck.

In reality there are 2 cases possible.

1. If we assume that the gap between the puck and tube is water proof then your derivation is correct. All such pucks will remain in such position. To think intuitively: imagine you somehow lift the puck by a small height. Now since the water above puck cannot slide through the water proof gap, it will force the puck back into its initial position. You can't push the puck downwards (as water is almost incompressible). So the puck will remain in its position.

2. If the gap is large enough to allow water to pass, we can assume a thin ring of water around the puck. This thin ring will create the dpg pressure and thus this situation will be similar to the usual case of buoyancy.

Note: The phenomenon of buoyancy is considered when an object is immersed in a fluid. In the case described by you, the object is only sandwiched by the fluid(water in this case). The excess pressure created at the bottom of the object in the usual case of buoyancy is because the density of objects is inadequate to increase the pressure as much as the surrounding water column does. The fluids can only transmit this additional pressure if they are in contact which is why buoyancy will not be seen in the case described by you.