Why do the two balls come down at the same time?

Question

I saw this video on YouTube in which two balls slide down on different inclines.

The part which confused me was the one at 2:00 seconds. I couldn't understand the physical reason for that.

If we drop two balls from different heights they do take different times to come down. But here they are coming simultaneously.

---

One reason which I thought could be that the velocity gained by the balls is proportional to length of the incline but I am not sure and couldn't digest it .

---

A physical reason would be more appreciated than a mathematical one.

Edit : It is written in the comment section and in the answers that it is designed that way so that the balls reach the bottom at the same time.

But I still couldn't understand how does that structure of the tautochrone curve actually affect the timing? What's the physics behind that?

Answer 1

Without going into the math behind pendulums, we can still make it plausible that their periods do not depend on their amplitude. A basic feature of all harmonic pendulums is that they accelerate the swinging object with an acceleration proportional to its displacement. Meaning that if we increase initial displacement, we also get a higher initial acceleration, so the object's average speed increases. Doing the math, we will find that the average speed will be exactly proportional to the initial displacement, so no matter the displacement, one swing will take the same amount of time (four times the initial displacement divided by the average speed).

As for the incline: it has been specially constructed to make all objects take the same time, independent of initial height. Essentially, the higher you are on the incline, the steeper it is, so the higher an object starts, the higher its initial acceleration is, so like in the case of the pendulum, it gets a speed boost if it starts higher. And the speed boost exactly cancels the additional length to cover. But this only works for specially made inclines called tautochrones or isochrones, as mentioned in the comments.

Answer 2

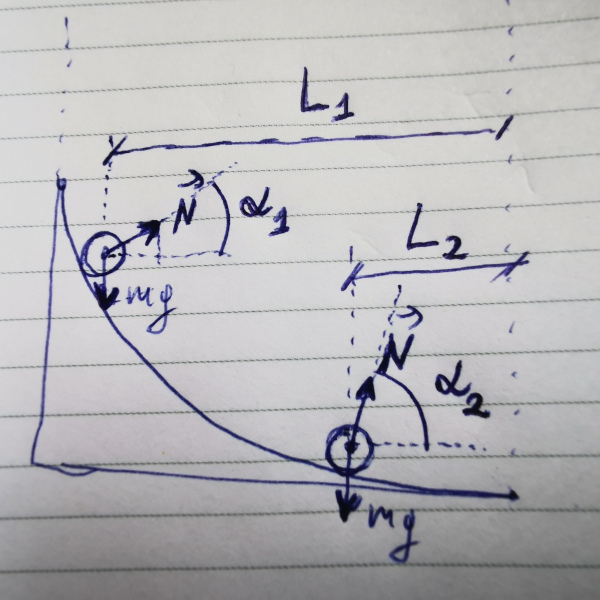
Gravity will pull on both balls the same. If there was nothing else in the way, it would cause both balls to accelerate at the same rate. However, there is something else in the system. There is the track, and it provides a "normal" force which pushes perpendicular to the track.

This normal force is partially opposing gravity. Near the bottom, where the track is nearly horizontal, this force is almost directly in the vertical plane, opposing gravity. Near the top, where the track is much steeper, the force isn't vertical. It's more at an angle.

To understand the movement, lets look at the situation in some funny coordinates known as curvilinear coordinates. Instead of making the x and y axes straight, we make it curved, following the track. Lets make the x axis be along the curved track, and the y axis be perpendicular to the curved track.

This is useful because it gets rid of the annoying changing normal force. Since the y axis is perpendicular to the track, we know that the normal force is always in this direction. Because its not in the x direction, along the track, the normal force can't add any velocity. We can ignore it. This is so helpful that it's worth the frustration of dealing with axes that are curved!

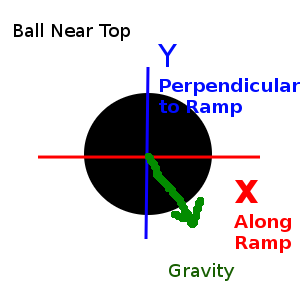
Which leaves us only with gravity. If the ball is very far up the track, where it is at a substantial angle, the force of gravity has a reasonable component in this x direction, along the path of the track. So a ball that starts on the top of the track accelerates forward quickly.

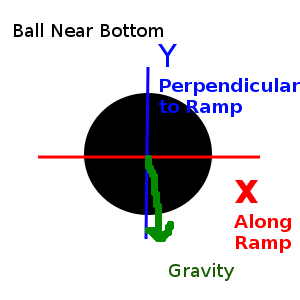
A ball that starts closer to the bottom of the track has gravity pulling almost in the y axis - almost perpendicular to the track. Since it has almost no acceleration in the x direction, along the track, it accelerates much more slowly.

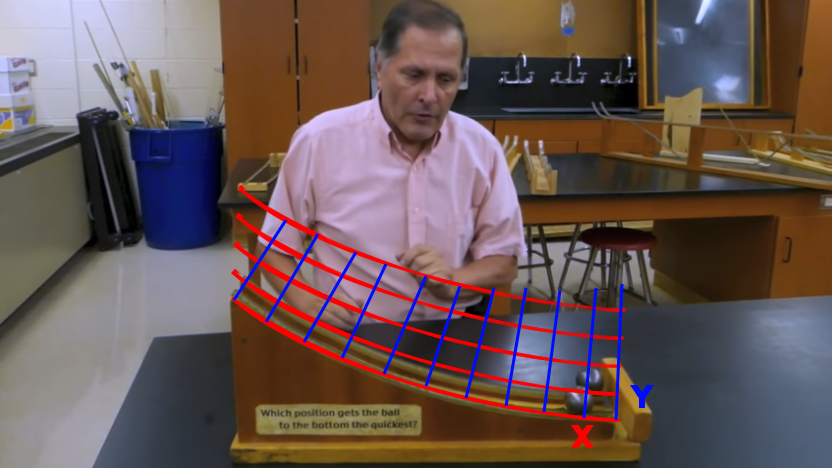
So the ball at the bottom has to accelerate slowly to reach the end. The ball near the top gets a quick burst of acceleration early on, when the track is steep. By the time it gets to the bottom, where the acceleration is smaller, it already had enough speed to blaze through.

And, as others have pointed out, the tautochrone is a curve that is carefully designed so that these effects cancel out. The higher you start the ball, the further it has to travel, but the more it can accelerate. If you start the ball low, it doesn't have to go very far, but doesn't get to accelerate very much.

Answer 3

why the two pendulums released from different angles have the same time period ?

The time period for a simple pendulum is independent of its initial conditions. $$T = 2\pi\sqrt{\frac{l}{g}}$$ where $l$ is the length of the string of the pendulum and $g$ is the acceleration due to gravity

As you can see its time period doesn't even depend on the mass or the initial angle at which it was released, provided there are some assumptions such as the angle should be small, the amplitude in terms of angle is also relatively small. In case you were wondering what does small means, it means angles less than $10^{\circ}$

If we drop two balls from different heights they do take different time to come down . But here they are coming simultaneously.

I think that might be due to friction on the surface of the incline

Answer 4

why the two balls hit the bottom at the same time

Not going into a lot of details, just notice inclination of normal force vectors :

So, $$ \frac {\alpha_2}{\alpha_1} \propto \frac {L_1}{L_2} $$

Or in words - ball at higher altitude is pushed down by a greater force, but it has to overcome a greater distance too. While bottom ball has comparatively small pushing force, but at the same time is has to travel a tiny distance too. So in the end - these balls has similar conditions of duration for reaching end wall.