I'm looking for an intuition on the relationship between time period and amplitude (for a small pertubation) of pendulums. Why does the period not depend on the amplitude? I know the math of the problem. I am looking for physical intuition.
$\begingroup$
$\endgroup$
3
-
$\begingroup$ Mathematical reason can be found Why does a simple pendulum show simple harmonic motion? $\endgroup$– YashasCommented Jul 20, 2017 at 14:40
-
15$\begingroup$ There is no intuition, since in fact the period does change with amplitude. The only intuitive thing you can say is that the change is too small to observe. $\endgroup$– Carl WitthoftCommented Jul 20, 2017 at 17:48
-
7$\begingroup$ @CarlWitthoft Obviously the OP has the harmonic oscillator model of the pendulum in mind, not the real-world pendulum. $\endgroup$– MostafaCommented Jul 20, 2017 at 19:16
Add a comment
|
9 Answers
$\begingroup$
$\endgroup$
9
The higher you are, the greater the maximum velocity and maximum potential energy.
Consider one pendulum lifted higher than a second both released at the same time. When the higher pendulum reaches the starting point of the second, it already has a velocity greater than 0.
This higher velocity allows the higher pendulum to complete its swing in the same amount of time as the lower, even though it has a longer path.
Since I'm at a computer now I will address a majority of what is said in the comments. For starters, this does not exactly apply to pendulum; only approximately, and the approximation gets worse as $\theta$ increases.
A good way to visualize this is through the Tautochrone curve which is a frictionless curve where for all heights, the time to fall is the same (this is the equivalent of a pendulum period if you ignore the backswing, or have 2 of these curves mirrored; which will be a perfect mirror of the front swing if energy is conserved in the system).
In this scenario, the accelerations work out perfectly (under the same gravity) so that they all arrive at the same time. This is unlike the circular motion, which is only approximately correct for small angles. The interesting thing to note is that looking at a small displacement of a tautochrone curve; it looks approximately circular if you only look at a small section near the bottom. This is an intuitive way to explain why a circular pendulum approximately has this behaviour with small angles.
(Henning mentioned a tautochrone curve in his answer as well. It seemed to be an appropriate way to add more intuition to this)
-
3$\begingroup$ So why in big pertubatuions this consideration doesn't work? @JMac $\endgroup$ Commented Jul 20, 2017 at 16:00
-
2$\begingroup$ And physical intuition for why his answer does not work in big perturbation? $\endgroup$ Commented Jul 20, 2017 at 16:43
-
2$\begingroup$ @NoamChai As far as I know it technically doesnt work for any perturbation perfectly. When the angle is small the periodic bevaivour appears linear (and approximately is), and this relationship only applies when it is linear. Since it is only ever approximately linear, they are only approximately the same period. $\endgroup$– JMacCommented Jul 20, 2017 at 17:06
-
2$\begingroup$ @NoamChai: It becomes more clear if we add a single word to the final sentence. The higher velocity allows the higher pendulum to complete its swing in about the same amount of time as the lower, even though it has a longer path. The reason this works is that, for small perturbations the additional velocity is almost exactly the amount needed to account for the additional distance. For large perturbations, the additional velocity is not almost exactly that amount. $\endgroup$ Commented Jul 20, 2017 at 17:40
-
6$\begingroup$ @CarlWitthoft I have elaborated a bit on that in my comment here; but "for a small perturbation" the math does state that they will be the exact same, as long as that assumption holds. In real life, the assumption never holds; but it's close enough that for practical purposes you can say it does. In real life you also never get a massless string with a point mass suspended; so it falls apart for many reasons; that we can choose to ignore depending on how you formulate the problem. $\endgroup$– JMacCommented Jul 20, 2017 at 18:37
$\begingroup$
$\endgroup$
8
Hand-wavy intuition: Suppose we don't know about pendulums but want to construct a one-dimensional path, such that a point mass constrained to this path can oscillate around a low point with different amplitudes but constant period.
We do this from the bottom up -- so imagine that we have constructed the path from an altitude of $h_1$ down to $0$ and back up to $h_1$ on the other side. Now we want to extend that up to a sligher higher altitude $h_2$.
When we release our point mass at $h_2$, we can compute what its kinetic energy (and therefore its speed) will be at any altitude, so we can (at least in principle) compute how long it takes it to pass by the already made $h_1$-to-$h_1$ segment. This will be less time than our desired period, and half of the remaining time will be how long the point mass should take to move from $h_2$ to $h_1$. If that is sufficient time (that is, more than it would take the mass to fall straight down from $h_2$ to $h_1$), we can adjust how long time it takes, simply by making the section from $h_2$ to $h_1$ a suitably inclined plane.
Taking this process to the limit (where $h_2$ is just infinitesimally higher than $h_1$) we get some horrendous continuous-delay differential equation that I don't care to either derive in detail or solve -- but Huygens did it in 1659 and found that the solution is an inverted cycloid.
So if we have a bob gliding frictionlessly along a cycloid, it will indeed have the same period for any amplitude.
A pendulum, of course, swings in a circle rather than a cycloid -- but the cycloid turns out to be smooth enough (with nonzero but finite curvature) at the bottom that it can be approximated by a circle. This approximation is good enough that the difference in period between the circle and cycloid go to $0$ as the amplitude goes to $0$.
answered Jul 20, 2017 at 19:31
-
$\begingroup$ I like your answer, I know about cycloid but I haven't thought about it before. Even if it's not a simple answer , it's pretty neat, thank's :) $\endgroup$ Commented Jul 20, 2017 at 20:14
-
$\begingroup$ @NoamChai I thought about editing in the Tautochrone curve into my answer as well. I think one of the important things to note about this is that near the end of the curve, the path looks approximately circular. That's one good semi-intuitive way to see how for small angles this applies to a pendulum. $\endgroup$– JMacCommented Jul 20, 2017 at 21:21
-
$\begingroup$ @jmac i think it's a great thing to add. And a credit for henning would be appreciated... $\endgroup$ Commented Jul 20, 2017 at 21:28
-
$\begingroup$ @NoamChai I was literally about to finish adding that to my answer anyways, for a bit more completeness. $\endgroup$– JMacCommented Jul 20, 2017 at 21:30
-
3$\begingroup$ It should perhaps be mentioned that "the cycloid can be approximated near the bottom by a circle" is not some remarkable property of cycloids and circles but rather a property of any sufficiently smooth curves with a local minimum. Near that minimum, the height function and its second derivative can be exactly matched by a circle, and the first derivative vanishes, so you can get approximation accurate up to a 3rd-order error. If the curve is left-right symmetric about the bottom point, then the error shrinks to 4th order. $\endgroup$ Commented Jul 22, 2017 at 6:06
$\begingroup$
$\endgroup$
12
For small angular displacements, the pendulum differential equation is effectively linear and as such, the amplitude of the oscillation must be independent of the period. Why?
For a linear system, if $x_1(t)$ and $x_2(t)$ are two independent solutions, then $x_3(t) = a_1x_1(t) + a_2x_2(t)$ is also a solution (superposition property).
And so, if a linearized pendulum has a sinusoidal solution $x_1(t) = \cos(\omega_0 t + \phi_0)$, then $A\cos(\omega_0 t + \phi_0)$ is also a solution by the superposition property.
answered Jul 20, 2017 at 15:57
-
3$\begingroup$ Doesn't quite satisfy the "physical intuition" aspect of the question... $\endgroup$ Commented Jul 20, 2017 at 16:16
-
2$\begingroup$ @maxathousand, it may be as close to a physical intuition as possible. The answers (including the accepted answer) that state the physical intuition is that there is higher potential energy at max displacement for larger amplitudes and thus higher kinetic energy at max velocity are wrong on my view; this intuition does not imply that the amplitude and period are independent. $\endgroup$ Commented Jul 20, 2017 at 19:05
-
$\begingroup$ OP said he understood the math. He wanted "physical intuition" on why this works. This answer is entirely related to the differential equations, not the physics. Although I agree there are plenty of nuances to this question, i believe he accepted my answer because it was a simple physical explanation for why they can have the same period. I purposefully avoided math and merely provided intuition on how it was possible from a physics school perspective. It seemed to be what the OP wanted more intuition about. $\endgroup$– JMacCommented Jul 20, 2017 at 19:53
-
$\begingroup$ @maxathousand Intuitively this translates to the fact that 1D harmonic motion (say, along the y axis) is just the image of a circular motion on the y axis. By changing the amplitude of the harmonic motion, you change the radius of the circle, but the period only depends on $\omega$. $\endgroup$– MostafaCommented Jul 20, 2017 at 19:58
-
$\begingroup$ @JMac, the fact you state in the first sentence of your answer could be true even if the period changes with amplitude. Also, it isn't true that my answer is entirely related to differential equations; the concepts of linearity and superposition are not limited to the context of differential equations. Indeed, I could remove the phrase "differential equation" from my answer as it isn't actually required. $\endgroup$ Commented Jul 20, 2017 at 20:26
$\begingroup$
$\endgroup$
0
Here's an intuitive and non-rigorous answer.
By conservation on energy you can deduce that the pendulum will be moving more slowly when it has a smaller amplitude since it has a smaller change in height and so a smaller change in kinetic energy.
With smaller amplitudes the pendulum does not have as far to travel to complete a complete swing but it is also moving more slowly. The two effects cancel each other out (at least if the amplitude of vibration isn't too large).
$\begingroup$
$\endgroup$
2
The short answer is that a periodic function still has the same period if you scale it, so a linear differential equation with solutions of a given period has such solutions at any amplitude. The angle $\theta$ of a pendulum from the vertical satisfies $\ddot{\theta}=-\frac{g}{l}\sin\theta$, but for small angles we can replace $\sin\theta$ with $\theta$, giving an approximately linear equation of motion.
-
$\begingroup$ That is decidedly not intuitive, and also circular, to a degree: The OP knows that scaling doesn't change the period (and, by inference, the mathematical solutions for such systems will of course have the same period, duh); s/he is asking why. $\endgroup$ Commented Jul 20, 2017 at 17:19
-
$\begingroup$ @PeterASchneider I disagree. I said that the scale invariance of periods implies linear differential equations' periodic solutions have amplitude-independent periods (the result to be explained). I thus provided an explanation in terms of another fact. $\endgroup$– J.G.Commented Jul 20, 2017 at 17:50
$\begingroup$
$\endgroup$
You can say it's because average speed (in the RMS sense) scales in the same way as displacement does: double the amplitude, and the RMS speed will also double. (To first-order approximation at least.)
Why would that be? Well, it's because average acceleration also scales proportionally, as that simply depends on the force. It's the particular feature of the harmonic oscillator that force depends linearly on displacement.
answered Jul 20, 2017 at 18:45
$\begingroup$
$\endgroup$
One possible approach to explain why the period does increase with initial angular starting off point is to imagine a pendulum where the string is made of taut wire. Imagine setting the initial angle at exactly $\pi$ radians. In this case the pendulum is in exact equilibrium and you may then say that the period is infinite. Now, you'd expect the relationship between the period and the starting angle to be monotonic, and since it starts off small for small angles, if the period increases from something small to infinite, at in-between starting angles you'd expect the period to get larger as the starting angle increases.
For small enough angles, this monotonic function presumably is flat, meaning that period is roughly independent of initial starting angle.
$\begingroup$
$\endgroup$
1
The larger the swing, the more vertical is the angle at which the pendulum bob starts falling at, hence the faster it accelerates at the start, and the increase in speed exactly balances the longer swing length.
Just read Enn's answer in more detail, and my answer is, more or less, a more intuitive presentation of his. The best answer is to combine his and mine: larger acceleration at start due to increased angle, and larger fall distance, hence faster movement.
-
5$\begingroup$ What you're saying is true, but it doesn't by itself give any proof at all that the period does not depend on amplitude. In fact, any convex potential function has the property that the acceleration gets ever larger as the amplitude increases, but most of these potentials do not give rise to isochronous orbits, which is what the question is about. In particular the standard circular pendulum is not isochronous, it only becomes approximately isochronous in the low-amplitude limit. $\endgroup$ Commented Jul 20, 2017 at 18:55
$\begingroup$
$\endgroup$
I wrote this answer as a response to this question but as it was marked as a duplicate I add to the answers here.
The most important thing is that the acceleration (and hence the force) is proportional to the displacement from a fixed point.
Suppose that we start with a displacement of $A$ from a fixed position with the object at rest.
Let the acceleration of the object at this position be $KA$ where $K$ is a constant which would be $\dfrac{\text{spring constant}}{\text{mass}}$ for a spring mass system or something equivalent for a simple pendulum.
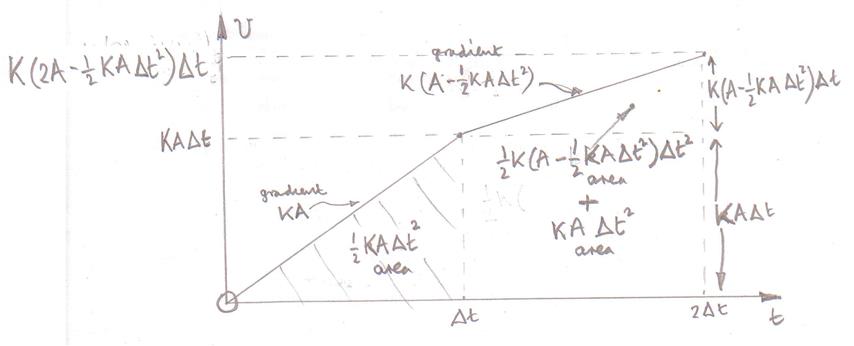
Below there is a velocity against time graph to illustrate the motion of the object from $\rm time = 0$ to $ {\rm time} = 2 \Delta t$.
In the first interval the acceleration is approximately $KA$ the change in velocity is $KA\Delta t$ and the displacement of the object is approximately $\frac 1 2 KA \Delta t^2$.
In the second interval the acceleration is approximately $K(A-\dfrac 12 KA \Delta t^2)$ the change in velocity is $K(A-\dfrac 12 KA \Delta t^2)\Delta t$ and the displacement of the object is approximately $\frac 1 2 K(3A-\frac 12 KA\Delta t^2 )\Delta t^2$.
One could continue but the key feature is already there.
Noting that $K$ is a constant of the motion, the displacement in each successive interval of time is proportional to the initial displacement $A$ which is the amplitude of the motion.
If the amplitude doubled so would the distance covered in a given time.
Thus the period of the motion does not depend on the amplitude.
This analysis shows that for the amplitude to be the common factor in successive displacements there must be a linear relationship between the acceleration and the displacement.
