0
$\begingroup$

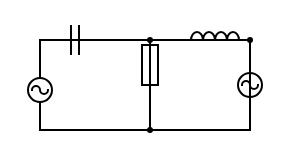
I'm having a hard time understanding why would the current flowing through the resistor in the following diagram be the sum of the currents "beside" the two AC sources.

ctri

I know the sum of the diverging currents at a node is equal to the "original" current before divergence, but I fail to see how that applies here. Does a current always diverge to every possible branch whenever it reaches a node (assuming there isn't infinite resistance there)?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

Kirchhoff's current law (KCL) simply states that the sum of currents flowing into a node must be zero. It says nothing about when one or more of these currents might be zero.

Improve this answer
$\endgroup$
3
  • $\begingroup$ What about my other questions? I can't seem to apply it here because the current of every AC source diverges to the resistor and to the other AC source. How does that work? $\endgroup$
    – Darkenin
    Commented Jun 26, 2020 at 7:55
  • $\begingroup$ I'm not sure what you mean here. There is only one current in each branch, and they add to zero where these branches join. The typical analysis involves solving for each of these branch currents. It isn't necessarily helpful to think of each branch current as a sum of the currents due to individual current sources (although this can be done and is useful in certain cases). $\endgroup$
    – Puk
    Commented Jun 26, 2020 at 8:00
  • $\begingroup$ I don't get how you deduce the result algebraically... $\endgroup$
    – Darkenin
    Commented Jun 26, 2020 at 11:37

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.