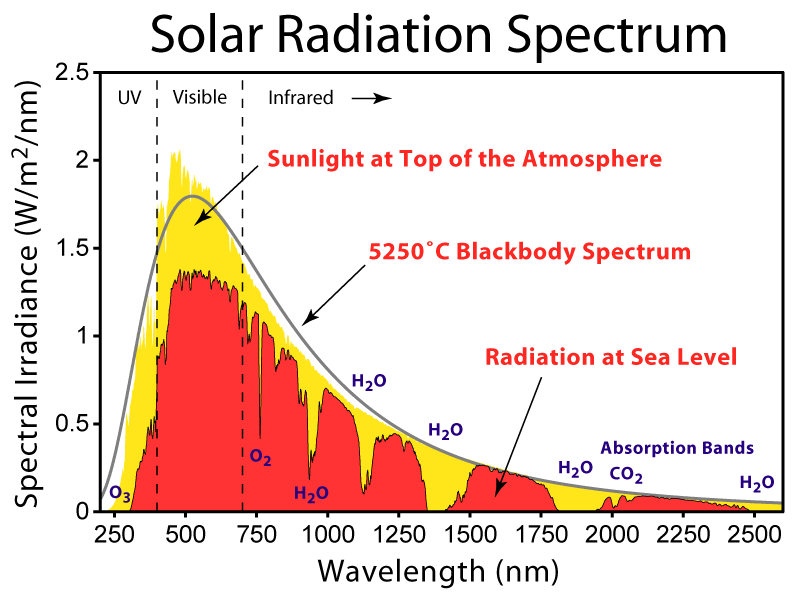
I've read a lot of answers to the questions why the sky is blue. However all the answers I found contain mostly qualitative analysis: Rayleigh scattering is changing the direction of blue light, so there is more blue light coming to the eye along the line of sight than the red one.
However these explanations raise additional questions.
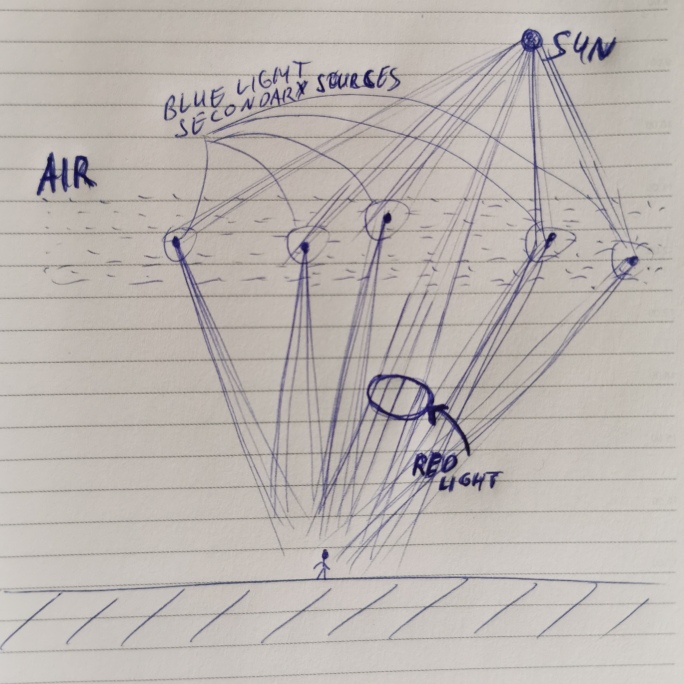
First of all, the scheme of only single scattering seems to be an oversimplification: the light direction should be changed more than once. Can we prove that this is negligible by calculation, or is it not negligible? Does this change the analysis?
Further, the explanation says nothing about the exact amount of the blue light being scattered when looking into a particular direction. Assuming the sun is in zenith, it follows from symmetry that the color of the sky in the directions having the same zenith angle must be the same, but closer to the horizon the way of the scattered light differs a lot from the rays coming near zenith — so is it possible to derive theoretically a formula which would predict the color of sky given the azimuth angle and the position of the Sun (at least in a simple geometrical setup when the Sun is in zenith)? It's not clear why the color should not rapidly change from near blue at horizon to almost red near the Sun position: after all, the atmosphere is thicker along the lines going closer to horizon! The sky seems to be more uniformly blue than the typical explanation suggests.
Further, it follows from the usual explanation that blue light is partially reflected back into the space. Due to this, about half of all scattered light should be lost, so the total amount of red light coming from sun should be greater than the amount of blue light, which seems to contradict the observable reality. Does it?
I'm mainly interested in quantitative analysis, not the observations or qualitative considerations.
I've read the answers to this question and know that the physiology of the eye comes additionally into play, but let's neglect this for the sake of simplicity.