0
$\begingroup$

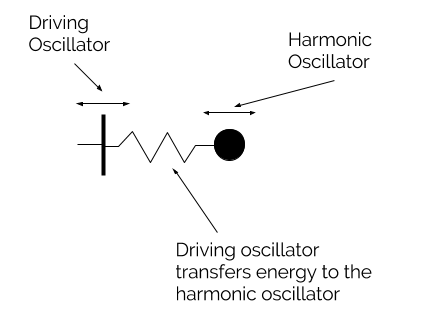
In a set up such as the following:

enter image description here

I have read in many places that below resonance the driving force is in phase with the harmonic oscillator. I have also read that the driving oscillator is in phase with the harmonic oscillator, however, I would not expect both to be true.

In a harmonic oscillator, I am under the impression that the acceleration (proportional to force) and the displacement are in antiphase. Therefore one would not expect the driving force and driving oscillator to be in phase.

I was wondering if the force acting on the driving force is not the same as the force it applies on the harmonic oscillator, and that perhaps the displacement and applied force of a driving oscillator are in fact in phase.

Is this correct, or do the two original statements, in fact, contradict each other?

Thanks.

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ The author of this answer believes it is relevant. $\endgroup$
    – rob ♦
    Commented Oct 23, 2018 at 18:56

3 Answers 3

Reset to default
2
$\begingroup$

The driveR is applying a force say $F_0 \cos \omega t$ at the end of the "spring" remote from the "mass".
That end is executing simple harmonic motion and so the displacement of the end of the spring must be proportional to the force.

If there is no damping and you do not set your initial conditions carefully you are bound to have transient behaviour superimposed on steady state behaviour.

With damping you eventually on have steady state behaviour where the amplitude of the "mass" is constant.

To figure out and possibly remember what happens as the frequency of the driveR changes consider this.

You are holding the top of a spring and there is a mass attached to the bottom of the spring.
Very, very slowly you move the top of the spring up a certain distance and then do the same with the top of the spring moving down.
What will happen to the mass at the end of the spring?
Now this is where you have to put the mass into a beaker of water if you are actually doing the experiment or assume a reasonable amount of damping so that the transients die away reasonably quickly an do not mask the steady state motion.
The mass at the end of the spring will follow almost exactly the motion of your hand.
The driveR (your hand) and the driveN (the mass at the end of the spring) move in phase with one another.

As you move your hand up and down quicker but with the same amplitude the mass will start lagging behind the motion of your hand and the amplitude of the mass will increase.
It is difficult but not impossible to show this increase in amplitude of the driveN for constant amplitude driveR as the frequency of the driveR increases.
What is easier to show and see is that at higher frequencies of the driveR the amplitude of the driven decreases to a small value and the spring looks as though at times the top and bottom of the spring is being pulled in opposite direction.
The driveR (your hand) and the driveN (mass) are almost in anti-phase.

Here is a video produced by MIT replacing your hand with a wheel the rim of which is coupled by mechanical linkages to the top of a spring which has a mass at the other end.
The advantage of producing a video is that one can wait for the transients to die away before starting to record the motion.
Notice that the mass is damped using air resistance made larger by having a large horizontal circular disc attached to it.
The video shows very clearly that although the amplitude of the driveR (rod on the right) is constant the amplitude of the driveN, which at low frequency is the sane as that of the driveR, changes as the frequency of the driveR increase going though a maximum and then dropping off towards zero.
The phase changes are also clearly to see.

Another demonstration of mechanical resonance which shows the amplitude and phase relationships all at one time is Barton's pendula (sorry about the music) where an osculating pendulum bob of large mass acts as the driveR and pendula of different length with light bobs act as the driveN systems.
You have to wait for the transients to die down.
I chose this video because it also showed the side view which makes it a lot easier to observe both the amplitude of the motions of the pendulum bobs and their relative phase.

Improve this answer
$\endgroup$
1
$\begingroup$

The answer depends on the frequency, and to some extent on the degree of damping of the system. Assuming you are talking about a lightly damped oscillator (without damping there would be no steady state), we have to look at the phase as a function of frequency.

The math is worked out on this website (or frankly, 1000's of others). The phase difference between the driving force $F = A\cos\omega t$ and the response amplitude $x = x_0 \cos(\omega t - \phi)$is given by

$$\phi = \tan^{-1}\frac{\nu\omega}{\omega_0^2-\omega^2}$$

Of course the velocity is the derivative of position, so there is a $\pi/2$ phase shift between position and velocity.

At resonance (when $\omega = \omega_0$), $\phi = -\pi/2$ and the force and velocity are in phase (this is how you get the optimal coupling of power into the oscillator). But at that point there is a phase shift between displacement and force of $\pi/2$.

Note - the acceleration of the mass is due to both the restoring force, and the driving force; you cannot simply equate "the force" with "the driving force" as that ignores the role of the spring (or other restoring force). A system without a spring has a resonant frequency of 0, and then all the above math becomes meaningless.

Improve this answer
$\endgroup$
6
  • $\begingroup$ "without damping the amplitude continues to grow so there would be no steady state" - is that true? Yes, if driven on resonance, amplitude grows linearly with time without bound, but not off resonance, correct? $\endgroup$
    – Alfred Centauri
    Commented Oct 5, 2017 at 2:22
  • $\begingroup$ @AlfredCentauri actually I believe you get a funny beating pattern when you drive an undamped oscillator off resonance. $\endgroup$
    – Floris
    Commented Oct 5, 2017 at 2:51
  • 1
    $\begingroup$ Ignoring the homogeneous solution (setting it to zero), the particular solution has an amplitude with $\omega_0^2 - \omega^2$ in the denominator which means the low frequency amplitude approaches a constant while the high frequency amplitude goes to zero. Note that as the driving frequency $\omega$ approaches the natural frequency $\omega_0$ from below, the amplitude goes to positive infinity, 'circles 'round at infinity' and comes up from negative infinity as $\omega$ increases past $\omega_0$. That is, for $\omega \ne \omega_0$, the amplitude is constant and finite. $\endgroup$
    – Alfred Centauri
    Commented Oct 5, 2017 at 3:29
  • 1
    $\begingroup$ @alfredcentauri that is true if the driving force has been applied since the beginning of time. If you start driving an undamped system at rest at t=0, your solution will not be steady state (because in effect the driving force contains all frequencies). While the "other terms" will eventually disappear in a damped system, that doesn't happen in an undamped system. That is what I meant by "no steady state". $\endgroup$
    – Floris
    Commented Oct 5, 2017 at 3:49
  • $\begingroup$ @Floris I think you'd need to double-check the math here, DSolve[x''[t] + w0^2 x[t] == Cos[w t] && x[0] == 0 && x'[0] == 0, x[t], t] - Mathematica seems to think otherwise. $\endgroup$
    – LLlAMnYP
    Commented Oct 5, 2017 at 9:27
1
$\begingroup$

I have read in many places that below resonance the driving force is in phase with the harmonic oscillator. I have also read that the driving oscillator is in phase with the harmonic oscillator, however, I would not expect both to be true.

Back to basics:

Let the position of the 'wall' be given by

$$x_w(t) = X_{max}\cos\omega t$$

The force on the mass is then

$$F_m(t) = m\ddot x_m = -k[(x_m(t) - x_0) - x_w(t)]$$

where $x_0$ is the relaxed length of the spring. The differential equation is then

$$\ddot x_m + \frac{k}{m}x_m = \frac{k}{m}[x_0 + x_w(t)]$$

The transfer function, $\frac{X_m(\omega)}{X_w(\omega)}$, is then (for $\omega > 0$)

$$\frac{X_m(\omega)}{X_w(\omega)} = \frac{\omega^2_0}{\omega^2_0 - \omega^2},\qquad \omega_0 \equiv \sqrt{\frac{k}{m}}$$

It is easy to see that the wall and mass displacements are in phase for $\omega < \omega_0$ and in anti-phase for $\omega > \omega_0$.

$$x_m(t) = \frac{\omega^2_0}{\omega^2_0 - \omega^2}X_{max}\cos\omega t$$

If the 'driving force' is understood as the forcing function on the right hand side of the differential equation then, being proportional to $x_w(t)$, it too is in phase for $\omega < \omega_0$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.