2
$\begingroup$

$\angle I +\angle E=\angle A+\angle D$

Angle of incidence + angle of emergence = angle of prism (Normally $60^\circ$) + angle of deviation.

If their sum is not equal,we made personal error in doing an experiment with prism. Please make sense of this equation.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
6
$\begingroup$

The following diagram shows the prism with the incoming and outgoing light rays.

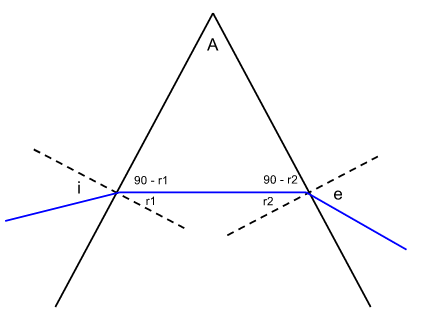
Prism

If you follow the incident light ray in, it gets bent by an angle $\theta_1 = i- r_1$. If you follow the light ray where it leaves the glass, it gets bent again by an angle $\theta_2 = e - r_2$, so the total deviation is:

$$ \begin{align} D &= \theta_1 + \theta_2 \\ &= i + e - (r_1 + r_2) \end{align} $$

For the next step look at the triangle formed by the top of the prism and the light ray, and note that the internal angles must add up to 180°. So:

$$ A + (90 - r_1) + (90 - r_2) = 180 $$

and a quick rearrangement gives:

$$ A = r_1 + r_2 $$

Now substitute for $r_1 + r_2$ in our first equation and we get:

$$ D = i + e - A $$

or:

$$ D + A = i + e $$

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Thanks, though sadly I can't claim this is an original answer as you'll come across it in any introductory optics course. $\endgroup$
    – John Rennie
    Commented Mar 12, 2013 at 11:54
0
$\begingroup$

Anenter image description here Please check this answer it is simpler one and easy to understand😀😀

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Hi and welcome to Physics SE. Please, read that we don't like images for formula. Images should only be destined to diagrams and phootographs, but not text. Among the main reasons: some people can't see them properly, and the searcher cannot index the formuals if they're on a picture. Please, type the formulae manually. $\endgroup$
    – FGSUZ
    Commented Dec 2, 2018 at 18:21
  • 1
    $\begingroup$ Welcome to Physics! Please do not post images of texts you want to quote, but type it out instead so it is readable for all users and so that it can be indexed by search engines. For formulae, use MathJax instead. $\endgroup$
    – Kyle Kanos
    Commented Dec 2, 2018 at 18:22
  • $\begingroup$ To reinforce the previous comments: your answer may be correct but it is not useful in its current format and as a result is unlikely to attract much attention. Please take a few moments to go through the basic MathJax tutorial: math.meta.stackexchange.com/questions/5020/… $\endgroup$
    – ZeroTheHero
    Commented Dec 2, 2018 at 19:21

Not the answer you're looking for? Browse other questions tagged or ask your own question.