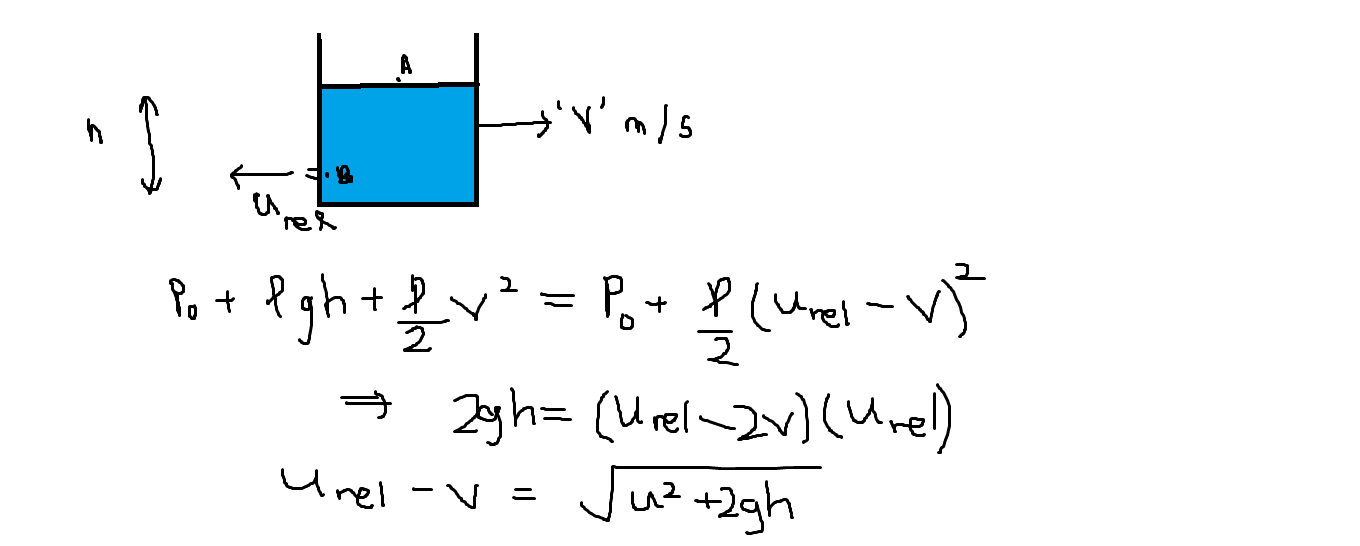0
$\begingroup$

enter image description hereSuppose there is a big tank of water with a small hole near the bottom. Now if this tank starts moving with say a velocity 'v', then will Toricelli's law hold? I tried directly applying Bernoulli's equation taking into account the velocity of the container, which gives me a result of absolute velocity of efflux as square root of $(u^2 + 2gh)$. But can we use the Bernoulli's law here?

Also another question - Now what will be the force exerted by the ejected fluid on the tank?

Improve this question
$\endgroup$
12
  • 1
    $\begingroup$ Show us how you came up with that result. A tank moving horizontally at a constant velocity v is an inertial frame of reference, and the laws of physics do not change between inertial frames of reference. $\endgroup$
    – Chet Miller
    Commented Mar 18, 2016 at 16:21
  • 1
    $\begingroup$ yes! if air resistance is neglected then you will see the water coming out will also move with velocity $v$ in horiz. direction and it will be stationary in horiz. direction w.r.t container! so happily apply bernoulli's law $\endgroup$
    – user5954246
    Commented Mar 18, 2016 at 16:27
  • 1
    $\begingroup$ @Shodai: Chester Miller is 100 % correct. Carry out this experiment on a train moving at constant speed and the result is the same as carrying it out on the passenger platform (stationary). Things would be different if there was acceleration by the train going on but that is a different question from yours. $\endgroup$
    – Gert
    Commented Mar 18, 2016 at 17:31
  • 1
    $\begingroup$ As reckoned from a stationary frame of reference relative to the moving tank, the fluid flow is non-steady-state. So to apply the Bernoulli equation to this situation, a non-steady-state version of the Bernoulli equation must be used. I haven't figured out how to do this yet. From a frame of reference of an observer moving with the tank, the usual steady state version can be used, and the Toricelli law is obtained. $\endgroup$
    – Chet Miller
    Commented Mar 19, 2016 at 1:17
  • 1
    $\begingroup$ @Shodai As I said in my previous answer, you can't use the standard bernoulli equation to get the correct result for the moving train (if you do the analysis from a fixed from of reference). You need to use a modified version of the Bernoulli equation that takes into account the non-steady nature of the flow as reckoned from the stationary frame. If you use the modified version, you will get the same answer. Google "Transient Bernoulli Equation." $\endgroup$
    – Chet Miller
    Commented Mar 19, 2016 at 11:52

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.