No.
The question is based on a false assumption.
Nothing "seeks ground" in the sense the expression implies.
Current flows in an electrical circuit. If part of the circuit is connected to "ground" then current will flow into or out of ground as part of flowing in the circuit as a whole. If you look at the "circuit diagrams" of electric circuits, in many cases the connection between two points in the circuit, which are usually made by use of a wire could instead by passing the current through "ground". Passing current into And out of ground involves a degree of electrical "resistance" to current flow - but this resistance can be relatively low if enough effort, material and money is expended.
This effect is used to produce "SWER" (single wire earth return) power systems which use a two "conductor" circuit with one conductor being a wire and the other wire is "ground". These circuits have enough technical problems that they are not used in most applications but are still in use in many countries to provide power feed to small remote loads in rural areas.
Wikipedia Single Wire Earth Return powerlines
SWER circuit using ground as one conductor of the two conductor power circuit
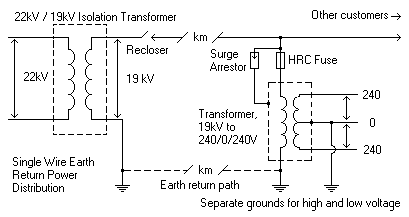
Many SWER examples
Example of electricity NOT seeking ground:
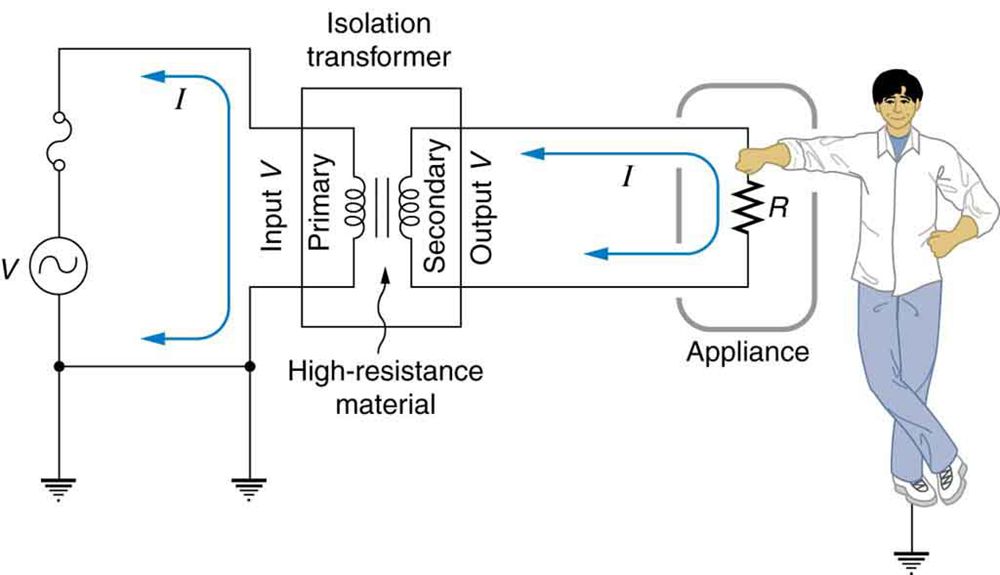
In the diagram below which is figure 8 on this page the secondary circuit is isolated from ground - with energy being transferred from input to output by the isolating transformer's magnetic field - and the person shown does not experience electric shock.
SWER line:
One aerial wire feeds a pole mounted transformer. A ground wire runs from the transformer, down the pole to a suitable "earth connection"

________________________________
Matter does not "seek ground" in the sense generally intended by the expression.
If the term "seeks" is used to refer to "gravitational attraction" then all matter seeks all other matter - with a force proportional to the product of their masses and the inverse square of their distance $d$ apart. i.e. $$F= \frac{k m_1 m_2}{d^2}$$
Where $k$ is a constant used to provide the correct magnitude result in the units system used.
In the case of e.g. a thrown rock and our planet - the rock seeks the planet AND the planet seeks the rock. BOTH move towards each other. However, as both experience the same force and as acceleration is proportional to the inverse of mass, the planet does not move "very much at all" before the rock reaches it. If you place two identical rocks (spherical and symmetrical to avoid complications) in space a long way away from any other mass and both stationary relative to some frame of reference then both will seek each other equally, both will accelerate towards the other with equal acceleration and the velocity of each relative to the frame of reference will be equal and opposite. This applies regardless of whether the "rocks' are say 1 kg in mass or say 1,000,000 kg in mass, or planet sized (ignoring aspects such as strength of materials which will cause disintegration at planet scales as they come within a certain distance (known as the Roche limit). In the case of planet sized rocks both could be considered to be "ground" and both would equally "seek" each other.
Note that mechanical vibration and electromagnetic energy are not equivalent and that the reconciliation of gravity and electromagnetic force eluded Einstein and everyone else since.



