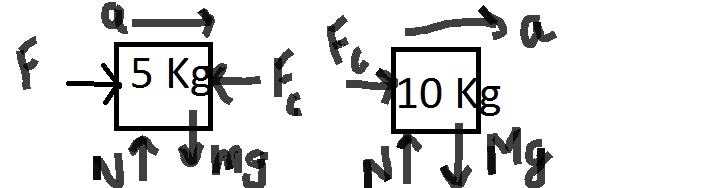1
$\begingroup$

enter image description here

A $5$ kg and a $10$ kg box are touching each other. A $45N$ horizontal force is applied to the $5$kg box in order to accelerate both boxes across the floor. Ignore friction forces and determine the force between the hand and the boxes.

Considering the body as a single mass, we can find the acceleration of the body as $3\ \mathrm{ms}^{-2}$. The force between the blocks can similarly be found as $30\ \mathrm{N}$. But coming to the question, I cannot be sure as to whether to take the two blocks as a single body. If I do so, then as per my understanding of Newton's Third Law, the hand should experience a force of $-45\ \mathrm{N}$. Now, despite my hand experiencing an equal force in the opposite direction, I am able to keep contact with the block and continue pushing the 2 blocks over a distance (say $d$). Could someone please help me by explaining why this is so? Thanks a lot!

(This is not an original question. I had come across it 'the Physics Classroom' and thought of this variant.)

Improve this question
$\endgroup$
10
  • $\begingroup$ What would be the problem with having a negative force? You say you exert a force of $45N$ on the box so surely you would expect by $N3L$ that the box would exert the same force in the opposite direction onto your hand? $\endgroup$
    – tey yreryt
    Commented May 1, 2015 at 15:43
  • $\begingroup$ Sorry, I somehow forgot to add a few lines to the question. I'm editing it now. Please do help. $\endgroup$
    – WORLD 1
    Commented May 1, 2015 at 15:46
  • $\begingroup$ @teyyreyt Is it alright now? $\endgroup$
    – WORLD 1
    Commented May 1, 2015 at 15:50
  • 1
    $\begingroup$ Possible duplicates: physics.stackexchange.com/q/45653/2451 and links therein. $\endgroup$
    – Qmechanic ♦
    Commented Apr 14, 2017 at 20:43
  • 1
    $\begingroup$ Does this answer your question? Given Newton's third law, why are things capable of moving? $\endgroup$
    – Brian Drake
    Commented Nov 15, 2020 at 13:11

2 Answers 2

Reset to default
1
$\begingroup$

First We'll see the FBD (Free Body Diagram)and we get: enter image description here

Where Fc is normal force acting (Force of contact).

From FBD of 5 Kg block (By newton's 2nd law)

$$F-F_c = ma$$ (1)

From FBD of 10 Kg block

$$F_c=Ma$$

Solving above equations we will get:

$$F=ma+Ma$$ $$a=F/(m+M)$$

Putting the values you may get your result and your resultant force.

Improve this answer
$\endgroup$
2
  • $\begingroup$ $F-F_c = ma$ .$F_c=Ma$. From these, shouldn't we get $F=ma+Ma$? $\endgroup$
    – WORLD 1
    Commented May 1, 2015 at 16:05
  • $\begingroup$ @WORLD1 You are right ... Just get it little bit wrong :P i have edited $\endgroup$
    – Shashank
    Commented May 1, 2015 at 16:12
0
$\begingroup$

The net force on the 5kg block will be 15N in the direction of the hand pushing. the subsequent acceleration or the 5kg block will be 3m per second per second. the net force on the 10kg block will be 30N giving an acceleration 3m per second per second. The accelleration will continue until the the hand is removed resulting in the blocks continuing at a constant velocity until a further force is actioned on the boxes.

Improve this answer
$\endgroup$
7
  • $\begingroup$ But why should the hand be able to keep on moving in contact with the blocks? Shouldn't Newton's Third Law prevent it from doing so? $\endgroup$
    – WORLD 1
    Commented May 1, 2015 at 16:29
  • $\begingroup$ I Think yes the hand does experience a force of 45N in the opposite direction meaning the relative velocity of the hand and the boxes remains zero. But the hand has to accellerate along with the boxes in order to maintain the zero relative velocity between them. $\endgroup$
    – 8Mad0Manc8
    Commented May 1, 2015 at 16:46
  • $\begingroup$ Could you please explain how the relative velocity between the hand and the boxes remains $0$? Thanks a lot in advance! $\endgroup$
    – WORLD 1
    Commented May 1, 2015 at 17:03
  • $\begingroup$ If the hand and the box are accelerating at the same amount and in the same direction the relative velocity between the two is zero ie they are travelling at the same velocity in the same direction at any instant in time thus the relative velocity between them is zero. Iam still thinking about your question though. $\endgroup$
    – 8Mad0Manc8
    Commented May 1, 2015 at 17:08
  • $\begingroup$ I must admit newtons third law states equal and opposite thus if a 45N force is exerted in one direction the same must be exerted in the other but if this was the case every force would result in no change in an objects acceleration. $\endgroup$
    – 8Mad0Manc8
    Commented May 1, 2015 at 17:35

Not the answer you're looking for? Browse other questions tagged or ask your own question.