Obviously this is impossible in relativity; however, if we ignore relativity and use only Newtonian mechanics, is this possible? How (or why not)?
$\begingroup$
$\endgroup$
5
asked Aug 4, 2011 at 17:02
BlueRaja - Danny PflughoeftBlueRaja - Danny Pflughoeft
2,8783 gold badges29 silver badges29 bronze badges
-
2$\begingroup$ The question as posed is trivial. If the force as a function of time F(t) integrates to infinity, then of course the answer is yes. $\endgroup$– user4552Commented Aug 5, 2011 at 2:45
-
$\begingroup$ @Ben Crowell, what is infinite speed? It's nonsense... $\endgroup$– kuzandCommented Aug 5, 2011 at 6:20
-
$\begingroup$ Vector quantities like velocity etc. cannot be infinite. This is meaningless. You should reformulate your question (the title). $\endgroup$– kuzandCommented Aug 5, 2011 at 16:56
-
$\begingroup$ @ANKU: It's not meaningless - please see BebopButUnsteady's answer $\endgroup$– BlueRaja - Danny PflughoeftCommented Aug 5, 2011 at 17:23
-
$\begingroup$ @ANKU One comment about one topic is enough. $\endgroup$– user68Commented Aug 5, 2011 at 20:20
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
8
The answer is yes in some unintersting senses: Take two gravitational attracting point particles and set them at rest. They will attract each other and their velocity will go to $\infty$ in finite time. Note this doesn't contradict conservation of energy since the gravitational potential energy is proportional to $-1/r$. This isn't so interesting since it's just telling you that things under gravity collide. But its technically important in dealing with the problem of gravitationally attracting bodies.
Now a more intersting question: Is there a situation where the speed of a particle goes to infinity without it just being a collision of two bodies?
Suprisingly, the answer to this question is yes, even in a very natural setting. The great example is given Xia in 1995 (Z. Xia, “The Existence of Noncollision Singularities in Newtonian Systems,” Annals Math. 135, 411-468, 1992). His example is five bodies gravitational interacting. With the right initial conditions one of the bodies can be made to oscillate faster with the frequency and amplitude going to infinity in finite amount of time.
Added
Here is an image. The four masses $M$ are paired into two binary systems which rotate in opposite directions. The little mass $m$ oscillates up and down faster. It's behavior becomes singular in finite time.
answered Aug 4, 2011 at 18:04
-
-
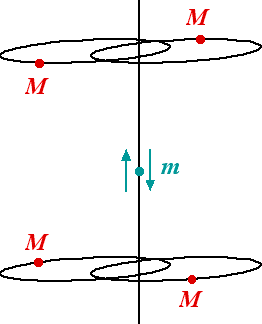
$\begingroup$ We can tell the same for atracting point charges. As the distance r decreases the force between them increases. Taking limit $r\to 0$ you get $F\to \infty$, therefore $u\to \infty$. But this means that the speed aproaches the infinity, not that the velocity is infinite. Take a look at definition of the limit. $\endgroup$– kuzandCommented Aug 4, 2011 at 19:07
-
$\begingroup$ I'm having trouble understanding your diagram. I'm fine with the fact that $m$ is a comparatively small mass, but where does it go when it oscillates to infinite speeds? Does it get out of the gravity well eventually? And how do the 2 binary systems maintain the distance from each other? Is it supposed to be implied that they're rotating, or that they're oscillating as well? $\endgroup$ Commented Aug 4, 2011 at 20:32
-
2$\begingroup$ @AlanRominger: I know this is a very old discussion, but since nobody's responded to it: the trick in the paper is that the orbital periods are tuned so that each time the small body encounters one of the binary systems of large bodies, it steals a little bit of energy from the binary, thus pulling the bodies in the binary closer together (and so reducing their mutual potential energy) while getting flung back with more velocity (and thus more kinetic energy) than it had before. Of course, since momentum is conserved, each encounter also pushes the two binaries further away from each other. $\endgroup$ Commented Apr 22, 2020 at 18:22
-
2$\begingroup$ … Also, since pulling the binary closer together reduces its orbital period, it's possible to arrange the system so that a suitable encounter is always possible even though the smaller body keeps bouncing more and more frequently between the two binaries. In the limit, the semi-major axis of each binary tends to zero (which, for pointlike bodies under Newton's laws, makes their potential energy tend to minus infinity) while each binary is pushed away from the other at a velocity that tends to infinity as a result of ever more frequent encounters with the small body shuttling between them. $\endgroup$ Commented Apr 22, 2020 at 18:28
$\begingroup$
$\endgroup$
2
If by "ignoring relativity", you mean ignoring the fact that nothing can move faster than the speed of light, then the answer is still no.
Since kinetic energy is proportional to the square of the speed, infinite speed would mean infinite energy, which you cannot provide, whatever the amount of time you are considering.
-
$\begingroup$ I'm sure the quest to accelerate to infinity will be easier due to the numerous physically implausible inventions that are possible in this world without relativity :-P $\endgroup$ Commented Aug 4, 2011 at 18:52
-
3$\begingroup$ Others have provided simple counterexamples to your statement. $\endgroup$– user4552Commented Aug 5, 2011 at 2:36
$\begingroup$
$\endgroup$
5
Lab Reference Frame
Let's start assuming a force is exerted on the object from equipment "lab" reference frame, meaning that there is negligible recoil on the lab frame from accelerating the object. Not only is it the case that $F=ma$, but the power delivered to the object grows as $P=v F$. Take a device like the large hadron collider, and just completely wave off the technical difficulty of applying force to an object of progressively increasing velocity, and let's not the following.
$$v' = a = \frac{F}{m} = \frac{P}{v m}$$
The requirement is that $v \rightarrow \infty$ in a finite time. You know, just for fun let's use an actual functional form.
$$v(t) = -\frac{1}{t}$$
For t from $-\infty$ to $t=0$.
$$P = m v' v = -\frac{m}{t^3}$$
So the power delivered must increase at the hilariously fast rate of $1/t^3$ as $t$ goes to zero, not that it matters because we all knew this would result in requiring infinite energy, but this just shows exactly how intangible it is.
Rocket
In the case of a rocket the propellant has to be hauled along with the payload, but the tradeoff is that you then don't have the $P=v F$ proportionality, since the propellant itself is still moving after being ejected. The equation of motion for a rocket that starts with mass $M$ and ends with mass $m$, ejects propellant with speed $v_e$ is as follows. I'll add in the approximation for $M \gg m$, because obviously that must be the case since we're talking about going to infinity.
$$v = v_e \ln{ \frac{M}{m} } $$
This is certainly interesting. It is interesting to note that the speed the rocket can reach is proportional to the speed the propellant is ejected at, which is also not limited by the speed of light. The energy required to expel that propellant also isn't a problem (haha) because it is not the case that $E=mc^2$ in this world. But it would be skirting the problem to say that the propellant is ejected at infinite speed, so we still seek a way for the above expression to limit to infinity with $v_e \neq \infty$. But we would also really like $\frac{M}{m} \neq \infty$, because that would require either an infinitely large starting mass or an infinitely small ending mass. Neither of these options are appealing, unless atoms also don't exist in this world, allowing us to use fractal math to claim that an infinitely small chuck of something went to infinite speed.
To the extent that we don't make these absurd assumptions, your request is impossible, even given the already absurd assumption of allowable superluminal speed.
answered Aug 4, 2011 at 18:12
-
1$\begingroup$ "Let's start assuming a large reference frame acts on the object." What does this mean? A frame of reference is not an object that exerts forces on other objects. $\endgroup$– user4552Commented Aug 5, 2011 at 2:37
-
$\begingroup$ @Ben Edited the answer for clarity. $\endgroup$ Commented Aug 5, 2011 at 2:48
-
$\begingroup$ Will somebody PLEASE explain the downvotes, because as far as I can tell this is a useful and thoughtful answer. If there is something that you know that I don't, of course I want to hear it. $\endgroup$ Commented Aug 5, 2011 at 12:49
-
1$\begingroup$ Downvoted because it's too limited in imagination --- as the top comment makes clear. There seems to be a great deal of "classical" work that people are in the dark about. You've done a great job of explaining a particular situation, but clearly failed to account for the full general case. $\endgroup$– gennethCommented Aug 6, 2011 at 16:01
-
$\begingroup$ @genneth That's fair. I would just add that the case of two passing charges fully satisfies the requirement of delivering the rate of power specified, that being the $1/t^3$ form I gave. It's interesting to put things in these terms, because it shows that no answer can really be "right". Since it's a made-up world, quantum mechanics could prohibit the flyby that leads to infinite velocity... or it could not. $\endgroup$ Commented Aug 6, 2011 at 22:45
$\begingroup$
$\endgroup$
It sounds like the answer is yes for particles with mass but zero physical extent. I assume if the particles have finite extent (or we bring in quantum mechanics), then the answer is no, as it would be if we brought in relativity.
