I'll try to point by point address the question.
So we have $E(\omega)$ the energy radiated at a given frequency.
Actually, no, we have an energy per unit frequency. It's like a density. For a mass density $\rho$ you have a mass per unit volume, and the density can vary from place to place, so to get the total mass you break up space into a bunch of little volumes, each of which has density constant to within some tolerance, then you take that density and that volume and multiply them together to get the mass of that piece, and add up all the pieces, or $m=\iiint\rho dx dy dz$.
Similarly you have energy $U$ and energy density $u$. But they come in multiple kinds, you can have an energy per unit wavelength $u_\lambda$ or an energy per unit frequency $u_\nu$, and these two different energy densities (per wavelength and per frequency) don't even have the same units. For an energy per wavelength $u_\lambda$, you take all the possible wavelengths, chop the interval into pieces, each of which the density $u_\lambda$ doesn't change much and multiply the density $u_\lambda$ by the interval of wavelengths in the pieces $\Delta \lambda$ to get $u_\lambda \Delta \lambda$ the total energy $dU$ in that piece, then you add that up for every piece of the total interval to get the total energy:
$$U=\int_0^\infty u_\lambda d\lambda.$$
Similarly for frequency, you take the total frequency range $(0,\infty)$, chop the interval into pieces, each of which the density $u_\nu$ doesn't change much and multiply the density $u_\nu$ by the interval of frequencies in the piece $\Delta \nu$ to get $u_\nu \Delta \nu$ the total energy in that piece, then you add that up for every piece $dU$ of the total interval to get the total energy:
$$U=\int_0^\infty u_\nu d\nu.$$
You could even do this in reverse. Take the interval of all frequencies and break it into pieces, then for each piece take the total energy in that piece $dU$, and divide it by the size of the piece $\Delta \nu$ to get $dU/\Delta\nu$, and notice that when $\Delta \nu$ is small enough, the quotient $dU/\Delta \nu$ doesn't change anymore ($dU$ gets smaller too when you consider a smaller range of frequencies). That quotient is the energy density $u_\nu$.
If you do this, you see that for any piece of the total interval, there is a real amount of energy in the interval, $dU$, and that there is a real lower frequency(high wavelength) and a real higher frequency (low wavelength) and everything you think is fine. The only difference is the length of the interval of wavelengths is different than the length of the interval of frequencies.
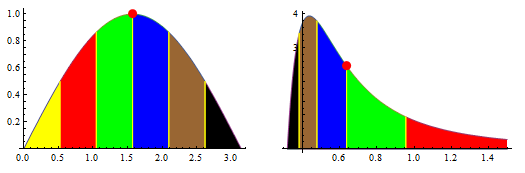
For instance if you went from 1Hz to 2Hz in frequency, then $\Delta \nu=$2Hz-1Hz=1Hz, so you get $u_\nu = dU/\Delta \nu=dU/$1Hz. For the exact same interval of frequencies there is an interval of wavelengths that covers the same piece of the total. Since $\lambda \nu=c$ (your equation was off by a factor of $2\pi$ since you used angular frequency), we have a lower wavelength of $c/2Hz$ and an upper wavelength of $c/1Hz$, these are wavelengths and have units of wavelength, and in this case it is about $1.5\times10^8 m$. The same $dU$ is divided by 1Hz to get $u_\nu$ but is divided by $1.5\times10^8 m$ to get $u_\lambda$, doesn't seem like a big deal. However, what if your frequency range was from $2 Hz$ to $3 Hz$ , then there is a new $dU$ for this range. And the $\Delta \nu$ is again $3Hz-2Hz=1Hz$, but the $\Delta \lambda$ equals $c/2Hz-c/3Hz$ which is about $5\times10^7 m$. So the exact same bit of energy $dU$ is divided by a smaller range of wavelengths, so for the exact same interval of physical waves $u_\lambda$ is bigger than $u_\nu$ (bigger than it was for a slightly different interval that in frequency space was just as long). The energy involved $dU$ is exactly the same always, and the cut off wavelengths obey $\lambda \nu = c$, but the $\Delta \lambda$ and the $\Delta \nu$ are different from interval to interval. So the densities are different, since the density is the ratio of $dU$ the real energy to $\Delta \lambda$ (or $\Delta \nu$) for energy per wavelength (or energy per frequency respectively).
Now this function has a maximum somewhere, so there is a frequency where a maximal amount of energy is emitted.
The function is not energy at a frequency, it is the energy $dU$ divided by the length $\Delta \nu$ of the frequency interval in question. It isn't the energy. It is a maximum when $dU$ gets larger than any other $dU$ for intervals with the same sized $\Delta \nu$. Imagine taking a very very small interval $\Delta \nu$, and moving it to different places (but keeping the same size) until you find a place where $dU$ for that interval is largest. That's what it means for a density to be at its maximum. That's the frequency where a window of frequencies of fixed frequency spread gives the most energy. And these windows of fixed frequency spread (say 1Hz, or one mHz or one $\mu$Hz) don't have a fixed wavelength spread as you move them to different places, so it has nothing to do with where density per wavelength is maximum.
To find where $u_\lambda$ is maximal, note that is the energy $dU$ divided by the length $\Delta \lambda$ of the frequency interval in question. It isn't the energy. It is a maximum when $dU$ gets larger than any other $dU$ for intervals with the same sized $\Delta \lambda$. Imagine taking a very very small interval $\Delta \lambda$, and moving it to different places (but keeping the same size) until you find a place where $dU$ for that interval is largest. That's what it means for a density to be at its maximum. That's the wavelength where a window of wavelengths of fixed wavelength spread gives the most energy. And these windows of fixed wavelength spread (say 1mm, or one m or one km) don't have a fixed frequency spread as you move them to different places, so it has nothing to do with where density per frequency is maximum.
In other words: If you add the sum of the photon's energies at each frequency that are emitted, you will notice that the maximum is reached at this [frequency].
No, and this time it is wrong on many additional levels, this brings up photons, and energy density depends on total energy in the interval, it is not a per photon measure, that would be a totally different issue. And we aren't adding up energy per frequency, we are adding up the energy from intervals of frequencies, the result for each interval is $dU=u_\nu\Delta\nu$.
Now $E(\lambda)$ tells you basically the same for the wavelength
Except if we use the same intervals, they now have the same $dU$ for each interval, but the $\Delta \lambda$ for each interval is different, so $u_\lambda$ is different.
We know where at which frequency the maximal amount of energy is radiated, so we know the corresponding wavelength.
We might know which interval $dU$ gave the most energy, and if all the intervals were of fixed size $\Delta \nu$ in frequency space then indeed we can take $u_\nu=dU/\Delta\nu$ and the largest one will be where $u_\nu$ is largest. But to get $u_\lambda$, we have to compute $u_\lambda=dU/\Delta\lambda$ and the $\Delta \lambda$ are different for these intervals, so the one that made $u_\nu=dU/\Delta\nu$ largest is not the one that makes $u_\lambda=dU/\Delta\lambda$ largest.
It really comes down to knowing what an energy density is, an energy divided by an amount of stuff (spread of wavelengths, spread of frequency, region of volume, etc.)
The bounty was for well documented answers, so I tried to include all the details so that this answer by itself answers everything. The only thing you really need to know is what a density is, which is something you put into an integal, energy per wavelength $u_\lambda$ is something that gives
$$U=\int u_\lambda d\lambda,$$
for the total energy for any range of wavelengths. And energy per frequency $u_\nu$ is something that gives
$$U=\int u_\nu d\nu,$$
for the total energy for any range of frequencies. Absolutely everything else follows from knowing that, which is just the definition of a density.

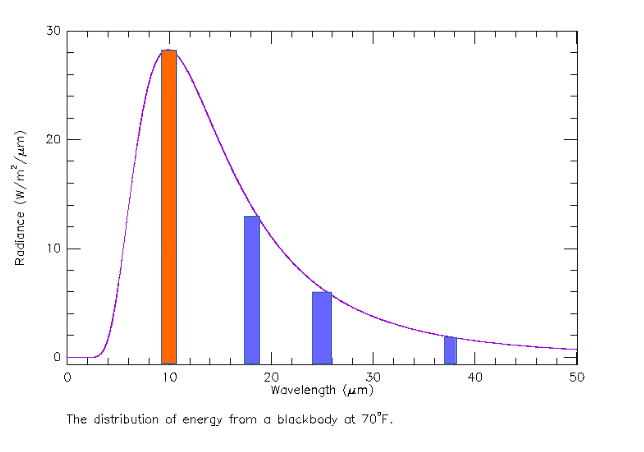 Planck curve.
Planck curve.