3
$\begingroup$

I've come to this sentence and I don't understand the term Nyquist edge.

Because observing in the FM band is not feasible, a sampling frequency of 200 MHz has been chosen for most of the receiver modes. This sampling results in a Nyquist edge almost at the center of the FM band.

Improve this question
$\endgroup$
2

1 Answer 1

Reset to default
5
$\begingroup$

In signal processing, the Nyquist–Shannon sampling theorem says you need at least 2 samples of a frequency to be able to perfectly reconstruct it. So in your question, a sampling rate of $200\: \mathrm{MHz}$ means you can perfectly reconstruct frequencies in the range of $0 - 100\: \mathrm{MHz}$. So what happens when frequencies above $100\: \mathrm{MHz}$ are present? They fold over (are aliased) into the the $0 - 100\: \mathrm{MHz}$ range and the fold-over point at $100\: \mathrm{MHz}$ is the Nyquist Edge.

For example: 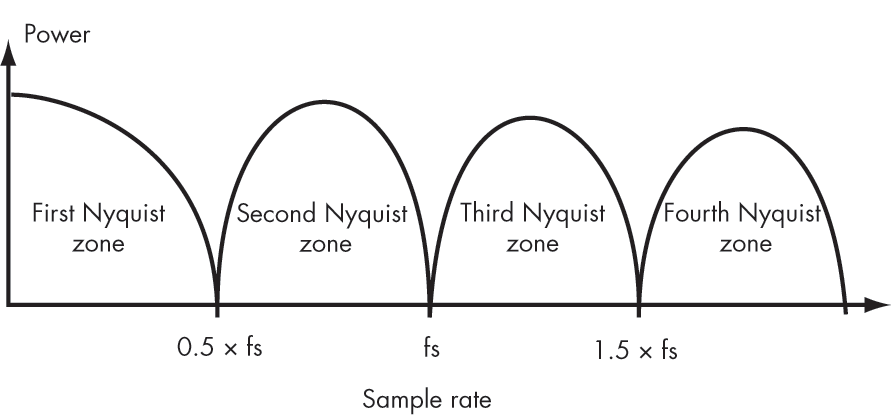nyqust zones about edges There is a pretty good article about this behavior at here.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Also, for the curious, there is an absolutely fantastic video about frequencies, sampling rates, reconstructing signals from samples, etc. over at Xiph.org here: http://www.xiph.org/video/vid2.shtml $\endgroup$
    – Brandon Enright
    Commented Dec 16, 2013 at 21:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.