I'm convinced that water waves for example:
are a combination of longitudinal and transverse. Any references or proofs of this or otherwise?
$\begingroup$
$\endgroup$
9
-
$\begingroup$ Fluids can't sustain transverse oscillations, as far as I know, but I can't think of a reference off the top of my head. $\endgroup$– David ZCommented Mar 8, 2011 at 1:13
-
1$\begingroup$ The waves in your picture are exhibiting highly nonlinear behavior. It'll be a lot easier to answer the question for linear waves, depending on what you want. $\endgroup$– Mark EichenlaubCommented Mar 8, 2011 at 4:56
-
4$\begingroup$ This question (deliberately?) mixes ambiguous terminology with nontrivial physics. The ocean surface is just 2-dimensional so both transverse and longitudinal waves on the surface have 1 polarization only, and they can't really be distinguished mathematically. It's just about the formalism. What you would have to ask is the direction of the possible gradient of pressure inside the water. It is mostly vertical (outside the plane where the wave propagates, so it's mostly longitudinal, despite the orthogonality) and then in the direction of motion (longitudinal) but none in the 3rd L/R direction. $\endgroup$– Luboš MotlCommented Mar 8, 2011 at 5:34
-
$\begingroup$ @Lubos I don't understand your comment. The surface is two-dimensional, but it exists in three-dimensional space. The question is, if you have a leaf floating on the surface of the water, how does the leaf move as a wave passes? Back-and-forth in the direction of propagation of the wave or up-and-down perpendicular to the wave's propagation? $\endgroup$– Mark EichenlaubCommented Mar 8, 2011 at 5:56
-
2$\begingroup$ Dear Mark, I wrote the very same thing. What Carl really wants to ask is how a leaf moves in the 3D space. But that's not the same thing as the question whether the waves are transverse or longitudinal. Technically, both front-rear motion and the vertical ones should be counted as longitudinal waves. The waves are waves in 2 dimensions of the surface only, so the only truly transverse direction is the horizontal left-right direction perpendicular to the direction of the wave, and non-turbulent waves don't have any such component. So both modes you mentioned are longitudinal (or scalar) waves. $\endgroup$– Luboš MotlCommented Mar 8, 2011 at 8:24
|
Show 4 more comments
6 Answers
$\begingroup$
$\endgroup$
4
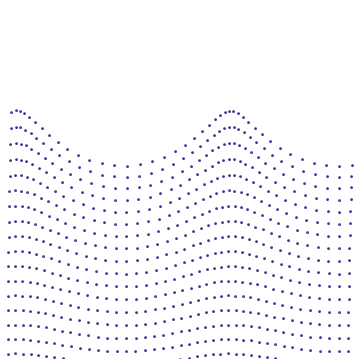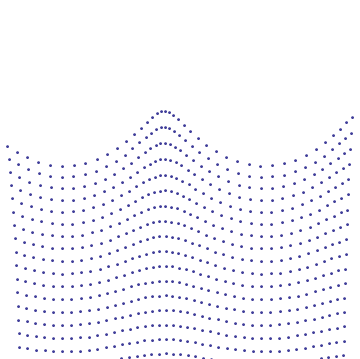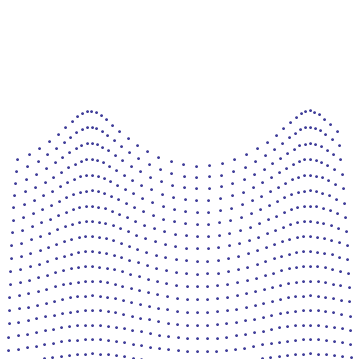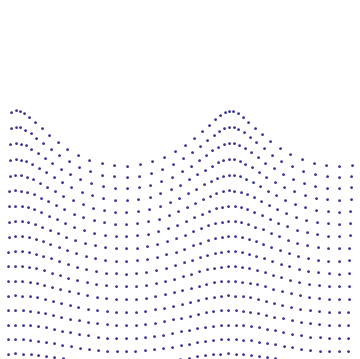
Each point is moving according to:
$x(t) = x_0 + a e^{-y_0/l} \cos(k x_0+\omega t)$
$y(t) = y_0 + a e^{-y_0/l} \sin(k x_0+\omega t)$
With $x_0,y_0$ -- "motion centre" for each particle, $a$ -- the amplitude, $l$ -- decay length with depth.
So you have exact "circular" superposition of longitudinal and transverse waves.
-
3$\begingroup$ In your animation the medium is rather compressible. $\endgroup$ Commented Mar 8, 2011 at 16:50
-
1$\begingroup$ We could consider the dots as "leaves" places throughout the medium. In that case I'd say its fairly accurate. $\endgroup$– Jus12Commented Mar 23, 2016 at 4:56
-
$\begingroup$ Although the dots appear closer at different times, this could be accounted for by displaced material, the surface of the water has more height when the dots are closer. I have a follow up question, can someone prove that this model does or does not exhibit compression? $\endgroup$– AlexCommented Mar 21, 2022 at 17:42
-
$\begingroup$ The velocity of a particle is $<-ae^{-y_0/l}\omega cos(kx_0+\omega t),ae^{-y_0/l}\omega sin(kx_0+\omega t)>$. At time $t=-kx_0/\omega$ the particle at position $<x,y>$ has $x_0=x-ae^{-y_0/l}$ and $y_0=y$. Therefore at time $t=-kx_0/\omega$ the particle at position $<x,y>$ has velocity $<-ae^{-y/l}\omega,0>$, which has zero divergence at this location and time. This model doesn't seem to have any preferred times or locations in regard to the behavior, so I believe the zero divergence applies everywhere meaning the model is not exhibiting compression. $\endgroup$– AlexCommented Mar 21, 2022 at 18:51
$\begingroup$
$\endgroup$
0
In deep waters, the fluid particles describe circles when a wave passes by. So, in a sense, these waves are neither transverse nor longitidinal. For a demonstration, see for example Howard Georgi's book (chapter 11).
In very shallow waters the particles go essentially back and forth. In the intermediate cases they follow eliptical trajectories.
$\begingroup$
$\endgroup$
1
The ocean waves are usually called "surface" waves. Whatever a particle trajectory is, the deeper in water, the smaller its amplitude. Several lengths below surface the water is still.
However deep inside there may be volume waves - from submarines, for example. They are detectable.
answered Mar 8, 2011 at 10:22
-
$\begingroup$ Interesting comment on volume waves. Perhaps we should have some sort of question on that. $\endgroup$ Commented Mar 8, 2011 at 23:17
$\begingroup$
$\endgroup$
I think the wave nature of water is both transverse and longitudinal. When we drop a stone in water that we can see there the transverse waves but then we can heard sound also, so, water waves are transverse as well as longitudinal waves!
$\begingroup$
$\endgroup$
Maybe sea waves are longitudinal at sea but when they hit the shallows of the shore they become transverse waves and take the shape of a wheel and roll towards the shore.
Just guessing this from my years of surfing. The waves are up & down out in the deep but turn into tubes when they reach the shallows.
$\begingroup$
$\endgroup$
3
I will just say what I think I know:
In the open ocean or great lakes the waves are transverse: the water goes up and down. They are originated by the winds in the surface.
Near the shore the waves become also longitudinal: the small distance from the the surface to the bottom of ocean make the difference.
added:
I described the net transport of energy along the direction of propagation of the waves.
In deep waters it is the vertical motion of the surface that is able to do work (on devices that extend more than a wavelength) against the gravitational field.
-
$\begingroup$ The Physics of Ocean Waves, by Michael Twardos at UCI $\endgroup$ Commented Mar 8, 2011 at 4:03
-
6$\begingroup$ The water cannot just go up and down in the deep water because it's essentially incompressible. If the water level goes up somewhere, it has to come in from the sides. $\endgroup$ Commented Mar 8, 2011 at 4:54
-
$\begingroup$ @Mark : I agree with your comment and Carlos answer. I was describing in terms of net transport of energy along the direction of the wave, and in this sence I believe that my answer is still OK. $\endgroup$ Commented Mar 9, 2011 at 14:31